Article contents
On Stieltjes-Volterra integral equations
Published online by Cambridge University Press: 17 April 2009
Abstract
A Stieltjes-Volterra integral equation system
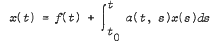
is firstly considered. Pointwise estimates and boundedness of its solutions are obtained under various conditions on the function K. To do this, the well-known Gronwall-Bellman integral inequality is generalized. For a particular choice of u, it is shown that the integral equation reduces to a difference equation. The problem of existence (and non-existence), uniqueness (and non-uniqueness) of the difference equation is discussed. Gronwall-Bellman inequality is further generalized to n linear terms and is subsequently applied to obtain sufficient conditions in order that a certain stability of the unperturbed Volterra system
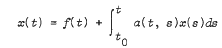
implies the corresponding local stability of the (discontinuously) perturbed system
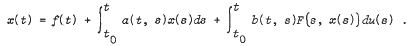
- Type
- Research Article
- Information
- Copyright
- Copyright © Australian Mathematical Society 1978
References
- 2
- Cited by


