Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-26T22:44:46.640Z Has data issue: false hasContentIssue false
  • English
  • Français

ON PROPERTIES OF FINITE-ORDER MEROMORPHIC SOLUTIONS OF A CERTAIN DIFFERENCE PAINLEVÉ I EQUATION

Part of: Entire and meromorphic functions, and related topics

Published online by Cambridge University Press:  08 December 2011

MEI-RU CHEN  and
ZONG-XUAN CHEN
MEI-RU CHEN*
Affiliation:
School of Mathematical Sciences, South China Normal University, Guangzhou, 510631, PR China (email: [email protected])
ZONG-XUAN CHEN
Affiliation:
School of Mathematical Sciences, South China Normal University, Guangzhou, 510631, PR China (email: [email protected])
*
✉For correspondence; e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper, we investigate properties of finite-order transcendental meromorphic solutions, rational solutions and polynomial solutions of the difference Painlevé I equation 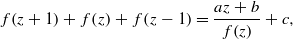where a, b and c are constants, ∣a∣+∣b∣≠0.

Keywords

difference Painlevé equationtranscendental solutionrational solutionpolynomial solution

MSC classification

Secondary: 30D35: Distribution of values, Nevanlinna theory
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 85 , Issue 3 , June 2012 , pp. 463 - 475
Copyright
Copyright © Australian Mathematical Publishing Association Inc. 2011

Footnotes

This project was supported by the National Natural Science Foundation of China (Nos 10871076 and 11171119).

References

[1]Ablowitz, M. J. and Clarkson, P. A., Solutions, Nonlinear Evolution Equations and Inverse Scattering, London Mathematical Society Lecture Note Series, 149 (Cambridge University Press, Cambridge, 1997), pp. 347–420.Google Scholar
[2]Ablowitz, M., Halburd, R. G. and Herbst, B., ‘On the extension of Painlevé property to difference equations’, Nonlinearity 13 (2000), 889–905.CrossRefGoogle Scholar
[3]Ablowitz, M. J. and Segur, H., Solitons and the Inverse Scattering Transform (SIAM, Philadelphia, 1981), pp. 233–250.CrossRefGoogle Scholar
[4]Bergweiler, W. and Langley, J. K., ‘Zeros of differences of meromorphic functions’, Math. Proc. Cambridge Philos. Soc. 142 (2007), 133–147.CrossRefGoogle Scholar
[5]Chen, Z. X., ‘On properties of meromorphic solutions for some difference equations’, Kodai Math. I. 34 (2011), 244–256.Google Scholar
[6]Chen, Z. X. and Shon, K. H., ‘On the zeros and fixed points of differences of meromorphic functions’, J. Math. Anal. Appl. 344 (2008), 373–383.CrossRefGoogle Scholar
[7]Chen, Z. X. and Shon, K. H., ‘Value distribution of meromorphic solutions of certain difference Painlevé equations’, J. Math. Anal. Appl. 364 (2010), 556–566.CrossRefGoogle Scholar
[8]Chiang, Y. M. and Feng, S. J., ‘On the Nevanlinna characteristic of f(z+η) and difference equations in the complex plane’, Ramanujan J. 16 (2008), 105–129.CrossRefGoogle Scholar
[9]Fokas, A. S., ‘From continuous to discrete Painlevé equations’, J. Math. Anal. Appl. 180 (1993), 342–360.CrossRefGoogle Scholar
[10]Halburd, R. G. and Korhonen, R., ‘Difference analogue of the lemma on the logarithmic derivative with applications to difference equations’, J. Math. Anal. Appl. 314 (2006), 477–487.CrossRefGoogle Scholar
[11]Halburd, R. G. and Korhonen, R., ‘Existence of finite-order meromorphic solutions as a detector of integrability in difference equations’, Phys. D 218 (2006), 191–203.CrossRefGoogle Scholar
[12]Halburd, R. G. and Korhonen, R., ‘Meromorphic solution of difference equation, integrability and the discrete Painlevé equations’, J. Phys. A 40 (2007), 1–38.CrossRefGoogle Scholar
[13]Halburd, R. G. and Korhonen, R., ‘Finite-order meromorphic solutions and the discrete Painlevé equations’, Proc. Lond. Math. Soc. 94 (2007), 443–474.CrossRefGoogle Scholar
[14]Hayman, W. K., Meromorphic Functions (Clarendon Press, Oxford, 1964).Google Scholar
[15]Heittokangas, J., Korhonen, R., Laine, I., Rieppo, J. and Hohge, K., ‘Complex difference equations of Malmquist type’, Comput. Methods Funct. Theory 1 (2001), 27–39.CrossRefGoogle Scholar
[16]Heittokangas, J., Korhonen, R., Laine, I., Rieppo, J. and Zhang, J., ‘Value sharing results for shifts of meromorphic functions, and sufficient conditions for periodicity’, J. Math. Anal. Appl. 355 (2009), 352–363.CrossRefGoogle Scholar
[17]Its, A. R. and Novokshenov, Yu. V., The Isomonodromic Deformation Method in the Theory of Painlevé Equations, Lecture Notes in Mathematics, 1191 (Springer, Berlin, 1986).CrossRefGoogle Scholar
[18]Kudryashov, N. A., ‘The second Painlevé equation as a model for the electric field in a semiconductor’, Phys. Lett. A 233 (1997), 397–400.CrossRefGoogle Scholar
[19]Laine, I., Nevanlinna Theory and Complex Differential Equations (Walter de Gruyter, Berlin, 1993).CrossRefGoogle Scholar
[20]Laine, I. and Yang, C. C., ‘Clunie theorems for difference and q-difference polynomials’, J. Lond. Math. Soc. 76(3) (2007), 556–566.CrossRefGoogle Scholar
[21]Painlevé, P., ‘Mémoire sur les équations differentielles dont l’intégrale générale est uniforme’, Bull. Soc. Math. France 28 (1900), 201–261.CrossRefGoogle Scholar
[22]Shimomura, S., ‘Entire solutions of a polynomial difference equation’, J. Fac. Sci. Univ. Tokyo Sect. IA Math. 28 (1981), 253–266.Google Scholar
[23]Yanagihara, N., ‘Meromorphic solutions of some difference equations’, Funkcial. Ekvac. 23 (1980), 309–326.Google Scholar
[24]Yang, L., Value Distribution and New Research (Science Press, Beijing, 1982) (in Chinese).Google Scholar