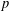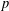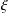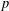Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Bugeaud, Yann
and
Kekeç, Gülcan
2020.
On Mahler’s p-adic S-, T -, and U-numbers.
Analele Universitatii "Ovidius" Constanta - Seria Matematica,
Vol. 28,
Issue. 1,
p.
81.
Charlier, É.
Massuir, A.
Rigo, M.
and
Rowland, E.
2022.
Ultimate periodicity problem for linear numeration systems.
International Journal of Algebra and Computation,
Vol. 32,
Issue. 03,
p.
561.
Kekeç, Gülcan
2023.
On Transcendental Regular Continued Fractions.
Mathematica Slovaca,
Vol. 73,
Issue. 6,
p.
1399.
KEKEÇ, GÜLCAN
2024.
TRANSCENDENTAL RUBAN p-ADIC CONTINUED FRACTIONS.
Bulletin of the Australian Mathematical Society,
p.
1.