Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-26T14:58:49.839Z Has data issue: false hasContentIssue false
  • English
  • Français

ON FINDING SOLUTIONS TO EXPONENTIAL CONGRUENCES

Part of: Finite fields and commutative rings (number-theoretic aspects) Theory of computing Computational number theory Diophantine equations

Published online by Cambridge University Press:  27 December 2018

IGOR E. SHPARLINSKI Open the ORCID record for IGOR E. SHPARLINSKI [Opens in a new window]
IGOR E. SHPARLINSKI*
Affiliation:
Department of Pure Mathematics, University of New South Wales, Sydney, NSW 2052, Australia email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We improve some previously known deterministic algorithms for finding integer solutions 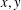$x,y$ to the exponential equation of the form 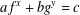$af^{x}+bg^{y}=c$ over finite fields.

Keywords

exponential equationalgorithmfinite field

MSC classification

Primary: 11D61: Exponential equations
Secondary: 11T71: Algebraic coding theory; cryptography 11Y16: Algorithms; complexity 68Q25: Analysis of algorithms and problem complexity
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 99 , Issue 3 , June 2019 , pp. 388 - 391
Copyright
© 2018 Australian Mathematical Publishing Association Inc. 

Footnotes

During the preparation of this work, the author was supported in part by the Australian Research Council Grant DP170100786.

References

Berndt, B., Evans, R. and Williams, K. S., Gauss and Jacobi Sums, Canadian Mathematical Society Series of Monographs and Advanced Texts, 21 (John Wiley & Sons, New York, 1998).Google Scholar
Crandall, R. and Pomerance, C., Prime Numbers: A Computational Perspective (Springer, Berlin, 2005).Google Scholar
Lenstra, A. K. and de Weger, B., On the Possibility of Constructing Meaningful Hash Collisions for Public Keys, Lecture Notes in Computer Science, 3574 (Springer, Berlin, 2005), 267–279.Google Scholar
Sasaki, Y., ‘On zeros of exponential polynomials and quantum algorithms’, Quantum Inf. Processing 9 (2010), 419–427.Google Scholar
Shanks, D., Class Number, A Theory of Factorization and Genera, Proceedings of Symposia in Pure Mathematics, 20 (American Mathematical Society, Providence, RI, 1971), 415–440.Google Scholar
Storer, T., Cyclotomy and Difference Sets, Lectures in Advanced Mathematics, 2 (Markham Publishing Company, Chicago, IL, 1967).Google Scholar
van Dam, W. and Shparlinski, I. E., Classical and Quantum Algorithms for Exponential Congruences, Lecture Notes in Computer Science, 5106 (Springer, Berlin, 2008), 1–10.Google Scholar