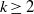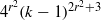Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
GOYAL, MEGHA
2019.
AN ANALOGUE OF EULER’S IDENTITY AND SPLIT PERFECT PARTITIONS.
Bulletin of the Australian Mathematical Society,
Vol. 99,
Issue. 03,
p.
353.
Vavilov, N. A.
2023.
Computers As a Novel Mathematical Reality: III. Mersenne Numbers and Sums of Divisors.
Doklady Mathematics,
Vol. 107,
Issue. 3,
p.
173.