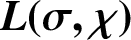Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-30T21:42:28.692Z Has data issue: false hasContentIssue false
  • English
  • Français

A NOTE ON LARGE VALUES OF $\boldsymbol{L(\sigma ,\chi )}$

Part of: Zeta and $L$-functions: analytic theory

Published online by Cambridge University Press:  04 October 2021

XUANXUAN XIAO Open the ORCID record for XUANXUAN XIAO [Opens in a new window]  and
QIYU YANG Open the ORCID record for QIYU YANG [Opens in a new window]
XUANXUAN XIAO
Affiliation:
Faculty of Information Technology, Macau University of Science and Technology, Macau e-mail: [email protected]
QIYU YANG*
Affiliation:
Faculty of Information Technology, Macau University of Science and Technology, Macau
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In this note, by introducing a new variant of the resonator function, we give an explicit version of the lower bound for $\log |L(\sigma ,\chi )|$ in the strip 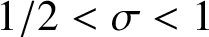$1/2<\sigma <1$ , which improves the result of Aistleitner et al. [‘On large values of 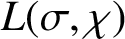$L(\sigma ,\chi )$ ’, Q. J. Math. 70 (2019), 831–848].

Keywords

large valueDirichlet L-functionresonance method

MSC classification

Primary: 11M06: $zeta (s)$ and $L(s, chi)$
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 105 , Issue 3 , June 2022 , pp. 412 - 418
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

This work is supported by the Science and Technology Development Fund, Macau SAR (File no. 0095/2018/A3).

References

Aistleitner, C., ‘Lower bounds for the maximum of the Riemann zeta function along vertical lines’, Math. Ann. 365 (2016), 473–496.CrossRefGoogle Scholar
Aistleitner, C., Mahatab, K., Munsch, M. and Peyrot, A., ‘ On large values of $L\left(\sigma, \chi \right)$ ’, Q. J. Math. 70 (2019), 831–848.CrossRefGoogle Scholar
Bondarenko, A. and Seip, K., ‘Note on the resonance method for the Riemann zeta function’, in: 50 Years with Hardy Spaces, Operator Theory: Advances and Applications, 261 (eds. Baranov, A., Kisliakov, S. and Nikolski, N.) (Birkhaüser, Cham, 2018), 121–140.CrossRefGoogle Scholar
Davenport, H., Multiplicative Number Theory, 2nd edn, Graduate Texts in Mathematics, 74 (revised by Montgomery, H. L.) (Springer, New York–Berlin, 1980).CrossRefGoogle Scholar
Hilberdink, T., ‘An arithmetical mapping and applications to $\Omega$ -results for the Riemann zeta function’, Acta Arith. 139 (2009), 341–367.CrossRefGoogle Scholar
Montgomery, H. L., ‘Extreme values of the Riemann zeta function, Comment. Math. Helv. 52 (1977), 511–518.CrossRefGoogle Scholar
Soundararajan, K., ‘Extreme values of zeta and L-functions’, Math. Ann. 342 (2008), 467–486.CrossRefGoogle Scholar