Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-26T16:36:28.618Z Has data issue: false hasContentIssue false
  • English
  • Français

NORMAL BASES FOR MODULAR FUNCTION FIELDS

Part of: Discontinuous groups and automorphic forms Arithmetic algebraic geometry

Published online by Cambridge University Press:  02 March 2017

JA KYUNG KOO ,
DONG HWA SHIN  and
DONG SUNG YOON
JA KYUNG KOO
Affiliation:
Department of Mathematical Sciences, KAIST, Daejeon 34141, Republic of Korea email [email protected]
DONG HWA SHIN
Affiliation:
Department of Mathematics, Hankuk University of Foreign Studies, Yongin-si, Gyeonggi-do 17035, Republic of Korea email [email protected]
DONG SUNG YOON*
Affiliation:
Department of Mathematical Sciences, KAIST, Daejeon 34141, Republic of Korea email [email protected]
*
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We provide a concrete example of a normal basis for a finite Galois extension which is not abelian. More precisely, let 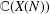$\mathbb{C}(X(N))$ be the field of meromorphic functions on the modular curve 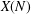$X(N)$ of level $N$. We construct a completely free element in the extension 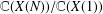$\mathbb{C}(X(N))/\mathbb{C}(X(1))$ by means of Siegel functions.

Keywords

modular functionsmodular unitsnormal bases

MSC classification

Primary: 11F03: Modular and automorphic functions
Secondary: 11G16: Elliptic and modular units
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 95 , Issue 3 , June 2017 , pp. 384 - 392
Copyright
© 2017 Australian Mathematical Publishing Association Inc. 

Footnotes

The second author was supported by Hankuk University of Foreign Studies Research Fund of 2016.

References

Blessenohl, D. and Johnsen, K., ‘Eine Verschärfung des Satzes von der Normalbasis’, J. Algebra 103(1) (1986), 141–159.CrossRefGoogle Scholar
Hachenberger, D., ‘Universal normal bases for the abelian closure of the field of rational numbers’, Acta Arith. 93(4) (2000), 329–341.CrossRefGoogle Scholar
Jung, H. Y., Koo, J. K. and Shin, D. H., ‘Normal bases of ray class fields over imaginary quadratic fields’, Math. Z. 271(1–2) (2012), 109–116.Google Scholar
Koo, J. K. and Shin, D. H., ‘Completely normal elements in some finite abelian extensions’, Cent. Eur. J. Math. 11(10) (2013), 1725–1731.Google Scholar
Kubert, D. and Lang, S., Modular Units, Grundlehren der Mathematischen Wissenschaften, 244 (Springer, New York, 1981).Google Scholar
Lang, S., Elliptic Functions, 2nd edn, Graduate Texts in Mathematics, 112 (Springer, New York, 1987).CrossRefGoogle Scholar
Leopoldt, H.-W., ‘Über die Hauptordnung der ganzen Elemente eines abelschen Zahlkörpers’, J. reine angew. Math. 201 (1959), 119–149.CrossRefGoogle Scholar
Okada, T., ‘On an extension of a theorem of S. Chowla’, Acta Arith. 38(4) (1980/81), 341–345.Google Scholar
Schertz, R., ‘Galoismodulstruktur und elliptische Funktionen’, J. Number Theory 39(3) (1991), 285–326.CrossRefGoogle Scholar
Shimura, G., Introduction to the Arithmetic Theory of Automorphic Functions (Iwanami Shoten and Princeton University Press, Princeton, NJ, 1971).Google Scholar
Taylor, M. J., ‘Relative Galois module structure of rings of integers and elliptic functions II’, Ann. of Math. (2) 121(3) (1985), 519–535.CrossRefGoogle Scholar
van der Waerden, B. L., Algebra I (Springer, New York, 1991).Google Scholar