Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-08T05:32:08.509Z Has data issue: false hasContentIssue false
  • English
  • Français

Multipliers between some function spaces on groups

Published online by Cambridge University Press:  17 April 2009

A.K. Gupta  and
U.B. Tewari
A.K. Gupta
Affiliation:
Department of Mathematics, Indian Institute of Technology Kanpur, Kanpur, India.
U.B. Tewari
Affiliation:
Department of Mathematics, Indian Institute of Technology Kanpur, Kanpur, India.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let G be a nondiscrete locally compact abelian group with dual group Γ. For 1 ≤ p ≤ ∞, denote by Ap(G) the space of integrable functions on G whose Fourier transforms belong to Lp(Γ). We investigate multipliers from Ap(G) to Aq(G). If G is compact and 2 < p1, p2 < ∞, we show that multipliers of 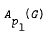and multipliers of 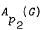are different, provided Pl ≠ P2. For compact G, we also exhibit a relationship between lr (Γ) and the multipliers from Ap(G) to Aq(G). If G is a compact nonabelian group we observe that the spaces Ap(G) behave in the same way as in the abelian case as far as the multiplier problems are concerned.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 18 , Issue 1 , February 1978 , pp. 1 - 11
Copyright
Copyright © Australian Mathematical Society 1978

References

[1]Edwards, R.E., “Changing signs of Fourier coefficients”, Pacific J. Math. 15 (1965), 463–475.CrossRefGoogle Scholar
[2]Figà–Talamanca, Alessandro and Gaudry, Garth I., “Multipliers and sets of uniqueness of Lp”, Michigan Math. J. 17 (1970), 179–191.CrossRefGoogle Scholar
[3]Fournier, John J.F., “Local complements to the Hausdorff–Young theorem”, Michigan Math. J. 20 (1973), 263–276.CrossRefGoogle Scholar
[4]Hewitt, Edwin and Ross, Kenneth A., Abstract harmonic analysis, Volume II (Die Grundlehren der mathematischen Wissenschaften, 152. Springer–Verlag, Berlin, Heidelberg, New York, 1970).Google Scholar
[5]Kelley, J.L., Namioka, Isaac and Donoghue, W.F. Jr, Lucas, Kenneth R., Pettis, B.J., Poulsen, Ebbe Thue, Price, G. Baley, Robertson, Wendy, Scott, W.R., Smith, Kennan T., Linear topological spaces (Van Nostrand, Princeton, New Jersey; Toronto; New York; London; 1963. Second printing: Graduate Texts in Mathematics, 36. Springer–Verlag, New York, Heidelberg, Berlin, 1976).CrossRefGoogle Scholar
[6]Krogstad, Harald E., A note on Ap–algebras (Institute Mittag-Leffler, Report no. 5, 1974).Google Scholar
[7]Larsen, Ronald, An introduction to the theory of multipliers (Die Grundlehren der mathematischen Wissenschaften, 175. Springer-Verlag, Berlin, Heidelberg, New York, 1971).CrossRefGoogle Scholar
[8]Larsen, R., “The algebras of functions with Fourier transforms in Lp: a survey”, Nieuw Arch. Wisk. (3) 22 (1974), 195–240.Google Scholar
[9]Price, J.F., “Some strict inclusions between spaces of Lp – multipliers”, Trans. Amer. Math. Soc. 152 (1970), 321–330.Google Scholar
[10]Tewari, U.B. and Gupta, A.K., “The algebra of functions with Fourier transforms in a given function space”, Bull. Austral. Math. Soc. 9 (1973), 73–82.CrossRefGoogle Scholar