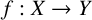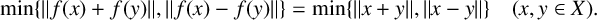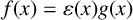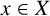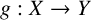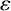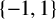MIN-PHASE-ISOMETRIES IN STRICTLY CONVEX NORMED SPACES

Abstract

Suppose that X and Y are two real normed spaces. A map $f:X\rightarrow Y$ is called a min-phase-isometry if it satisfies

$$ \begin{align*} \min\{\|f(x)+f(y)\|,\|f(x)-f(y)\|\}=\min\{\|x+y\|,\|x-y\|\} \quad (x,y\in X). \end{align*} $$

We present properties of min-phase-isometries in the case that Y is strictly convex and show that if a min-phase-isometry f (not necessarily surjective) fixes the origin, then it is phase-equivalent to a linear isometry, that is, $f(x)=\varepsilon (x)g(x)$ for $x\in X$, where $g:X\rightarrow Y$ is a linear isometry and $\varepsilon $ is a map from X to $\{-1,1\}$.

1 Introduction

For normed spaces X and Y, a map $f:X\rightarrow Y$ is called an isometry if

$$ \begin{align*} \|f(x)-f(y)\|=\|x-y\| \quad (x,y\in X), \end{align*} $$

f is called a phase-isometry if

(1.1) $$ \begin{align} \{\|f(x)-f(y)\|,\|f(x)+f(y)\|\}=\{\|x-y\|,\|x+y\|\} \quad (x,y\in X), \end{align} $$

and $f$ is called a min-phase isometry if

$$ \begin{align*} \min\{\|f(x)+f)y)\|, \|f(x)-f(y)\|\} = \min\{\|x+y\|,\|x-y\|\} \quad (x,y\in X). \end{align*} $$

Two maps $f,g:X\rightarrow Y$ are phase-equivalent if there is a phase function $\varepsilon :X\rightarrow \mathbb {T}$ such that $f=\varepsilon \cdot g$ , where $\mathbb {T}=\{-1, 1\}$ in the real case and $\mathbb {T}=\{\lambda \in \mathbb {C} : |\lambda |=1\}$ in the complex case.

Wigner’s theorem on symmetry transformations plays a fundamental role in quantum mechanics. There are several proofs for this result (see [5, 6, 16, 17]) and more details can be found in the survey [2]. This theorem has many equivalent forms. One of these reads as follows. Assume that H and K are real or complex inner product spaces. A map $f : H\rightarrow K$ satisfies

(1.2) $$ \begin{align} |\langle f(x), f(y) \rangle|=|\langle x, y \rangle| \quad (x,y\in H) \end{align} $$

if and only if it is phase-equivalent to a linear or a conjugate-linear isometry from H to K.

It is easily checked that for a map f between two real inner product spaces H and K, (1.1) and (1.2) are equivalent. In other words, every phase-isometry from one real inner product space to another is phase-equivalent to a linear isometry. Hence, a natural question arises (see [14, Problem 1]): under what conditions, when X and Y are real normed rather than inner product spaces, is every phase-isometry from X to Y phase-equivalent to a linear isometry. For surjective phase-isometries, Ilišević, Omladič and Turnšek in [12, Theorem 4.2] cleverly gave a positive answer to this problem using the proof of the Mazur–Ulam theorem. Removing the assumption of surjectivity, supposing instead that Y is strictly convex, they also answered it affirmatively in [11, Theorem 2.4]. In [10], Huang and the first author considered the problem under a weaker condition than (1.1) and obtained the same results. For further results, see [7–9, 18].

In this note, we continue to study min-phase-isometries in the case that the surjectivity assumption is replaced by the strict convexity of the target space Y. It should be remarked that it is easy to show that every phase-isometry f (not necessarily surjective) or surjective min-phase-isometry fixes the origin, that is, ${f(0)=0}$ . However, this is no longer true for min-phase-isometries that are not surjective (see Example 2.3). Thus a min-phase-isometry, in general, may not be phase-equivalent to any linear isometry.

Similar to the approach in [11], we apply the strict convexity of Y to show that the midpoints of the segments $[x, y]$ map to one of the midpoints of the segments $[\pm f(x), \pm f(y)]$ . However, the proof is different, because we only have one side of the equation. Moreover, these results in the weaker case provide a tool for wider applicability, which can be seen in the remarks and a related inference below.

For a normed space X, the quotient space $\widetilde {X}:=X/\{\pm 1\}$ is obtained by identifying a pair of antipodal points. A natural choice of metric on this space is induced by the metric on X given by

(1.3) $$ \begin{align} d(\tilde{x}, \tilde{y})=\min\{\|x+y\|, \|x-y\|\} \quad (\tilde{x},\tilde{y}\in \widetilde{X}). \end{align} $$

To study the stability of the phase retrieval process, $\widetilde {X}$ must be given a reasonable topological structure. The quotient metric (1.3) is often used to avoid ambiguity. It is worth noting that phase retrieval plays an important role in diffraction imaging [1], astronomy [4], radar [13] and speech recognition [15].

Our main result is the following theorem.

Theorem 1.1. Suppose that X and Y are two real normed spaces with Y being strictly convex. If $f:X\rightarrow Y$ is a min-phase-isometry with $f(0)=0$ , then f is phase-equivalent to a linear isometry.

We can get an immediate corollary from the main theorem.

Corollary 1.2. Suppose that X and Y are two real normed spaces with Y being strictly convex. If $F: \widetilde {X} \rightarrow \widetilde {Y}$ is a mapping which satisfies $F(0)=0$ and is distance-preserving, that is,

$$ \begin{align*} d(F(\widetilde{x}),F(\widetilde{y}))=d(\widetilde{x},\widetilde{y}) \quad (x,y\in X), \end{align*} $$

then there exists a surjective linear isometry $U:X \rightarrow Y$ such that $F(\widetilde {x})=\widetilde {U(x)}$ .

2 Main result

Recall that a normed space Y is said to be strictly convex if $\|x+y\|\leq \|x\|+\|y\|$ for all $x,y\in Y$ , and the equality holds if and only if x and y are positively linearly dependent. In a strictly convex normed space, the midpoint $z=\tfrac 12(x+y)$ of the segment $[x,y]$ is the only point such that $\|z-x\|=\|z-y\|=\tfrac 12\|x-y\|$ .

Throughout this paper, all spaces are assumed to be real. For all real numbers $a,b$ , we set $a\wedge b:=\min (a,b)$ . We begin with a fundamental property of min-phase-isometries.

Lemma 2.1. Suppose that $X,Y$ are normed spaces with Y being strictly convex. If $f:X\rightarrow Y$ is a min-phase-isometry, then $\|f(x)\|\geq \|x\|$ for all $x\in X$ .

Proof. For every nonzero vector $x\in X$ , since f is a min-phase-isometry, we see that

(2.1) $$ \begin{align} (\|f(2x)+f(x)\| \wedge\|f(2x)-f(x)\|) +(\|f(x)+f(0)\|)\wedge\|f(x)-f(0)\|)=2\|x\|. \end{align} $$

However,

(2.2) $$ \begin{align} \|f(2x)+f(x)\|+\|f(x)+f(0)\| & \geq \|f(2x)+f(x)+(-f(x)-f(0))\|\nonumber \\ &\geq\|f(2x)-f(0)\|\geq2\|x\| \end{align} $$

and

$$ \begin{align*} \|f(2x)+f(x)\|+\|f(x)-f(0)\|&\geq \|f(2x)+f(x)+(-f(x)+f(0))\|\\ &\geq\|f(2x)+f(0)\|\geq2\|x\|. \end{align*} $$

Similarly, we can get

$$ \begin{align*} \|f(2x)-f(x)\|+\|f(x)+f(0)\|&\geq2\|x\| \end{align*} $$

and

$$ \begin{align*} \|f(2x)-f(x)\|+\|f(x)-f(0)\|\geq2\|x\|. \end{align*} $$

These inequalities combined with (2.1) imply that at least one equality is achieved. Without loss of generality, we may assume that (2.2) is an equality. Since Y is strictly convex, we conclude that

$$ \begin{align*} 2f(x)= -f(2x)-f(0) \end{align*} $$

and hence $\|f(x)\|\geq \|x\|$ .

Remark 2.2. One may wonder if a min-phase-isometry is norm-preserving. This is true in the surjective case (see [10, Lemma 2.1(a)]). However, we present an example to show that it is not valid in the nonsurjective case even in finite-dimensional spaces.

Example 2.3. Let $f: \mathbb {R}\rightarrow \mathbb {R}$ be a map such that $f(0)=-\tfrac 13$ , $f(x)=-\tfrac 13-x$ if $x>0$ and $f(x)=f(-x)$ if $x<0$ . Then f is a min-phase-isometry but not norm-preserving.

As seen in the above example, a min-phase-isometry may not map the origin to the origin, but it satisfies a local property which is established next.

Proposition 2.4. Suppose that $X, Y$ are normed spaces with Y being strictly convex. If $f:X\rightarrow Y$ is a min-phase-isometry, then for all $x,y\in X$ with $\|x-y\|\leq \|x+y\|$ , there are $\alpha , \beta \in \{-1,1\}$ such that

$$ \begin{align*} 2f\bigg(\frac{x+y}{2}\bigg)=\alpha f(x)+\beta f(y). \end{align*} $$

Proof. Set $z=\tfrac 12(x+y)$ . Since $\|x-y\|\leq \|x+y\|$ , it follows that

(2.3) $$ \begin{align} \|x+z\|\geq2\|z\|- \|z-x\|=\|x+y\|-\|x-y\|/2 \geq \|x-y\|/2=\|x-z\|. \end{align} $$

Similarly, we get

(2.4) $$ \begin{align} \|z+y\| \geq \|x-y\|/2=\|z-y\|. \end{align} $$

Since f is a min-phase-isometry, we see that

$$ \begin{align*} \min \{\|f(x)+f(y)\|, \|f(x)-f(y)\|\}=\|x-y\|. \end{align*} $$

Obviously, we have

(2.5) $$ \begin{align} \|x-z\|+\|z-y\|=\|x-y\| \end{align} $$

and it is easily checked that

$$ \begin{align*} &\|f(x)-f(z)\|+\|f(z)-f(y)\|\geq \|f(x)-f(y)\| \\ &\|f(x)-f(z)\|+\|f(z)+f(y)\|\geq \|f(x)+f(y)\|\\ &\|f(x)+f(z)\|+\|f(z)-f(y)\|\geq \|f(x)+f(y)\|\\ &\|f(x)+f(z)\|+\|f(z)+f(y)\|\geq \|f(x)-f(y)\|. \end{align*} $$

These combined with (2.3), (2.4) and (2.5) guarantee that at least one of the previous four inequalities is an equality. We may assume that the first one is an equality, that is, $\|f(x)-f(z)\|+\|f(z)-f(y)\|=\|f(x)-f(y)\|$ . Since Y is strictly convex,

$$ \begin{align*} f(z)=\tfrac{1}{2}(f(x)+f(y)) \end{align*} $$

as desired. The proof is complete.

Example 2.3 also demonstrates that we cannot expect Proposition 2.4 to be valid for all $x,y\in X$ since it does not hold for $x=-1, y=1$ in Example 2.3, but we do get this if we consider those min-phase-isometries fixing the origin.

Lemma 2.5. Assume that $X, Y$ are normed spaces. If $f:X\rightarrow Y$ is a min-phase- isometry with $f(0)=0$ , then f is norm-preserving.

Proof. The conclusion follows immediately from

$$ \begin{align*} \|f(x)\|=\min\{\|f(x)+f(0)\|,\|f(x)-f(0)\|\} =\min\{\|x+0\|,\|x-0\|\}=\|x\|.\\[-36pt] \end{align*} $$

In what follows, it will be shown that those min-phase-isometries fixing the origin enjoy better properties, enabling us to show that they are phase-equivalent to linear isometries.

Lemma 2.6. Suppose that $X, Y$ are normed spaces with Y being strictly convex. If $f:X\rightarrow Y$ is a min-phase-isometry with $f(0)=0$ , then $f(\lambda x)\in \{-\lambda f(x), \lambda f(x)\}$ for $\lambda \in \mathbb {R}$ and $x\in X$ .

Proof. Let $y=\lambda x$ and $\lambda \geq 0$ . Replacing $\lambda $ by $1/\lambda $ , we may assume that $\lambda \in [0,1]$ . Then

$$ \begin{align*} (1-\lambda)\|x\|&=\min\{\|f(x)+f(y)\|,\|f(x)-f(y)\|\}\\ &\geq\|f(x)\|-\|f(y)\| =\|x\|-\|y\| =\|x\|-\lambda\|x\|=(1-\lambda)\|x\|, \end{align*} $$

so $\|f(x)+f(\lambda x)\|\wedge \|f(x)-f(\lambda x)\|=\|f(x)\|-\|f(\lambda x)\|$ . Strict convexity of Y yields $f(\lambda x)\in \{-\lambda f(x),\lambda f(x)\}$ . Finally, from $\min \{\|f(-z)+f(z)\|, \|f(-z)-f(z)\|\}=0$ for ${z\in X}$ , we deduce that $f(-z)\in \{-f(z),f(z)\}$ . This finishes the proof.

The following easy fact will be of use later.

Lemma 2.7. Suppose that $X, Y$ are normed spaces with Y being strictly convex, and assume that $f:X\rightarrow Y$ is a min-phase-isometry with $f(0)=0$ . If $x, y \in X$ are linearly independent, then $f(x)$ and $f(y)$ are linearly independent.

Proof. Assume, in contrast, there are $x,y\in X$ which are linearly independent such that $f(y)=\lambda f(x)$ with $\lambda \neq 0$ . Lemma 2.6 guarantees that

$$ \begin{align*} \|\lambda x+y\| \wedge\|\lambda x-y\|=\|f(\lambda x)+f(y)\| \wedge \|f(\lambda x)-f(y)\|=0. \end{align*} $$

This is a contradiction since $x,y$ are linearly independent. The proof is complete.

By the previous lemmas, we reach our main proposition which is an extension of Proposition 2.4 by removing the assumption on X.

Proposition 2.8. Suppose that $X, Y$ are normed spaces with Y being strictly convex, and assume that $f:X\rightarrow Y$ is a min-phase-isometry with $f(0)=0$ . Then for all ${x,y\in X}$ , there exist $\alpha ,\beta \in \{\pm 1\}$ such that $f(x+y)=\alpha f(x)+\beta f(y)$ .

Proof. By Proposition 2.4 and Lemma 2.6, we only need to consider those $x,y\in X$ such that $\|x-y\|>\|x+y\|$ . We may assume that $\|x\|\leq \|y\|$ and $x,y$ are linearly independent. Then it follows from Lemma 2.7 that $f(x)$ and $f(y)$ are linearly independent.

We apply Proposition 2.4 and Lemma 2.6 to $-x$ and y to obtain $\alpha _1,\beta _1\in \{-1,1\}$ such that

(2.6) $$ \begin{align} f(y-x)=\alpha_1 f(x)+\beta_1f(y). \end{align} $$

Set $u=y-x$ and $v=y+x$ . Then

$$ \begin{align*} \|u-v\|\leq\|u+v\|. \end{align*} $$

It follows from Proposition 2.4 and Lemma 2.6 that there are $\alpha _2,\beta _2\in \{-1,1\}$ such that

$$ \begin{align*} f(u+v)=\alpha_2f(u)+\beta_2f(v), \end{align*} $$

that is, $f(2y)=\alpha _2f(y-x)+\beta _2f(x+y)$ . This combined with (2.6) yields

$$ \begin{align*} f(x+y)=\alpha f(x)+\theta f(y) \end{align*} $$

for some $\alpha \in \{-1,1\}$ and $\theta \in \{\pm 1,\pm 3\}$ . To complete the proof, it remains to exclude the possibility that $|\theta |=3$ . If $|\theta |=3$ , Lemma 2.5 and the strict convexity of Y imply that

$$ \begin{align*} \|x+y\|=\|f(x+y)\|=\|\alpha f(x)+\theta f(y)\|>3\|f(y)\|-\|f(x)\|\geq2\|y\|. \end{align*} $$

This contradiction gives the desired conclusion.

To deal with the case of dimension two, we need one more lemma.

Lemma 2.9. Assume that $X, Y$ are normed spaces with Y being strictly convex, and suppose that $f:X\rightarrow Y$ is a min-phase-isometry with $f(0)=0$ . Then for all $x,y\in X$ and $a,b\in \mathbb {R}$ , there is a $\theta \in \{-1,1\}$ such that $f(ax+by)=\theta (\alpha a f(x)+\beta b f(y))$ where $\alpha ,\beta \in \{-1,1\}$ satisfy $f(x+y)=\alpha f(x)+ \beta f(y)$ .

Proof. We may assume that $x,y$ are linearly independent and $a,b$ are nonzero. Proposition 2.8 yields the existence of $\alpha ,\beta \in \{-1,1\}$ satisfying $f(x+y)=\alpha f(x)+ \beta f(y)$ . We conclude from Proposition 2.8 and Lemma 2.6 that there are $\alpha _1, \beta _1 \in \{-1,1\}$ such that

(2.7) $$ \begin{align} f(ax+by)=\alpha_1 a f(x)+\beta_1 b f(y). \end{align} $$

However, Proposition 2.8 and Lemma 2.6 again imply

$$ \begin{align*} f(ax+by)= f(x+y+(a-1)x+(b-1)y)=\alpha_2f(x+y)+\beta_2(f(a-1)x+(b-1)y) \end{align*} $$

for some $\alpha _2, \beta _2\in \{-1,1\}$ . Continuing in this way produces $\alpha _3, \beta _3\in \{-1,1\}$ such that

(2.8) $$ \begin{align} f(ax+by)&=\alpha_2f(x+y)+\beta_2(f(a-1)x+(b-1)y) \nonumber \\ &=\alpha_2(\alpha f(x)+ \beta f(y))+\beta_2 (\alpha_3 (a-1)f(x)+\beta_3(b-1)f(y)) \nonumber \\ &=(\alpha_2 \alpha + \beta_2 \alpha_3(a-1))f(x)+(\alpha_2\beta+ \beta_2\beta_3(b-1))f(y). \end{align} $$

Since $f(x)$ and $f(y)$ are linearly independent from Lemma 2.7, we obtain from (2.7) and (2.8) that

$$ \begin{align*} \alpha_1=\alpha_2 \alpha \quad \mbox{and} \quad \beta_1 =\alpha_2\beta. \end{align*} $$

The proof is complete.

For our main result in vector spaces of dimension greater than two, we will introduce the fundamental theorem of projective geometry [3, Theorem 3] in the required version. For all $x,y\in X$ , set $[x,y]:=\mbox {span}\{x,y\}$ .

Theorem 2.10 (The fundamental theorem of projective geometry).

Let $X, Y$ be real linear spaces of dimension greater than two and let $f: X\rightarrow Y$ be a map such that

1. (a) $\mathrm{dim}(f(X))\geq 3$ ;

2. (b) if $z\in [x,y]$ , then $f(z)\in [f(x), f(y)].$

Then there is an injective linear mapping $T:X\rightarrow Y$ satisfying

$$ \begin{align*} \mathrm{span}\{f(x)\}=\mathrm{span}\{T(x)\} \quad (\mbox{for all } x\in X). \end{align*} $$

Now we can present the proof of Theorem 1.1. Except in dimension two, we follow the proof of [10, Theorem 2.11] or [8, Proposition 2.4].

Proof of Theorem 1.1.

If $\dim X=1$ , choose a norm-one ${x_0\in X}$ . For every ${\lambda \in \mathbb {R}}$ , define $U:X\rightarrow Y$ by $U(\lambda x_0)=\lambda f(x_0)$ . Then U is a linear isometry which is phase-equivalent to f by Lemma 2.6.

For $\dim X=2$ , fix two linearly independent vectors $x, y\in X$ . By Proposition 2.8, there are $\alpha ,\beta \in \{-1,1\}$ such that

$$ \begin{align*} f(x+y)=\alpha f(x)+\beta f(y). \end{align*} $$

Then we can define a map $U:X\rightarrow Y$ by

$$ \begin{align*} U(ax+by)=a\alpha f(x)+b\beta f(y) \end{align*} $$

for all $a,b\in \mathbb {R}$ . It is clear that U is linear and, by Lemma 2.9, U is phase-equivalent to f. Thus, $\|U(ax+by)\|=\|f(ax+by)\|=\|ax+by\|$ .

Let $\dim X\geq 3$ and let $x,y,z\in X$ be linearly independent. We will show that $f(x),f(y)$ and $f(z)$ are also linearly independent. Assume that this is not true. Then there are nonzero $a,b\in \mathbb {R}$ such that $f(z)=af(x)+bf(y)$ . We deduce from this and Lemma 2.9 that

$$ \begin{align*}f(z)=af(x)+bf(y)\in \{\pm f(ax+by),\pm f(ax-by)\}.\end{align*} $$

Since f is a min-phase-isometry, we see that

$$ \begin{align*} z\in \{\pm (ax+by),\pm(ax-by)\}. \end{align*} $$

This is a contradiction. It follows that the dimension of $f(X)$ is at least three. This combined with Proposition 2.8 guarantees that the conditions of the fundamental theorem of projective geometry are satisfied. So we conclude that f is induced by an injective linear mapping $A:X\rightarrow Y$ , that is, for each $x\in X$ , there is a real number $\lambda _x$ such that

(2.9) $$ \begin{align} f(x)=\lambda_x A(x). \end{align} $$

Hence, for any $y\in X$ , we have

$$ \begin{align*} f(x+y)=\lambda_{x+y}A(x+y)=\lambda_{x+y}A(x)+\lambda_{x+y}A(y). \end{align*} $$

It follows from Proposition 2.8 that

$$ \begin{align*} f(x+y)=\alpha f(x)+\beta f(y)= \alpha\lambda_x A(x)+\beta\lambda_y A(y) \end{align*} $$

with $\alpha ,\beta \in \{-1,1\}$ . Thus,

$$ \begin{align*} |\lambda_y|=|\lambda_{x+y}|=|\lambda_x|. \end{align*} $$

Therefore, $|\lambda _x|$ is a constant independent of x and we denote it by $\lambda $ . Let $U=\lambda A$ . Then by (2.9), U is a linear isometry and is phase-equivalent to f. This completes the proof when $\dim X\geq 3$ .