Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-12T19:42:57.411Z Has data issue: false hasContentIssue false
  • English
  • Français

LIMITING BEHAVIOUR FOR ARRAYS OF UPPER EXTENDED NEGATIVELY DEPENDENT RANDOM VARIABLES

Part of: Limit theorems

Published online by Cambridge University Press:  13 May 2015

JOÃO LITA DA SILVA
JOÃO LITA DA SILVA*
Affiliation:
Department of Mathematics, Faculty of Sciences and Technology, New University of Lisbon, Quinta da Torre, 2829-516 Caparica, Portugal email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

For triangular arrays 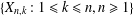$\{X_{n,k}:1\leqslant k\leqslant n,n\geqslant 1\}$ of upper extended negatively dependent random variables weakly mean dominated by a random variable 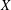$X$ and sequences $\{b_{n}\}$ of positive constants, conditions are given to guarantee an almost sure finite upper bound to 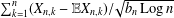$\sum _{k=1}^{n}(X_{n,k}-\mathbb{E}X_{n,k})/\!\sqrt{b_{n}\,\text{Log}\,n}$, where 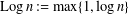$\text{Log}\,n:=\max \{1,\log n\}$, thus getting control over the limiting rate in terms of the prescribed sequence 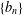$\{b_{n}\}$ and permitting us to weaken or strengthen the assumptions on the random variables.

Keywords

upper extended negatively dependent arrayslaw of the logarithmBernstein inequality

MSC classification

Primary: 60F15: Strong theorems
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 92 , Issue 1 , August 2015 , pp. 159 - 167
Copyright
© 2015 Australian Mathematical Publishing Association Inc. 

References

Bingham, N. H., Goldie, C. M. and Teugels, J. L., Regular Variation (Cambridge University Press, Cambridge, 1987).CrossRefGoogle Scholar
Chen, Y., Chen, A. and Ng, K. W., ‘The strong law of large numbers for extended negatively dependent random variables’, J. Appl. Probab. 47 (2010), 908–922.CrossRefGoogle Scholar
Chow, Y. S. and Teicher, H., Probability Theory: Independence, Interchangeability, Martingales, 3rd edn. (Springer, New York, 1997).CrossRefGoogle Scholar
Gut, A., ‘Complete convergence for arrays’, Period. Math. Hungar. 25(1) (1992), 51–75.CrossRefGoogle Scholar
Hoffmann-Jørgensen, J., Miao, Y., Li, X. C. and Xu, S. F., ‘Kolmogorov type law of the logarithm for arrays’, J. Theoret. Probab., to appear, doi:10.1007/s10959-014-0574-8.CrossRefGoogle Scholar
Hu, T. C., Móricz, F. and Taylor, R. L., ‘Strong laws of large numbers for arrays of rowwise independent random variables’, Acta Math. Hungar. 54 (1989), 153–162.CrossRefGoogle Scholar
Hu, T. C. and Weber, N. C., ‘On the rate of convergence in the strong law of large numbers for arrays’, Bull. Aust. Math. Soc. 45 (1992), 479–482.CrossRefGoogle Scholar
Li, D., Rao, M. B. and Wang, X. C., ‘On the strong law of large numbers and the law of the logarithm for weighted sums of independent random variables with multidimensional indices’, J. Multivariate Anal. 52 (1995), 181–198.CrossRefGoogle Scholar
Petrov, V. V., Limit Theorems of Probability Theory: Sequences of Independent Random Variables, Oxford Studies in Probability, 4 (Oxford Science, Oxford, 1995).Google Scholar
Qi, Y. C., ‘On strong convergence of arrays’, Bull. Aust. Math. Soc. 50 (1994), 219–223.CrossRefGoogle Scholar
Sung, S. H., ‘An analogue of Kolmogorov’s law of the iterated logarithm for arrays’, Bull. Aust. Math. Soc. 54 (1996), 177–182.CrossRefGoogle Scholar
Sung, S. H., ‘On complete convergence for weighted sums of arrays of dependent random variables’, Abstr. Appl. Anal. 11 (2011), 11 pages, doi:10.1155/2011/630583.Google Scholar