Article contents
LEE MONOIDS ARE NONFINITELY BASED WHILE THE SETS OF THEIR ISOTERMS ARE FINITELY BASED
Published online by Cambridge University Press: 28 March 2018
Abstract
We establish a new sufficient condition under which a monoid is nonfinitely based and apply this condition to Lee monoids 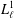 $L_{\ell }^{1}$, obtained by adjoining an identity element to the semigroup generated by two idempotents
$L_{\ell }^{1}$, obtained by adjoining an identity element to the semigroup generated by two idempotents  $a$ and
$a$ and 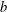 $b$ with the relation
$b$ with the relation 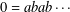 $0=abab\cdots \,$ (length
$0=abab\cdots \,$ (length 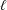 $\ell$). We show that every monoid
$\ell$). We show that every monoid 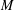 $M$ which generates a variety containing
$M$ which generates a variety containing 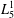 $L_{5}^{1}$ and is contained in the variety generated by
$L_{5}^{1}$ and is contained in the variety generated by 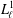 $L_{\ell }^{1}$ for some
$L_{\ell }^{1}$ for some 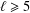 $\ell \geq 5$ is nonfinitely based. We establish this result by analysing
$\ell \geq 5$ is nonfinitely based. We establish this result by analysing  $\unicode[STIX]{x1D70F}$-terms for
$\unicode[STIX]{x1D70F}$-terms for 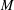 $M$, where
$M$, where  $\unicode[STIX]{x1D70F}$ is a certain nontrivial congruence on the free semigroup. We also show that if
$\unicode[STIX]{x1D70F}$ is a certain nontrivial congruence on the free semigroup. We also show that if  $\unicode[STIX]{x1D70F}$ is the trivial congruence on the free semigroup and
$\unicode[STIX]{x1D70F}$ is the trivial congruence on the free semigroup and 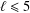 $\ell \leq 5$, then the
$\ell \leq 5$, then the  $\unicode[STIX]{x1D70F}$-terms (isoterms) for
$\unicode[STIX]{x1D70F}$-terms (isoterms) for  $L_{\ell }^{1}$ carry no information about the nonfinite basis property of
$L_{\ell }^{1}$ carry no information about the nonfinite basis property of 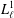 $L_{\ell }^{1}$.
$L_{\ell }^{1}$.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © 2018 Australian Mathematical Publishing Association Inc.
References
- 8
- Cited by


