Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Greim, P.
and
Jamison, J. E.
1987.
Hilbert spaces have the strong Banach-stone property for Bochner spaces.
Mathematische Zeitschrift,
Vol. 196,
Issue. 4,
p.
511.
Fleming, R. J.
and
Jamison, J. E.
1989.
Hermitian operators and isometries on sums of Banach spaces.
Proceedings of the Edinburgh Mathematical Society,
Vol. 32,
Issue. 2,
p.
169.
Cambern, Michael
Jarosz, Krzysztof
and
Wodinski, Georg
1989.
Almost-Lp-projections and Lp isomorphisms.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 113,
Issue. 1-2,
p.
13.
Greim, Peter
Jamison, James E.
and
Kamińska, Anna
1994.
Almost transitivity of some function spaces.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 116,
Issue. 3,
p.
475.


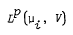 induces a Boolean isomorphism between the measure algebras
induces a Boolean isomorphism between the measure algebras 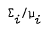 , thus generalizing a result of Cambern's for separable Hilbert spaces.
, thus generalizing a result of Cambern's for separable Hilbert spaces.