Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Wan, James G.
2014.
Hypergeometric generating functions and series for 1/.
ACM Communications in Computer Algebra,
Vol. 47,
Issue. 3/4,
p.
114.
Cooper, Shaun
2017.
Ramanujan's Theta Functions.
p.
613.
Cooper, Shaun
2017.
Ramanujan's Theta Functions.
p.
423.
Cooper, Shaun
2017.
Ramanujan's Theta Functions.
p.
467.
Cooper, Shaun
Wan, James G.
and
Zudilin, Wadim
2017.
Analytic Number Theory, Modular Forms and q-Hypergeometric Series.
Vol. 221,
Issue. ,
p.
179.
Luck, J M
2017.
Equilibration properties of small quantum systems: further examples.
Journal of Physics A: Mathematical and Theoretical,
Vol. 50,
Issue. 35,
p.
355301.
Fowler, J.
Ogle, C.
and
Bevis, M.
2019.
An Analytic Method for Computing the Infinite Sums Occurring in the Geoelastic Disk Load Problem.
Journal of Geophysical Research: Solid Earth,
Vol. 124,
Issue. 2,
p.
2184.
Straub, Armin
and
Zudilin, Wadim
2020.
From Analysis to Visualization.
Vol. 313,
Issue. ,
p.
423.
Sun, Zhi-Wei
2020.
New series for powers of <inline-formula><tex-math id="M1">$ \pi $</tex-math></inline-formula> and related congruences.
Electronic Research Archive,
Vol. 28,
Issue. 3,
p.
1273.
Wang, Liuquan
and
Yang, Yifan
2022.
Ramanujan-type $$1/\pi $$-series from bimodular forms.
The Ramanujan Journal,
Vol. 59,
Issue. 3,
p.
831.
Sivaraman, R.
López-Bonilla, J.
and
Macin-Moran, E. N.
2023.
On the Persson-Strang’s Identity for the Legendre Polynomials.
European Journal of Theoretical and Applied Sciences,
Vol. 1,
Issue. 6,
p.
246.
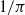 $1/ \pi $ which was experimentally observed by Sun.
$1/ \pi $ which was experimentally observed by Sun.

