Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-18T14:51:36.586Z Has data issue: false hasContentIssue false
  • English
  • Français

EXISTENCE OF RESOLVENT FOR VOLTERRA INTEGRAL EQUATIONS ON TIME SCALES

Part of: Volterra integral equations

Published online by Cambridge University Press:  17 March 2010

MURAT ADıVAR  and
YOUSSEF N. RAFFOUL
MURAT ADıVAR*
Affiliation:
Izmir University of Economics, Department of Mathematics, 35330 Izmir, Turkey (email: [email protected])
YOUSSEF N. RAFFOUL
Affiliation:
University of Dayton, Department of Mathematics, Dayton, OH 45469-2316, USA (email: [email protected])
*
✉For correspondence; e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

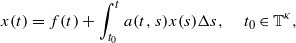We introduce the concept of ‘shift operators’ in order to establish sufficient conditions for the existence of the resolvent for the Volterra integral equation on time scales. The paper will serve as the foundation for future research on the qualitative analysis of solutions of Volterra integral equations on time scales, using the notion of the resolvent.

Keywords

existenceresolventshift operatortime scalesVolterra integral equation

MSC classification

Secondary: 45D05: Volterra integral equations
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 82 , Issue 1 , August 2010 , pp. 139 - 155
Copyright
Copyright © Australian Mathematical Publishing Association Inc. 2010

Footnotes

This work was supported by the Scientific and Technological Research Council of Turkey.

References

[1]Bohner, M. and Doslý, O., ‘Oscillation of symplectic dynamic systems’, ANZIAM J. 46 (2004), 17–32.CrossRefGoogle Scholar
[2]Bohner, M. and Guseinov, G., ‘Riemann and Lebesgue integration’, Advances in Dynamic Equations on Time Scales (Birkhäuser, Boston, MA, 2003).CrossRefGoogle Scholar
[3]Bohner, M. and Guseinov, G., ‘Multiple integration on time scales’, Dynam. Systems Appl. 14(3–4) (2005), 579–606.Google Scholar
[4]Bohner, M. and Guseinov, G., ‘Double integral calculus of variations on time scales’, Comput. Math. Appl. 54(1) (2007), 45–57.CrossRefGoogle Scholar
[5]Bohner, M. and Peterson, A., ‘Dynamic equations on time scales’, An Introduction with Applications (Birkhäuser, Boston, MA, 2001).Google Scholar
[6]Bohner, M. and Peterson, A., Advances in Dynamic Equations on Time Scales (Birkhäuser, Boston, MA, 2003).CrossRefGoogle Scholar
[7]Hilger, S., ‘Analysis on measure chains: a unified approach to continuous and discrete calculus’, Results Math. 18 (1990), 18–56.CrossRefGoogle Scholar
[8]Karpuz, B., ‘Unbounded oscillation of higher-order nonlinear delay dynamic equations of neutral type with oscillating coefficients’, Electron J. Qual. Theory Differ. Equ. 34(1–14) (2009).Google Scholar
[9]Kulik, T. and Tisdell, C. C., ‘Volterra integral equations on time scales: basic qualitative and quantitative results with applications to initial value problems on unbounded domains’, Int. J. Difference Equ. 3(1) (2008), 103–133.Google Scholar
[10]Miller, R. K., Nonlinear Volterra Integral Equations (W. A. Benjamin, Menlo Park, CA, 1971).Google Scholar
[11]Tisdell, C. C. and Zaidi, A., ‘Basic qualitative and quantitative results for solutions to nonlinear, dynamic equations on time scales with an application to economic modelling’, Nonlinear Anal. 68(11) (2008), 3504–3524.CrossRefGoogle Scholar
[12]Zafer, A., ‘The exponential of a constant matrix on time scales’, ANZIAM J. 48 (2006), 99–106.CrossRefGoogle Scholar