No CrossRef data available.
Article contents
EMBEDDINGS AND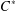 
$C^{\ast }$-ENVELOPES OF EXACT OPERATOR SYSTEMS
Published online by Cambridge University Press: 02 May 2017
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
We prove a necessary and sufficient condition for embeddability of an operator system into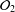 
${\mathcal{O}}_{2}$. Using Kirchberg’s theorems on a tensor product of 
${\mathcal{O}}_{2}$ and 
${\mathcal{O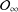}}_{\infty }$, we establish results on their operator system counterparts 
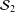${\mathcal{S}}_{2}$ and 
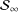${\mathcal{S}}_{\infty }$. Applications of the results, including some examples describing 
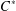$C^{\ast }$-envelopes of operator systems, are also discussed.
- Type
- Research Article
- Information
- Bulletin of the Australian Mathematical Society , Volume 96 , Issue 2 , October 2017 , pp. 274 - 285
- Copyright
- © 2017 Australian Mathematical Publishing Association Inc.
References
Argerami, M. and Farenick, D., ‘The C*-envelope of an irreducible periodic weighted unilateral shift’, Integral Equations Operator Theory
77(2) (2013), 199–210.Google Scholar
Argerami, M. and Farenick, D., ‘C*-envelopes of Jordan operator systems’, Oper. Matrices
9(2) (2015), 325–341.Google Scholar
Bratteli, O., Elliott, G. A., Evans, D. E. and Kishimoto, A., ‘On the classification of C
∗ -algebras of real rank zero III. The infinite case’, in: Operator Algebras and their Applications, II (Waterloo, Ontario, 1994/1995), Fields Institute Comm., 20 (1998), 11–72.Google Scholar
Cuntz, J., ‘Simple C*-algebra generated by isometries’, Comm. Math. Phys.
57(2) (1977), 173–185.Google Scholar
Farenick, D., Kavruk, A. S., Paulsen, V. I. and Todorov, I. G., ‘Operator systems from discrete groups’, Comm. Math. Phys.
329(1) (2014), 207–238.Google Scholar
Gupta, V. P. and Luthra, P., ‘Operator system nuclearity via C
∗ -envelopes’, J. Aust. Math. Soc.
101(3) (2016), 356–375.Google Scholar
Hamana, M., ‘Injective envelopes of operator systems’, Publ. Res. Inst. Math. Sci.
15(3) (1979), 773–785.Google Scholar
Kavruk, A. S., ‘Nuclearity related properties in operator systems’, J. Operator Theory
71(1) (2014), 95–156.Google Scholar
Kavruk, A. S., Paulsen, V. I., Todorov, I. G. and Tomforde, M., ‘Tensor products of operator systems’, J. Funct. Anal.
261(2) (2011), 267–299.Google Scholar
Kavruk, A. S., Paulsen, V. I., Todorov, I. G. and Tomforde, M., ‘Quotients, exactness, and nuclearity in the operator system category’, Adv. Math.
235 (2013), 321–360.Google Scholar
Kirchberg, E. and Phillips, C. N., ‘Embedding of exact C
∗ -algebras into 𝓞2
’, J. reine angew. Math.
525 (2000), 17–53.CrossRefGoogle Scholar
Kirchberg, E. and Wassermann, S., ‘C*-algebras generated by operator systems’, J. Funct. Anal.
155(2) (1998), 324–351.Google Scholar
Lupini, M., ‘Fraïssé limits in functional analysis’, Preprint, 2015, arXiv:1510.05188.Google Scholar
Lupini, M., ‘Operator space and operator system analogs of Kirchberg’s nuclear embedding theorem’, J. Math. Anal. Appl.
431(1) (2015), 47–56.Google Scholar
Ortiz, C. M. and Paulsen, V. I., ‘Lovász theta type norms and operator systems’, Linear Algebra Appl.
477 (2015), 128–147.Google Scholar
Paulsen, V. I. and Zheng, D., ‘Tensor products of the operator system generated by the Cuntz isometries’, J. Operator Theory
76(1) (2016), 67–91.Google Scholar
Pisier, G., Introduction to Operator Space Theory, London Mathematical Society Lecture Note Series, 294 (Cambridge University Press, Cambridge, 2003).CrossRefGoogle Scholar
Takesaki, M., Theory of Operator Algebras II, Vol. 125 (Springer Science & Business Media, Berlin, 2013).Google Scholar
Zheng, D., ‘The operator system generated by Cuntz isometries’, Preprint, 2014, arXiv:1410.6950.Google Scholar
You have
Access