Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-26T18:03:17.282Z Has data issue: false hasContentIssue false
  • English
  • Français

EMBEDDABILITY OF GENERALISED WREATH PRODUCTS

Part of: Structure and classification of infinite or finite groups Special aspects of infinite or finite groups

Published online by Cambridge University Press:  15 December 2014

CHRIS CAVE  and
DENNIS DREESEN
CHRIS CAVE*
Affiliation:
School of Mathematics, University of Southampton, Highfield, Southampton SO17 1BJ, UK email [email protected]
DENNIS DREESEN
Affiliation:
School of Mathematics, University of Southampton, Highfield, Southampton SO17 1BJ, UK email [email protected]
*
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Given two finitely generated groups that coarsely embed into a Hilbert space, it is known that their wreath product also embeds coarsely into a Hilbert space. We introduce a wreath product construction for general metric spaces 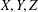$X,Y,Z$ and derive a condition, called the (${\it\delta}$-polynomial) path lifting property, such that coarse embeddability of 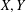$X,Y$ and 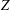$Z$ implies coarse embeddability of 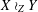$X\wr _{Z}Y$. We also give bounds on the compression of 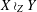$X\wr _{Z}Y$ in terms of ${\it\delta}$ and the compressions of $X,Y$ and $Z$.

Keywords

coarse embeddingcompressionwreath products

MSC classification

Primary: 20F65: Geometric group theory
Secondary: 20E22: Extensions, wreath products, and other compositions 20F69: Asymptotic properties of groups
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 91 , Issue 2 , April 2015 , pp. 250 - 263
Copyright
Copyright © 2014 Australian Mathematical Publishing Association Inc. 

References

Austin, T., ‘Amenable groups with very poor compression into Lebesgue spaces’, Duke Math. J. 159(2) (2011), 187–222.CrossRefGoogle Scholar
Chatterji, I., Druţu, C. and Haglund, F., ‘Kazhdan and Haagerup properties from the median viewpoint’, Adv. Math. 225(2) (2010), 882–921.CrossRefGoogle Scholar
Cherix, P.-A., Cowling, M., Jolissaint, P., Julg, P. and Valette, A., Groups with the Haagerup property: Gromov’s a-T-menability, Progress in Mathematics, 197 (Birkhäuser, Basel, 2001).Google Scholar
Chifan, I. and Ioana, A., ‘On relative property (T) and Haagerup’s property’, Trans. Amer. Math. Soc. 363(12) (2011), 6407–6420.Google Scholar
Dadarlat, M. and Guentner, E., ‘Constructions preserving Hilbert space uniform embeddability of discrete groups’, Trans. Amer. Math. Soc. 355(8) (2003), 3253–3275.CrossRefGoogle Scholar
Dadarlat, M. and Guentner, E., ‘Uniform embeddability of relatively hyperbolic groups’, J. reine angew. Math. 612 (2007), 1–15.CrossRefGoogle Scholar
de Cornulier, Y., Stalder, Y. and Valette, A., ‘Proper actions of wreath products and generalizations’, Trans. Amer. Math. Soc. 364(6) (2012), 3159–3184.CrossRefGoogle Scholar
de Cornulier, Y., Tessera, R. and Valette, A., ‘Isometric group actions on Hilbert spaces: growth of cocycles’, Geom. Funct. Anal. 17(3) (2007), 770–792.CrossRefGoogle Scholar
Erschler, A., ‘Generalized wreath products’, Int. Math. Res. Not. IMRN 2006 (2006), 14 pages; Article ID 57835.Google Scholar
Ferry, S. C., Ranicki, A. and Rosenberg, J. (eds) Novikov Conjectures, Index Theorems and Rigidity. Vol. 1, London Mathematical Society Lecture Note Series, 226 (Cambridge University Press, Cambridge, 1995), including papers from the conference held at the Mathematisches Forschungsinstitut Oberwolfach, Oberwolfach, 6–10 September 1993.Google Scholar
Gromov, M., ‘Asymptotic invariants of infinite groups’, in: Geometric Group Theory, Vol. 2 (Sussex, 1991), London Mathematical Society Lecture Note Series, 182 (Cambridge University Press, Cambridge, 1993), 1–295.Google Scholar
Guentner, E. and Kaminker, J., ‘Exactness and uniform embeddability of discrete groups’, J. Lond. Math. Soc. (2) 70(3) (2004), 703–718.CrossRefGoogle Scholar
Kasparov, G. and Yu, G., ‘The Novikov conjecture and geometry of Banach spaces’, Geom. Topol. 16(3) (2012), 1859–1880.Google Scholar
Li, S., ‘Compression bounds for wreath products’, Proc. Amer. Math. Soc. 138(8) (2010), 2701–2714.CrossRefGoogle Scholar
Skandalis, G., Tu, J. L. and Yu, G., ‘The coarse Baum–Connes conjecture and groupoids’, Topology 41(4) (2002), 807–834.CrossRefGoogle Scholar