Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-24T02:37:51.784Z Has data issue: false hasContentIssue false
  • English
  • Français

Doubly transitive permutation groups involving the one-dimensional projective special linear groups

Published online by Cambridge University Press:  17 April 2009

Cheryl E. Praeger
Cheryl E. Praeger
Affiliation:
Department of Mathematics, Institute of Advanced Studies, Australian National University, Canberra, ACT.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let G be a doubly transitive permutation group on a finite set Ω, and for α in Ω suppose that Gα has a set Σ of non-trivial blocks of imprimitivity in Ω - {α}. If Gα is 2-transitive but not faithful on Σ, when is it true that the stabiliser in Gα of a block of Σ does not act faithfully on that block (that is, there is a nontrivial element in Gα which fixes every point of the block)? In a previous paper this question was answered when 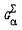is the alternating or symmetric group, or a Mathieu group in its usual representation. In this paper we answer the question when 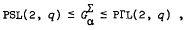, permuting the q + 1 points of the projective line, for some prime power q. We show that the only groups which arise satisfy either

(i) PSL(3, q) ≤ G ≤ PΓL(3, q) in its natural representation, or

(ii) G is a group of collineations of an affine translation plane of order q, and contains the translation group.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 14 , Issue 3 , June 1976 , pp. 349 - 358
Copyright
Copyright © Australian Mathematical Society 1976

References

[1]Dembowski, P., Finite geometries (Ergebnisse der Mathematik und ihrer Grenzgebiete, 44. Springer-Verlag, Berlin, Heidelberg, New York, 1968).CrossRefGoogle Scholar
[2]Gorenstein, Daniel, Finite groups (Harper and Row, New York, Evanston, London, 1968).Google Scholar
[3]Lang, Serge, Algebra (Addison-Wesley, Reading, Massachusetts, 1965).Google Scholar
[4]O'Nan, Michael E., “Normal structure of the one-point stabiliser of a doubly-transitive permutation group, II”, Trans. Amer. Math. Soc. 214 (1975), 43–74.Google Scholar
[5]Ostrom, T.G. and Wagner, A., “On projective and affine planes with transitive collineation groups”, Math. Z. 71 (1959), 186–199.Google Scholar
[6]Praeger, Cheryl E., “Doubly transitive permutation groups which are not doubly primitive”, J. Algebra (to appear).Google Scholar
[7]Wielandt, Helmut, Finite permutation groups (translated by Bercov, R.. Academic Press, New York, London, 1964).Google Scholar
[8]Witt, Ernst, “Die 5-fach transitiven Gruppen von Mathieu”, Abh. Math. Sem. Univ. Hamburg 12 (1938), 256–264.CrossRefGoogle Scholar