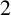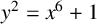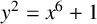1. Introduction
Given a curve defined over a field, its twist is another curve that becomes isomorphic to the given curve over the algebraic closure. For example, an elliptic curve over a field k whose defining equation is
![]() $ y^2 = f(x) $
is isomorphic to a curve defined by the equation
$ y^2 = f(x) $
is isomorphic to a curve defined by the equation
![]() $Dy^2 = f(x)$
over
$Dy^2 = f(x)$
over
![]() $k(\sqrt {D})$
. This is called a quadratic twist of the elliptic curve. In the case of a generic elliptic curve (that is, its j-invariant is not equal to
$k(\sqrt {D})$
. This is called a quadratic twist of the elliptic curve. In the case of a generic elliptic curve (that is, its j-invariant is not equal to
![]() $0, 1728$
), every twist is a quadratic twist.
$0, 1728$
), every twist is a quadratic twist.
For a curve of genus
![]() $\geq 2$
, the notion of the quadratic twist of an elliptic curve can be generalised to the hyperelliptic twist. A hyperelliptic twist of a curve
$\geq 2$
, the notion of the quadratic twist of an elliptic curve can be generalised to the hyperelliptic twist. A hyperelliptic twist of a curve
![]() $y^2 = f(x)$
has an affine equation
$y^2 = f(x)$
has an affine equation
![]() $Dy^2 = f(x)$
for some
$Dy^2 = f(x)$
for some
![]() $D \in k^\times /(k^\times )^2$
. We denote by
$D \in k^\times /(k^\times )^2$
. We denote by
![]() $X^{(D)}$
the hyperelliptic twist of the curve X. Again, X and
$X^{(D)}$
the hyperelliptic twist of the curve X. Again, X and
![]() $X^{(D)}$
become isomorphic over
$X^{(D)}$
become isomorphic over
![]() $k(\sqrt {D})$
. Since the automorphism group of a hyperelliptic curve can be larger than that of an elliptic curve, there are also nonhyperelliptic twists in the genus
$k(\sqrt {D})$
. Since the automorphism group of a hyperelliptic curve can be larger than that of an elliptic curve, there are also nonhyperelliptic twists in the genus
![]() $2$
case. The automorphism group of the genus
$2$
case. The automorphism group of the genus
![]() $2$
curve is one of
$2$
curve is one of
where
![]() $C_n$
is the cyclic group of order n,
$C_n$
is the cyclic group of order n,
![]() $D_n$
is the dihedral group of order n,
$D_n$
is the dihedral group of order n,
![]() $V_4$
is the Klein
$V_4$
is the Klein
![]() $4$
group,
$4$
group,
![]() $\widetilde {S_4}\cong \operatorname {\mathrm {GL}}_2(\mathbb {F}_3)$
is a double cover of the symmetric group
$\widetilde {S_4}\cong \operatorname {\mathrm {GL}}_2(\mathbb {F}_3)$
is a double cover of the symmetric group
![]() $S_4$
and
$S_4$
and
![]() $2D_{12}$
is a double cover of
$2D_{12}$
is a double cover of
![]() $D_{12}$
. The classification of isomorphism classes of curves whose automorphism group is
$D_{12}$
. The classification of isomorphism classes of curves whose automorphism group is
![]() $D_8, D_{12}, \widetilde {S}_4$
and
$D_8, D_{12}, \widetilde {S}_4$
and
![]() $ 2D_{12}$
is studied by Cardona and his collaborators [Reference Cardona1–Reference Cardona and Quer3].
$ 2D_{12}$
is studied by Cardona and his collaborators [Reference Cardona1–Reference Cardona and Quer3].
We consider twists of a genus
![]() $2$
curve defined over
$2$
curve defined over
![]() $\mathbb {Q}$
whose automorphism group is isomorphic to
$\mathbb {Q}$
whose automorphism group is isomorphic to
![]() $2D_{12}$
. There is only one such
$2D_{12}$
. There is only one such
![]() $\overline {\mathbb {Q}}$
-isomorphism class, and a representative is given by the equation
$\overline {\mathbb {Q}}$
-isomorphism class, and a representative is given by the equation
![]() $y^2 = x^6+1$
. From [Reference Cardona and Lario2], the classification of the
$y^2 = x^6+1$
. From [Reference Cardona and Lario2], the classification of the
![]() $\mathbb {Q}$
-isomorphism class of the curve
$\mathbb {Q}$
-isomorphism class of the curve
![]() $y^2 = x^6+1$
is given by two steps: first, the classification of the possible Galois module structures on
$y^2 = x^6+1$
is given by two steps: first, the classification of the possible Galois module structures on
![]() $2D_{12}$
; second, the classification of the curves whose automorphism group has the given Galois module structure.
$2D_{12}$
; second, the classification of the curves whose automorphism group has the given Galois module structure.
For nonhyperelliptic twists, we consider twists whose automorphism group has the Galois module structure defined over a biquadratic extension of
![]() $\mathbb {Q}$
. From [Reference Cardona and Lario2, Section 7]:
$\mathbb {Q}$
. From [Reference Cardona and Lario2, Section 7]:
-
(i) the biquadratic extension of
 $\mathbb {Q}$
should have
$\mathbb {Q}$
should have
 $\mathbb {Q}(\sqrt {-3})$
as a subfield;
$\mathbb {Q}(\sqrt {-3})$
as a subfield; -
(ii) let
 $\mathbb {Q}(\sqrt {d}, \sqrt {-3})$
be the biquadratic extension. Then, there are six types
$\mathbb {Q}(\sqrt {d}, \sqrt {-3})$
be the biquadratic extension. Then, there are six types
 $A, B, \ldots , E, G$
(see (2.2) and the discussion following Definition 2.5) so that any twist whose automorphism group is
$A, B, \ldots , E, G$
(see (2.2) and the discussion following Definition 2.5) so that any twist whose automorphism group is
 $\mathbb {Q}(\sqrt {d}, \sqrt {-3})$
is one of
$\mathbb {Q}(\sqrt {d}, \sqrt {-3})$
is one of
 $V_4^A, \ldots , V_4^E, V_4^G$
(type F does not appear when the base field is
$V_4^A, \ldots , V_4^E, V_4^G$
(type F does not appear when the base field is
 $\mathbb {Q}$
);
$\mathbb {Q}$
); -
(iii) if two curves of the same type also have the same biquadratic extension, then they are hyperelliptic twists of each other.
In short, the
![]() $\mathbb {Q}$
-isomorphism class of a twist is determined by three parameters: a type in
$\mathbb {Q}$
-isomorphism class of a twist is determined by three parameters: a type in
![]() $\{ A, B, \ldots , E, G \}$
, the defining field of the automorphism group
$\{ A, B, \ldots , E, G \}$
, the defining field of the automorphism group
![]() $\mathbb {Q}(\sqrt {d}, \sqrt {-3})$
and a hyperelliptic twist.
$\mathbb {Q}(\sqrt {d}, \sqrt {-3})$
and a hyperelliptic twist.
Types B and C are relatively simple because, in this case, the parameter d has no additional restrictions. We give a concrete decomposition of twists of these types.
Theorem 1.1. Let
![]() $X/\mathbb {Q}$
be a twist of type
$X/\mathbb {Q}$
be a twist of type
![]() $V_4^B$
or
$V_4^B$
or
![]() $V_4^C$
. Then, there are
$V_4^C$
. Then, there are
![]() $d, D \in \mathbb {Q}^\times /(\mathbb {Q}^\times )^2$
such that the Jacobian of X is isogenous to
$d, D \in \mathbb {Q}^\times /(\mathbb {Q}^\times )^2$
such that the Jacobian of X is isogenous to
![]() $E_0^{(-D)}\times E_0^{(-dD)}$
or
$E_0^{(-D)}\times E_0^{(-dD)}$
or
![]() $E_0^{(D)} \times E_0^{(dD)}$
where
$E_0^{(D)} \times E_0^{(dD)}$
where
![]() $E_0^m$
for
$E_0^m$
for
![]() $m\in\mathbb{Q}^\times$
is the elliptic curve given by
$m\in\mathbb{Q}^\times$
is the elliptic curve given by
![]() $my^2=x^3+1$
.
$my^2=x^3+1$
.
The main tool is a computation of L-factors of twists of
![]() $y^2 = x^6+1$
which is done by Fité and Sutherland [Reference Fité and Sutherland5]. In Section 2, we recall previous results on the twists of the curve
$y^2 = x^6+1$
which is done by Fité and Sutherland [Reference Fité and Sutherland5]. In Section 2, we recall previous results on the twists of the curve
![]() $y^2 = x^6+1$
. In Section 3, we study some properties of our twist families. In Section 4, we give a proof of the main theorem.
$y^2 = x^6+1$
. In Section 3, we study some properties of our twist families. In Section 4, we give a proof of the main theorem.
2. Preliminaries
In this section, we recall the classification [Reference Cardona and Lario2] of twists of the curve
![]() $y^2 = x^6+1$
, with an emphasis on the biquadratic case, and some previous results of [Reference Fité and Sutherland5].
$y^2 = x^6+1$
, with an emphasis on the biquadratic case, and some previous results of [Reference Fité and Sutherland5].
For a curve X over a field k, we say that another curve
![]() $X'$
over k is a twist of X if
$X'$
over k is a twist of X if
![]() $X'$
becomes isomorphic to X over the algebraic closure
$X'$
becomes isomorphic to X over the algebraic closure
![]() $\overline {k}$
of k. The set of k-isomorphism classes of X is denoted by
$\overline {k}$
of k. The set of k-isomorphism classes of X is denoted by
![]() $\operatorname {\mathrm {Twist}}(X/k)$
. In what follows, we abbreviate
$\operatorname {\mathrm {Twist}}(X/k)$
. In what follows, we abbreviate
![]() $\operatorname {\mathrm {Aut}}_{\overline {k}}(X):=\operatorname {\mathrm {Aut}}_{\overline {k}}(X_{\overline {k}})$
. It is well known (see, for example, [Reference Silverman6, Theorem X.2.2]) that there is a canonical bijection
$\operatorname {\mathrm {Aut}}_{\overline {k}}(X):=\operatorname {\mathrm {Aut}}_{\overline {k}}(X_{\overline {k}})$
. It is well known (see, for example, [Reference Silverman6, Theorem X.2.2]) that there is a canonical bijection
From now on, let
![]() $X_0/\mathbb {Q}$
denote the genus
$X_0/\mathbb {Q}$
denote the genus
![]() $2$
curve defined by the equation
$2$
curve defined by the equation
![]() $ y^2 = x^6+1$
. We recall that
$ y^2 = x^6+1$
. We recall that
![]() $\operatorname {\mathrm {Aut}}_{\overline {\mathbb {Q}}}(X_0)$
is isomorphic to
$\operatorname {\mathrm {Aut}}_{\overline {\mathbb {Q}}}(X_0)$
is isomorphic to
![]() $2D_{12}$
as an abstract group. Following [Reference Cardona and Lario2], we use a group presentation
$2D_{12}$
as an abstract group. Following [Reference Cardona and Lario2], we use a group presentation
which is isomorphic to
![]() $2D_{12}$
as a group. Its automorphism group is isomorphic to
$2D_{12}$
as a group. Its automorphism group is isomorphic to
![]() $C_2 \times D_{12}$
. We specify the elements of
$C_2 \times D_{12}$
. We specify the elements of
![]() $\operatorname {\mathrm {Aut}}(A)$
as follows:
$\operatorname {\mathrm {Aut}}(A)$
as follows:
![]() $\iota , \jmath $
are two central involutions, s the noncentral involution, t the automorphism of order
$\iota , \jmath $
are two central involutions, s the noncentral involution, t the automorphism of order
![]() $3$
with relation
$3$
with relation
![]() $ts = st^2$
(see [Reference Cardona and Lario2, Section 3]).
$ts = st^2$
(see [Reference Cardona and Lario2, Section 3]).
Suppose that X is a twist of
![]() $X_0$
. Then
$X_0$
. Then
![]() $\operatorname {\mathrm {Aut}}_{\overline {\mathbb {Q}}}(X)$
is also isomorphic to
$\operatorname {\mathrm {Aut}}_{\overline {\mathbb {Q}}}(X)$
is also isomorphic to
![]() $2D_{12}$
as a group, so we first consider possible
$2D_{12}$
as a group, so we first consider possible
![]() $G_{\mathbb {Q}}$
-module structures on A. Since giving a
$G_{\mathbb {Q}}$
-module structures on A. Since giving a
![]() $G_{\mathbb {Q}}$
-structure on A is equivalent to giving a morphism
$G_{\mathbb {Q}}$
-structure on A is equivalent to giving a morphism
![]() $G_{\mathbb {Q}} \to \operatorname {\mathrm {Aut}}(A)$
, we concentrate on the latter. Let K be the field of definition of the
$G_{\mathbb {Q}} \to \operatorname {\mathrm {Aut}}(A)$
, we concentrate on the latter. Let K be the field of definition of the
![]() $G_{\mathbb {Q}}$
-structure on A so that
$G_{\mathbb {Q}}$
-structure on A so that
for a certain subgroup H in
![]() $C_2 \times D_{12}$
. Then, a
$C_2 \times D_{12}$
. Then, a
![]() $G_{\mathbb {Q}}$
-structure on A is determined by
$G_{\mathbb {Q}}$
-structure on A is determined by
![]() $K, H$
and a group isomorphism between H and
$K, H$
and a group isomorphism between H and
![]() $\operatorname {\mathrm {Gal}}(K/\mathbb {Q})$
. To describe the extension
$\operatorname {\mathrm {Gal}}(K/\mathbb {Q})$
. To describe the extension
![]() $K/\mathbb {Q}$
, we need to define some subfields of K. We consider subgroups of H, which are
$K/\mathbb {Q}$
, we need to define some subfields of K. We consider subgroups of H, which are
They are index
![]() $1$
or
$1$
or
![]() $2$
, so induce a quadratic or trivial extension of
$2$
, so induce a quadratic or trivial extension of
![]() $\mathbb {Q}$
. We denote them by
$\mathbb {Q}$
. We denote them by
In other words, we define
![]() $u, v, v' \in \mathbb {Q}^\times /(\mathbb {Q}^\times )^2$
determined by a
$u, v, v' \in \mathbb {Q}^\times /(\mathbb {Q}^\times )^2$
determined by a
![]() $G_{\mathbb {Q}}$
-action on A.
$G_{\mathbb {Q}}$
-action on A.
Since we only consider a biquadratic extension
![]() $K/\mathbb {Q}$
, the subgroup H is isomorphic to
$K/\mathbb {Q}$
, the subgroup H is isomorphic to
![]() $V_4$
. We recall that there are seven subgroups of
$V_4$
. We recall that there are seven subgroups of
![]() $C_2 \times 2D_{12}$
isomorphic to
$C_2 \times 2D_{12}$
isomorphic to
![]() $V_4$
:
$V_4$
:
They correspond to the type
![]() $V_4^{\bullet }$
for
$V_4^{\bullet }$
for
![]() $\bullet \in \{ A, \ldots , G \}$
(see [Reference Cardona and Lario2, Table 3]). To describe a specific isomorphism (in
$\bullet \in \{ A, \ldots , G \}$
(see [Reference Cardona and Lario2, Table 3]). To describe a specific isomorphism (in
![]() $6=|\operatorname {\mathrm {Aut}}(V_4)|$
-choices) between
$6=|\operatorname {\mathrm {Aut}}(V_4)|$
-choices) between
![]() $\operatorname {\mathrm {Gal}}(K/\mathbb {Q})$
and H, it suffices to give quadratic subfields of K corresponding to the generators of H. For instance, we consider
$\operatorname {\mathrm {Gal}}(K/\mathbb {Q})$
and H, it suffices to give quadratic subfields of K corresponding to the generators of H. For instance, we consider
![]() $V_4^A$
, which corresponds to
$V_4^A$
, which corresponds to
![]() $\langle \iota , \jmath \rangle \leq \operatorname {\mathrm {Aut}}(A)$
. Then an isomorphism between
$\langle \iota , \jmath \rangle \leq \operatorname {\mathrm {Aut}}(A)$
. Then an isomorphism between
![]() $\operatorname {\mathrm {Gal}}(K/\mathbb {Q})$
and
$\operatorname {\mathrm {Gal}}(K/\mathbb {Q})$
and
![]() $\langle \iota , \jmath \rangle $
can be described by
$\langle \iota , \jmath \rangle $
can be described by
![]() $K^{\langle \iota \rangle }, K^{\langle \jmath \rangle }$
, which are quadratic extensions of
$K^{\langle \iota \rangle }, K^{\langle \jmath \rangle }$
, which are quadratic extensions of
![]() $\mathbb {Q}$
.
$\mathbb {Q}$
.
We next describe a constraint for our
![]() $G_{\mathbb {Q}}$
-module A.
$G_{\mathbb {Q}}$
-module A.
Lemma 2.1 [Reference Cassels and Flynn4, Ch. 1].
If
![]() $X/k$
is of genus
$X/k$
is of genus
![]() $2$
, then there is an injection
$2$
, then there is an injection
![]() $\operatorname {\mathrm {Aut}}_{\overline {k}}(X)\hookrightarrow \operatorname {\mathrm {GL}}_2(\overline {k})$
of
$\operatorname {\mathrm {Aut}}_{\overline {k}}(X)\hookrightarrow \operatorname {\mathrm {GL}}_2(\overline {k})$
of
![]() $G_k$
-groups.
$G_k$
-groups.
By Lemma 2.1, we only consider
![]() $G_{\mathbb {Q}}$
-subgroups of
$G_{\mathbb {Q}}$
-subgroups of
![]() $\operatorname {\mathrm {GL}}_2(\overline {\mathbb {Q}})$
whose underlying groups are isomorphic to
$\operatorname {\mathrm {GL}}_2(\overline {\mathbb {Q}})$
whose underlying groups are isomorphic to
![]() $2D_{12}$
. To get a more explicit condition using the parameters in (2.1), we introduce the following definition.
$2D_{12}$
. To get a more explicit condition using the parameters in (2.1), we introduce the following definition.
Definition 2.2. If
![]() $u,v \in \mathbb {Q}$
satisfy
$u,v \in \mathbb {Q}$
satisfy
![]() $(u, -3v) = 1 \in \mathrm {Br}_2(\mathbb {Q})$
, then by [Reference Cardona and Lario2, Remark 1],
$(u, -3v) = 1 \in \mathrm {Br}_2(\mathbb {Q})$
, then by [Reference Cardona and Lario2, Remark 1],
has solutions in
![]() $\mathbb {Q}^\times $
. Once solutions
$\mathbb {Q}^\times $
. Once solutions
![]() $\alpha ,\beta \in \mathbb {Q}^\times $
are chosen, we define constants
$\alpha ,\beta \in \mathbb {Q}^\times $
are chosen, we define constants
 $$ \begin{align*} z = 4 \alpha^3 - 3u\alpha, \quad s = \frac{u^2 - 2\alpha^2u + \alpha z}{3\beta}. \end{align*} $$
$$ \begin{align*} z = 4 \alpha^3 - 3u\alpha, \quad s = \frac{u^2 - 2\alpha^2u + \alpha z}{3\beta}. \end{align*} $$
Theorem 2.3 [Reference Cardona and Lario2, Theorem 2].
Let A be a
![]() $G_{\mathbb {Q}}$
-group with underlying group isomorphic to
$G_{\mathbb {Q}}$
-group with underlying group isomorphic to
![]() $2D_{12}$
, K the defining field of the
$2D_{12}$
, K the defining field of the
![]() $G_{\mathbb {Q}}$
-module structure on A which is a biquadratic extension of
$G_{\mathbb {Q}}$
-module structure on A which is a biquadratic extension of
![]() $\mathbb {Q}$
,
$\mathbb {Q}$
,
![]() $K_i$
the subfield of K defined by (2.1) for
$K_i$
the subfield of K defined by (2.1) for
![]() $i=1, 2, 3$
, and
$i=1, 2, 3$
, and
![]() $u, v, v'$
elements in
$u, v, v'$
elements in
![]() $\mathbb {Q}^\times /(\mathbb {Q}^\times )^2$
also defined by (2.1). Then A can be embedded in
$\mathbb {Q}^\times /(\mathbb {Q}^\times )^2$
also defined by (2.1). Then A can be embedded in
![]() $\operatorname {\mathrm {GL}}_2(\overline {\mathbb {Q}})$
if and only if
$\operatorname {\mathrm {GL}}_2(\overline {\mathbb {Q}})$
if and only if
![]() $(u, -3v) = 1 \in \mathrm {Br}_2(\mathbb {Q})$
and
$(u, -3v) = 1 \in \mathrm {Br}_2(\mathbb {Q})$
and
![]() $v' \equiv -3v \pmod {\mathbb {Q}^{\times 2}}$
. In this case,
$v' \equiv -3v \pmod {\mathbb {Q}^{\times 2}}$
. In this case,
![]() $A \cong \langle U, V, -1 \rangle $
, where
$A \cong \langle U, V, -1 \rangle $
, where
 $$ \begin{align*} U = \frac{1}{\sqrt{u}} \begin{pmatrix} \alpha & \beta \\ {3\beta}/{v} & -\alpha \end{pmatrix}, \quad V = \frac{\sqrt{-3}}{2} \begin{pmatrix} 1 & {\sqrt{v}}/{3} \\ -{1}/{\sqrt{v}} & 1 \end{pmatrix}, \quad -1 = \begin{pmatrix} -1 & 0 \\ 0 & -1 \end{pmatrix} \end{align*} $$
$$ \begin{align*} U = \frac{1}{\sqrt{u}} \begin{pmatrix} \alpha & \beta \\ {3\beta}/{v} & -\alpha \end{pmatrix}, \quad V = \frac{\sqrt{-3}}{2} \begin{pmatrix} 1 & {\sqrt{v}}/{3} \\ -{1}/{\sqrt{v}} & 1 \end{pmatrix}, \quad -1 = \begin{pmatrix} -1 & 0 \\ 0 & -1 \end{pmatrix} \end{align*} $$
and the field of definition is
![]() $K = \mathbb {Q}(\sqrt {u}, \sqrt {v}, \sqrt {-3})$
.
$K = \mathbb {Q}(\sqrt {u}, \sqrt {v}, \sqrt {-3})$
.
In particular, the biquadratic extension K should include
![]() $\mathbb {Q}(\sqrt {-3})$
. Using these parameters, we can give a concrete defining equation of a twist.
$\mathbb {Q}(\sqrt {-3})$
. Using these parameters, we can give a concrete defining equation of a twist.
Theorem 2.4 [Reference Cardona and Lario2, Propositions 3 and 4].
For a
![]() $G_{\mathbb {Q}}$
-group A embedded in
$G_{\mathbb {Q}}$
-group A embedded in
![]() $\operatorname {\mathrm {GL}}_2(\overline {\mathbb {Q}})$
and isomorphic to
$\operatorname {\mathrm {GL}}_2(\overline {\mathbb {Q}})$
and isomorphic to
![]() $2D_{12}$
as an abstract group, there is a twist with defining equation
$2D_{12}$
as an abstract group, there is a twist with defining equation
whose automorphism group is
![]() $G_{\mathbb {Q}}$
-isomorphic to A. Furthermore, two twists, whose automorphism group is
$G_{\mathbb {Q}}$
-isomorphic to A. Furthermore, two twists, whose automorphism group is
![]() $G_{\mathbb {Q}}$
-isomorphic to A, differ by a hyperelliptic twist.
$G_{\mathbb {Q}}$
-isomorphic to A, differ by a hyperelliptic twist.
Together with a classification of hyperelliptic twists [Reference Cardona and Lario2, Proposition 6], we obtain the complete classification of twists of
![]() $X_0$
, whose automorphism group is defined over K.
$X_0$
, whose automorphism group is defined over K.
Definition 2.5. A twist
![]() $X/k$
of
$X/k$
of
![]() $X_0/k$
is said to be of biquadratic type if the defining field of
$X_0/k$
is said to be of biquadratic type if the defining field of
![]() $\operatorname {\mathrm {Aut}}_{\overline {k}}(X)$
is a biquadratic extension of k.
$\operatorname {\mathrm {Aut}}_{\overline {k}}(X)$
is a biquadratic extension of k.
We note that this terminology works well with [Reference Cardona and Lario2] since the twists of biquadratic type are twists of type
![]() $V_4^\bullet $
for
$V_4^\bullet $
for
![]() $\bullet \in \{ A, B, \ldots , G \}$
in [Reference Cardona and Lario2].
$\bullet \in \{ A, B, \ldots , G \}$
in [Reference Cardona and Lario2].
We also have a complete classification of twists of biquadratic type. The classification is given in two steps. First, we specify the
![]() $G_{\mathbb {Q}}$
-module structure on
$G_{\mathbb {Q}}$
-module structure on
![]() $2D_{12}$
by describing the quadratic subfields (2.1). After that, Theorem 2.4 gives a twist whose automorphism group is isomorphic to a given
$2D_{12}$
by describing the quadratic subfields (2.1). After that, Theorem 2.4 gives a twist whose automorphism group is isomorphic to a given
![]() $G_{\mathbb {Q}}$
-module, and any other twists with the same conditions are the hyperelliptic twists of the given twist. By [Reference Cardona and Lario2, Section 7], we give
$G_{\mathbb {Q}}$
-module, and any other twists with the same conditions are the hyperelliptic twists of the given twist. By [Reference Cardona and Lario2, Section 7], we give
![]() $K_i$
(and hence, a
$K_i$
(and hence, a
![]() $G_{\mathbb {Q}}$
-module structure on
$G_{\mathbb {Q}}$
-module structure on
![]() $2D_{12}$
) for each type
$2D_{12}$
) for each type
![]() $V_4^\bullet $
,
$V_4^\bullet $
,
![]() $\bullet \in \{ A, B, \ldots , G \}$
. Here is a summary of [Reference Cardona and Lario2, Section 7]:
$\bullet \in \{ A, B, \ldots , G \}$
. Here is a summary of [Reference Cardona and Lario2, Section 7]:
-
• if
 $(d, -3) = 1 \in \operatorname {\mathrm {Br}}_2(\mathbb {Q})$
, there is the twist of type
$(d, -3) = 1 \in \operatorname {\mathrm {Br}}_2(\mathbb {Q})$
, there is the twist of type
 $V_4^A$
with
$V_4^A$
with
 $u = d, v=1$
;
$u = d, v=1$
; -
• there is the twist of type
 $V_4^B$
with
$V_4^B$
with
 $u = 1, v=d$
;
$u = 1, v=d$
; -
• there is the twist of type
 $V_4^C$
with
$V_4^C$
with
 $u = d, v=-3$
;
$u = d, v=-3$
; -
• if
 $(d, d) = 1 \in \operatorname {\mathrm {Br}}_2(\mathbb {Q})$
, there is the twist of type
$(d, d) = 1 \in \operatorname {\mathrm {Br}}_2(\mathbb {Q})$
, there is the twist of type
 $V_4^D$
with
$V_4^D$
with
 $u = d, v=-3u$
;
$u = d, v=-3u$
; -
• if
 $(d, -3d) = 1 \in \operatorname {\mathrm {Br}}_2(\mathbb {Q})$
, there is the twist of type
$(d, -3d) = 1 \in \operatorname {\mathrm {Br}}_2(\mathbb {Q})$
, there is the twist of type
 $V_4^E$
with
$V_4^E$
with
 $u = d, v=d$
;
$u = d, v=d$
; -
• there is no twist of the form
 $V_4^F$
;
$V_4^F$
; -
• if
 $(-3d, -3) = 1 \in \operatorname {\mathrm {Br}}_2(\mathbb {Q})$
, there is the twist of type
$(-3d, -3) = 1 \in \operatorname {\mathrm {Br}}_2(\mathbb {Q})$
, there is the twist of type
 $V_4^G$
with
$V_4^G$
with
 $u = -3, v=d$
.
$u = -3, v=d$
.
We recall that
![]() $K_1 = \mathbb {Q}(\sqrt {u}), K_2 = \mathbb {Q}(\sqrt {v})$
and
$K_1 = \mathbb {Q}(\sqrt {u}), K_2 = \mathbb {Q}(\sqrt {v})$
and
![]() $K_3 = \mathbb {Q}(\sqrt {v'})$
.
$K_3 = \mathbb {Q}(\sqrt {v'})$
.
Remark 2.6. We emphasise that the word ‘biquadratic’ in ‘biquadratic type’ is not the same as in the ‘quadratic’ twists of elliptic curves. In the case of elliptic curves, the only twists come from a character of order
![]() $2, 3, 4, 6$
(see [Reference Silverman6, Example X.2.4]). They are usually called quadratic, cubic, quartic and sextic twists of elliptic curves, referring to the minimal field defining
$2, 3, 4, 6$
(see [Reference Silverman6, Example X.2.4]). They are usually called quadratic, cubic, quartic and sextic twists of elliptic curves, referring to the minimal field defining
![]() $\phi : C^{\prime }_{\overline {k}} \to C_{\overline {k}}$
. For example, the map
$\phi : C^{\prime }_{\overline {k}} \to C_{\overline {k}}$
. For example, the map
![]() $ \phi (x, y) := (\kern-1pt\sqrt [3]{A^2}x, Ay) $
that gives a
$ \phi (x, y) := (\kern-1pt\sqrt [3]{A^2}x, Ay) $
that gives a
![]() $\overline {\mathbb {Q}}$
-isomorphism between
$\overline {\mathbb {Q}}$
-isomorphism between
![]() $E_A : y^2 = x^3 + A^2$
and
$E_A : y^2 = x^3 + A^2$
and
![]() $E_1 : y^2 = x^3 + 1$
is defined over
$E_1 : y^2 = x^3 + 1$
is defined over
![]() $\mathbb {Q}(\kern-1.5pt\sqrt [3]{A})$
. This is a cubic extension of
$\mathbb {Q}(\kern-1.5pt\sqrt [3]{A})$
. This is a cubic extension of
![]() $\mathbb {Q}$
when A is a cube-free integer, so it is called a cubic twist.
$\mathbb {Q}$
when A is a cube-free integer, so it is called a cubic twist.
The following definitions come from [Reference Fité and Sutherland5].
Definition 2.7. Let
![]() $X'/k$
be a twist of X and let
$X'/k$
be a twist of X and let
![]() $\phi : X^{\prime }_{\overline {k}} \to X_{\overline {k}}$
be a
$\phi : X^{\prime }_{\overline {k}} \to X_{\overline {k}}$
be a
![]() $\overline {k}$
-isomorphism. We use the following notation:
$\overline {k}$
-isomorphism. We use the following notation:
-
(1)
 $L_\phi $
is the defining field of the isomorphism
$L_\phi $
is the defining field of the isomorphism
 $\phi $
;
$\phi $
; -
(2) K is the defining field of
 $\operatorname {\mathrm {Aut}}_{\overline {k}}(X')$
;
$\operatorname {\mathrm {Aut}}_{\overline {k}}(X')$
; -
(3) L is the compositum of the defining fields of
 $\overline {k}$
-isomorphisms from
$\overline {k}$
-isomorphisms from
 $X'$
to X.
$X'$
to X.
We note that
![]() $K, L$
depend on
$K, L$
depend on
![]() $X'$
and
$X'$
and
![]() $L_\phi $
further depends on the choice of
$L_\phi $
further depends on the choice of
![]() $\phi $
. In the above example concerning
$\phi $
. In the above example concerning
![]() $E_A$
and
$E_A$
and
![]() $E_1$
, there are other choices of
$E_1$
, there are other choices of
![]() $\phi $
making
$\phi $
making
![]() $L_0 = \mathbb {Q}(\zeta _3\!\sqrt [3]{A})$
or
$L_0 = \mathbb {Q}(\zeta _3\!\sqrt [3]{A})$
or
![]() $\mathbb {Q}(\zeta _3^2\sqrt [3]{A})$
. However,
$\mathbb {Q}(\zeta _3^2\sqrt [3]{A})$
. However,
![]() $K = \mathbb {Q}(\zeta _3)$
and
$K = \mathbb {Q}(\zeta _3)$
and
![]() $L = KL_0 = \mathbb {Q}(\zeta _3, \sqrt [3]{A})$
for any choice of
$L = KL_0 = \mathbb {Q}(\zeta _3, \sqrt [3]{A})$
for any choice of
![]() $\phi $
. Hence a quadratic, cubic, quartic and sextic twist on an elliptic curve indicates the degree of
$\phi $
. Hence a quadratic, cubic, quartic and sextic twist on an elliptic curve indicates the degree of
![]() $L_{\phi }$
, and the word ‘biquadratic’ in the phrase ‘twists of biquadratic type’ means the extension of
$L_{\phi }$
, and the word ‘biquadratic’ in the phrase ‘twists of biquadratic type’ means the extension of
![]() $K/\mathbb {Q}$
.
$K/\mathbb {Q}$
.
Lemma 2.8 [Reference Fité and Sutherland5, Lemmas 4.2 and 4.3 and Proposition 4.6].
The following statements hold for a twist X of
![]() $X_0$
.
$X_0$
.
-
(1) K is the defining field of endomorphisms of
 $\operatorname {\mathrm {Jac}}(X_{\overline {\mathbb {Q}}})$
.
$\operatorname {\mathrm {Jac}}(X_{\overline {\mathbb {Q}}})$
. -
(2) The following extensions of K coincide:
-
(a) L as in Definition 2.7;
-
(b) the compositum of K and
 $L_{\phi }$
for any
$L_{\phi }$
for any
 $\overline {\mathbb {Q}}$
-isomorphism
$\overline {\mathbb {Q}}$
-isomorphism
 $\phi : X \to X_0$
;
$\phi : X \to X_0$
; -
(c) the defining field of all homomorphisms from
 $\operatorname {\mathrm {Jac}}(X_0)_{\overline {\mathbb {Q}}}$
to
$\operatorname {\mathrm {Jac}}(X_0)_{\overline {\mathbb {Q}}}$
to
 $\operatorname {\mathrm {Jac}}(X)_{\overline {\mathbb {Q}}}$
.
$\operatorname {\mathrm {Jac}}(X)_{\overline {\mathbb {Q}}}$
.
-
-
(3)
 $[L : K] \leq 2$
.
$[L : K] \leq 2$
.
3. Twist families of biquadratic type
In this section, we give a concrete parametrisation of twist types
![]() $V_4^B$
and
$V_4^B$
and
![]() $V_4^C$
. Then we prove that L is a subfield of
$V_4^C$
. Then we prove that L is a subfield of
![]() $K(i)$
in both cases.
$K(i)$
in both cases.
3.1. Type C
For
![]() $V_4^C$
, we have
$V_4^C$
, we have
![]() $u = d$
,
$u = d$
,
![]() $v = -3$
. According to Definition 2.2,
$v = -3$
. According to Definition 2.2,
![]() $ x^2-y^2=d$
has solutions in
$ x^2-y^2=d$
has solutions in
![]() $\mathbb {Q}^\times $
. We choose (with
$\mathbb {Q}^\times $
. We choose (with
![]() $d\neq \pm 1$
)
$d\neq \pm 1$
)
so that the defining equation in Theorem 2.4 becomes
![]() $y^2 = f_d(x)$
, where
$y^2 = f_d(x)$
, where
 $$ \begin{align} f_d(x) =54(d^3 + 1) x^6 & + 324( d^3 - 1) x^5 + 810(d^3 + 1) x^4 + 1080(d^3 - 1) x^3 \notag \\ &+ 810(d^3 + 1) x^2 + 324(d^3 - 1) x + 54( d^3 + 1). \end{align} $$
$$ \begin{align} f_d(x) =54(d^3 + 1) x^6 & + 324( d^3 - 1) x^5 + 810(d^3 + 1) x^4 + 1080(d^3 - 1) x^3 \notag \\ &+ 810(d^3 + 1) x^2 + 324(d^3 - 1) x + 54( d^3 + 1). \end{align} $$
Let
![]() $X_d^C$
be the twist defined by the equation
$X_d^C$
be the twist defined by the equation
![]() $y^2 = f_d(x)$
. Then, the field K of
$y^2 = f_d(x)$
. Then, the field K of
![]() $X_d^C$
is
$X_d^C$
is
![]() $\mathbb {Q}(\sqrt {d}, \sqrt {-3})$
since
$\mathbb {Q}(\sqrt {d}, \sqrt {-3})$
since
![]() $u = d$
and
$u = d$
and
![]() $v = -3$
. Also, by Theorem 2.4,
$v = -3$
. Also, by Theorem 2.4,
![]() $\{X_d^C\}$
is the one-parameter twist such that:
$\{X_d^C\}$
is the one-parameter twist such that:
-
• none of the elements is a hyperelliptic twist of another element;
-
• every twist of type
 $V_4^C$
is a hyperelliptic twist of
$V_4^C$
is a hyperelliptic twist of
 $\{X_d^C\}$
.
$\{X_d^C\}$
.
Hence, one can say that this family is a family of twists orthogonal to the hyperelliptic twists. This is the first motivation of our paper.
The polynomial
![]() $f_d(x)$
in (3.1) factors into
$f_d(x)$
in (3.1) factors into
 $$ \begin{align*} &54( (d+1)x^2 + 2(d-1)x + d + 1) \\ &\quad \times ((d^2-d+1) x^4 + 4 (d^2 - 1) x^3 + (6 d^2 + 2 d + 6) x^2 + 4 (d^2 - 1) x + d^2 - d + 1). \end{align*} $$
$$ \begin{align*} &54( (d+1)x^2 + 2(d-1)x + d + 1) \\ &\quad \times ((d^2-d+1) x^4 + 4 (d^2 - 1) x^3 + (6 d^2 + 2 d + 6) x^2 + 4 (d^2 - 1) x + d^2 - d + 1). \end{align*} $$
We further suppose that
![]() $d \neq 0$
and set
$d \neq 0$
and set
![]() $\delta _C = (1+\sqrt {-3})/2d$
. Then the zeros are
$\delta _C = (1+\sqrt {-3})/2d$
. Then the zeros are
 $$ \begin{align*} \frac{-(d-1) \pm 2\sqrt{-d}}{d+1}, \quad -\frac{1-\sqrt{\delta_C}}{1+\sqrt{\delta_C}}, \quad -\frac{1+\sqrt{\delta_C}}{1-\sqrt{\delta_C}}, \quad -\frac{1-\sqrt{\overline{\delta_C}}}{1+\sqrt{\overline{\delta_C}}}, \quad -\frac{1+\sqrt{\overline{\delta_C}}}{1-\sqrt{\overline{\delta_C}}}. \end{align*} $$
$$ \begin{align*} \frac{-(d-1) \pm 2\sqrt{-d}}{d+1}, \quad -\frac{1-\sqrt{\delta_C}}{1+\sqrt{\delta_C}}, \quad -\frac{1+\sqrt{\delta_C}}{1-\sqrt{\delta_C}}, \quad -\frac{1-\sqrt{\overline{\delta_C}}}{1+\sqrt{\overline{\delta_C}}}, \quad -\frac{1+\sqrt{\overline{\delta_C}}}{1-\sqrt{\overline{\delta_C}}}. \end{align*} $$
Lemma 3.1. Let d be a nonsquare rational number and let
![]() $L_d$
be the splitting field of the quartic factor of
$L_d$
be the splitting field of the quartic factor of
![]() $f_d(x)$
, that is,
$f_d(x)$
, that is,
Then,
![]() $L_d$
is a biquadratic extension of
$L_d$
is a biquadratic extension of
![]() $\mathbb {Q}$
satisfying
$\mathbb {Q}$
satisfying
![]() $L_d \subset K(i)$
when
$L_d \subset K(i)$
when
![]() $d \neq \pm 1, \pm 3$
.
$d \neq \pm 1, \pm 3$
.
Proof. One can easily compute that
and
 $$ \begin{align*} \frac{\displaystyle\frac{1 - \sqrt{\delta_C}}{1 + \sqrt{\delta_C}} -1 }{\displaystyle\frac{1 - \sqrt{\delta_C}}{1 + \sqrt{\delta_C}} +1} = -\sqrt{\delta_C}. \end{align*} $$
$$ \begin{align*} \frac{\displaystyle\frac{1 - \sqrt{\delta_C}}{1 + \sqrt{\delta_C}} -1 }{\displaystyle\frac{1 - \sqrt{\delta_C}}{1 + \sqrt{\delta_C}} +1} = -\sqrt{\delta_C}. \end{align*} $$
Hence,
 $$ \begin{align*} L_d = \mathbb{Q} \bigg({\frac{1-\sqrt{\delta_C}}{1+\sqrt{\delta_C}}, \frac{1-\sqrt{\overline{\delta_C}}}{1+\sqrt{\overline{\delta_C}}}}\bigg) = \mathbb{Q} \bigg(\frac{1-\sqrt{\delta_C}}{1+\sqrt{\delta_C}}\bigg) = \mathbb{Q}(\sqrt{\delta_C}). \end{align*} $$
$$ \begin{align*} L_d = \mathbb{Q} \bigg({\frac{1-\sqrt{\delta_C}}{1+\sqrt{\delta_C}}, \frac{1-\sqrt{\overline{\delta_C}}}{1+\sqrt{\overline{\delta_C}}}}\bigg) = \mathbb{Q} \bigg(\frac{1-\sqrt{\delta_C}}{1+\sqrt{\delta_C}}\bigg) = \mathbb{Q}(\sqrt{\delta_C}). \end{align*} $$
We note that each root of the quartic part of
![]() $f_d$
is not in
$f_d$
is not in
![]() $\mathbb {Q}$
because
$\mathbb {Q}$
because
 $$ \begin{align*} \frac{1-\sqrt{\delta_C}}{1 + \sqrt{\delta_C}} = -1 + \frac{2}{1 + \sqrt{\delta_C}} \not\in \mathbb{Q}, \quad \sqrt{\delta_C} \not\in \mathbb{Q}, \quad \delta_C \not\in\mathbb{Q}. \end{align*} $$
$$ \begin{align*} \frac{1-\sqrt{\delta_C}}{1 + \sqrt{\delta_C}} = -1 + \frac{2}{1 + \sqrt{\delta_C}} \not\in \mathbb{Q}, \quad \sqrt{\delta_C} \not\in \mathbb{Q}, \quad \delta_C \not\in\mathbb{Q}. \end{align*} $$
Hence, if the quartic part is reducible, then it is a product of two quadratics over
![]() $\mathbb {Q}$
. So in this case,
$\mathbb {Q}$
. So in this case,
![]() $L_d \supset \mathbb {Q}(\sqrt {-3})$
is a biquadratic extension of
$L_d \supset \mathbb {Q}(\sqrt {-3})$
is a biquadratic extension of
![]() $\mathbb {Q}$
.
$\mathbb {Q}$
.
Otherwise, the discriminant of the quartic is
![]() ${2^{16}\cdot 3^2 \cdot d^6}/{(d^2-d+1)^6}$
and the resolvent of the quartic is
${2^{16}\cdot 3^2 \cdot d^6}/{(d^2-d+1)^6}$
and the resolvent of the quartic is
 $$ \begin{align*} &x^3 + \frac{-6d^2 - 2d - 6}{d^2 - d + 1} \cdot x^2 + \frac{12d^4 + 8d^3 - 44d^2 + 8d + 12}{d^4 - 2d^3 + 3d^2 - 2d + 1} \cdot x \\ &\qquad+ \frac{-8d^4 - 16d^3 + 104d^2 - 16d - 8}{d^4 - 2d^3 + 3d^2 - 2d + 1}\\ &\quad=\bigg(x + \frac{-2d^2 - 10d - 2}{d^2 - d + 1}\bigg) \cdot (x - 2) \cdot \bigg(x + \frac{-2d^2 + 6d - 2}{d^2 - d + 1}\bigg). \end{align*} $$
$$ \begin{align*} &x^3 + \frac{-6d^2 - 2d - 6}{d^2 - d + 1} \cdot x^2 + \frac{12d^4 + 8d^3 - 44d^2 + 8d + 12}{d^4 - 2d^3 + 3d^2 - 2d + 1} \cdot x \\ &\qquad+ \frac{-8d^4 - 16d^3 + 104d^2 - 16d - 8}{d^4 - 2d^3 + 3d^2 - 2d + 1}\\ &\quad=\bigg(x + \frac{-2d^2 - 10d - 2}{d^2 - d + 1}\bigg) \cdot (x - 2) \cdot \bigg(x + \frac{-2d^2 + 6d - 2}{d^2 - d + 1}\bigg). \end{align*} $$
Hence,
![]() $L_d$
is again a biquadratic extension of
$L_d$
is again a biquadratic extension of
![]() $\mathbb {Q}$
, since the discriminant is square and the resolvent is reducible.
$\mathbb {Q}$
, since the discriminant is square and the resolvent is reducible.
We can say that
![]() $L_d = \mathbb {Q}(\sqrt {-3}, \sqrt {t})$
for some
$L_d = \mathbb {Q}(\sqrt {-3}, \sqrt {t})$
for some
![]() $t \in \mathbb {Q}^\times /(\mathbb {Q}^\times )^2$
since
$t \in \mathbb {Q}^\times /(\mathbb {Q}^\times )^2$
since
![]() $\sqrt {-3} \in L_d$
. Then,
$\sqrt {-3} \in L_d$
. Then,
![]() $K(\sqrt {\delta _C}) = \mathbb {Q}(\sqrt {-3}, \sqrt {d}, \sqrt {t})$
should be a triquadratic extension containing
$K(\sqrt {\delta _C}) = \mathbb {Q}(\sqrt {-3}, \sqrt {d}, \sqrt {t})$
should be a triquadratic extension containing
![]() $\mathbb {Q}(\sqrt {\delta _C})$
or
$\mathbb {Q}(\sqrt {\delta _C})$
or
![]() $\mathbb {Q}(\sqrt {\delta _C})$
itself. However,
$\mathbb {Q}(\sqrt {\delta _C})$
itself. However,
 $$ \begin{align*} \sqrt{d}\sqrt{\delta_C} = \sqrt{ \frac{1 + \sqrt{-3}}{2} } = \frac{i}{\frac{1+\sqrt{-3}}{2}} \in K(\sqrt{\delta_C}), \end{align*} $$
$$ \begin{align*} \sqrt{d}\sqrt{\delta_C} = \sqrt{ \frac{1 + \sqrt{-3}}{2} } = \frac{i}{\frac{1+\sqrt{-3}}{2}} \in K(\sqrt{\delta_C}), \end{align*} $$
which implies that
![]() $i \in K(\sqrt {\delta _C})$
. Hence, when
$i \in K(\sqrt {\delta _C})$
. Hence, when
![]() $d \neq \pm 3$
,
$d \neq \pm 3$
,
![]() $K(\sqrt {\delta _C}) = \mathbb {Q}(\sqrt {-3}, i, \sqrt {d} )$
and
$K(\sqrt {\delta _C}) = \mathbb {Q}(\sqrt {-3}, i, \sqrt {d} )$
and
![]() $\mathbb {Q}(\sqrt {\delta _C}) \subset K(i)$
.
$\mathbb {Q}(\sqrt {\delta _C}) \subset K(i)$
.
3.2. Type B
For
![]() $V_4^B$
, we have
$V_4^B$
, we have
![]() $u=1$
and
$u=1$
and
![]() $v=d$
. According to Definition 2.2,
$v=d$
. According to Definition 2.2,
![]() $x^2+({3}/{d})y^2=1$
has solutions in
$x^2+({3}/{d})y^2=1$
has solutions in
![]() $\mathbb {Q}^\times $
. We choose (with
$\mathbb {Q}^\times $
. We choose (with
![]() $d\neq \pm 3$
)
$d\neq \pm 3$
)
so that the defining equation in Theorem 2.4 becomes of the form
![]() $y^2 = f_d(x)$
, where
$y^2 = f_d(x)$
, where
 $$ \begin{align*} f_d(x) &= 27 \frac{(d - 3)(d^2 - 42d + 9)}{(d + 3)^3} \bigg( x^2 + \frac{{4d}}{{d - 3}}x - \frac{{1}}{{3}}d \bigg) \\ &\quad \times \bigg( x^4 + \frac{{32d^2 - 96d}}{{d^2 - 42d + 9}}x^3 + \frac{{-\frac{{14}}{{3}}d^3 + 68d^2 - 42d}}{{d^2 - 42d + 9}}x^2 + \frac{{-\frac{{32}}{{3}}d^3 + 32d^2}}{{d^2 - 42d + 9}}x + \frac{{1}}{{9}}d^2 \bigg). \end{align*} $$
$$ \begin{align*} f_d(x) &= 27 \frac{(d - 3)(d^2 - 42d + 9)}{(d + 3)^3} \bigg( x^2 + \frac{{4d}}{{d - 3}}x - \frac{{1}}{{3}}d \bigg) \\ &\quad \times \bigg( x^4 + \frac{{32d^2 - 96d}}{{d^2 - 42d + 9}}x^3 + \frac{{-\frac{{14}}{{3}}d^3 + 68d^2 - 42d}}{{d^2 - 42d + 9}}x^2 + \frac{{-\frac{{32}}{{3}}d^3 + 32d^2}}{{d^2 - 42d + 9}}x + \frac{{1}}{{9}}d^2 \bigg). \end{align*} $$
As before, we denote by
![]() $X_d^B$
the twist
$X_d^B$
the twist
![]() $y^2 = f_d(x)$
. Then the family
$y^2 = f_d(x)$
. Then the family
![]() $\{X_d^B\}$
has the same properties as the family
$\{X_d^B\}$
has the same properties as the family
![]() $\{X_d^C\}$
discussed in the previous section.
$\{X_d^C\}$
discussed in the previous section.
The zeros of the quadratic factors of
![]() $f_d$
are
$f_d$
are
 $$ \begin{align*} \frac{-6d \pm (d+3)\sqrt{3d}}{3(d-3)}, \end{align*} $$
$$ \begin{align*} \frac{-6d \pm (d+3)\sqrt{3d}}{3(d-3)}, \end{align*} $$
and the zeros of the quartic factor are
 $$ \begin{align*} -\frac{1}{2}\Bigg({\delta_B \pm \sqrt{\delta_B^2 + \frac{4d}{3}} }\Bigg), \quad -\frac{1}{2}\Bigg({\overline{\delta_B} \pm \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}} }\Bigg), \end{align*} $$
$$ \begin{align*} -\frac{1}{2}\Bigg({\delta_B \pm \sqrt{\delta_B^2 + \frac{4d}{3}} }\Bigg), \quad -\frac{1}{2}\Bigg({\overline{\delta_B} \pm \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}} }\Bigg), \end{align*} $$
where
 $$ \begin{align*} \delta_B := \frac{-16d^2 + 48d + 2(d+3)^2 \sqrt{d}}{d^2-42d+9}, \quad \overline{\delta_B} := \frac{-16d^2 + 48d - 2(d+3)^2 \sqrt{d}}{d^2-42d+9}. \end{align*} $$
$$ \begin{align*} \delta_B := \frac{-16d^2 + 48d + 2(d+3)^2 \sqrt{d}}{d^2-42d+9}, \quad \overline{\delta_B} := \frac{-16d^2 + 48d - 2(d+3)^2 \sqrt{d}}{d^2-42d+9}. \end{align*} $$
The defining fields of zeros of the quadratic and the quartic are
 $$ \begin{align*} \mathbb{Q}(\sqrt{3d}), \quad \mathbb{Q}\Bigg(\sqrt{d}, \sqrt{\delta_B^2 + \frac{4d}{3}}, \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}}\Bigg). \end{align*} $$
$$ \begin{align*} \mathbb{Q}(\sqrt{3d}), \quad \mathbb{Q}\Bigg(\sqrt{d}, \sqrt{\delta_B^2 + \frac{4d}{3}}, \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}}\Bigg). \end{align*} $$
The latter is denoted by
![]() $L_d$
, which is the splitting field of the quartic part of
$L_d$
, which is the splitting field of the quartic part of
![]() $f_d$
.
$f_d$
.
Lemma 3.2. In the above settings,
![]() $L_d = \mathbb {Q}(\sqrt {d}, \sqrt {3})$
when
$L_d = \mathbb {Q}(\sqrt {d}, \sqrt {3})$
when
![]() $d\neq \pm 3$
is a nonsquare rational number.
$d\neq \pm 3$
is a nonsquare rational number.
Proof. The quartic part of
![]() $f_d$
is
$f_d$
is
 $$ \begin{align*} &x^4 + \frac{32d^2 - 96d}{d^2 - 42d + 9} x^3 + \frac{-\frac{14}{3}d^3 + 68d^2 - 42d}{d^2 - 42d + 9} x^2 + \frac{-\frac{32}{3}d^3 + 32d^2}{d^2 - 42d + 9} x + \frac{1}{9}d^2. \end{align*} $$
$$ \begin{align*} &x^4 + \frac{32d^2 - 96d}{d^2 - 42d + 9} x^3 + \frac{-\frac{14}{3}d^3 + 68d^2 - 42d}{d^2 - 42d + 9} x^2 + \frac{-\frac{32}{3}d^3 + 32d^2}{d^2 - 42d + 9} x + \frac{1}{9}d^2. \end{align*} $$
Since
 $$ \begin{align*} \sqrt{\delta_B^2 + \frac{4d}{3}} \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}} = \frac{16}{3} \frac{d(d+3)^2}{(d^2 - 42 d + 9)} \in \mathbb{Q}, \end{align*} $$
$$ \begin{align*} \sqrt{\delta_B^2 + \frac{4d}{3}} \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}} = \frac{16}{3} \frac{d(d+3)^2}{(d^2 - 42 d + 9)} \in \mathbb{Q}, \end{align*} $$
we have
 $$ \begin{align*} L_d = \mathbb{Q}\Bigg(\sqrt{d}, \sqrt{\delta_B^2 + \frac{4d}{3}}, \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}}\Bigg) = \mathbb{Q}\Bigg({\sqrt{\delta_B^2 + \frac{4d}{3}}}\Bigg). \end{align*} $$
$$ \begin{align*} L_d = \mathbb{Q}\Bigg(\sqrt{d}, \sqrt{\delta_B^2 + \frac{4d}{3}}, \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}}\Bigg) = \mathbb{Q}\Bigg({\sqrt{\delta_B^2 + \frac{4d}{3}}}\Bigg). \end{align*} $$
However,
 $$ \begin{align*} \Bigg({\sqrt{\delta_B^2 + \frac{4d}{3}} + \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}}}\Bigg)^2 &= \delta_B^2 + \overline{\delta_B}^2 +\frac{8d}{3} + \sqrt{\delta_B^2 + \frac{4d}{3}} \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}} \in \mathbb{Q}, \end{align*} $$
$$ \begin{align*} \Bigg({\sqrt{\delta_B^2 + \frac{4d}{3}} + \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}}}\Bigg)^2 &= \delta_B^2 + \overline{\delta_B}^2 +\frac{8d}{3} + \sqrt{\delta_B^2 + \frac{4d}{3}} \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}} \in \mathbb{Q}, \end{align*} $$
which means that
 $$ \begin{align*} \mathbb{Q}\Bigg({\sqrt{\delta_B^2 + \frac{4d}{3}} + \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}}}\Bigg) \subset \mathbb{Q} \Bigg({\sqrt{\delta_B^2 + \frac{4d}{3}} }\Bigg) \end{align*} $$
$$ \begin{align*} \mathbb{Q}\Bigg({\sqrt{\delta_B^2 + \frac{4d}{3}} + \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}}}\Bigg) \subset \mathbb{Q} \Bigg({\sqrt{\delta_B^2 + \frac{4d}{3}} }\Bigg) \end{align*} $$
is a quadratic subfield. Since
 $$ \begin{align*} \Bigg({\sqrt{\delta_B^2 + \frac{4d}{3}} + \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}}}\Bigg)^2 = \Bigg({\frac{8}{3} \frac{(d-3)(d+3)}{d^2 - 42d + 9}\sqrt{3d}}\Bigg)^2, \end{align*} $$
$$ \begin{align*} \Bigg({\sqrt{\delta_B^2 + \frac{4d}{3}} + \sqrt{\overline{\delta_B}^2 + \frac{4d}{3}}}\Bigg)^2 = \Bigg({\frac{8}{3} \frac{(d-3)(d+3)}{d^2 - 42d + 9}\sqrt{3d}}\Bigg)^2, \end{align*} $$
we can conclude that
![]() $L_d \supset \mathbb {Q}(\sqrt {d}, \sqrt {3})$
.
$L_d \supset \mathbb {Q}(\sqrt {d}, \sqrt {3})$
.
Next, we claim that each zero of the quartic is not rational. Suppose that
 $$ \begin{align*} \delta_B\pm\sqrt{\delta_B^2+\frac{4d}{3}}\in\mathbb{Q}. \end{align*} $$
$$ \begin{align*} \delta_B\pm\sqrt{\delta_B^2+\frac{4d}{3}}\in\mathbb{Q}. \end{align*} $$
Then there is a rational number a such that
 $$ \begin{align*} \sqrt{\delta_B^2+\frac{4d}{3}}=a\mp\frac{2(d+3)^2}{d^2-42d+9}\sqrt{d}. \end{align*} $$
$$ \begin{align*} \sqrt{\delta_B^2+\frac{4d}{3}}=a\mp\frac{2(d+3)^2}{d^2-42d+9}\sqrt{d}. \end{align*} $$
Taking the squares of both sides,
 $$ \begin{align*} \frac{16d(d+3)^2(d^2+30d+9)}{3(d^2-42d+9)^2} & \mp\frac{64(d-3)d(d+3)^2}{(d^2-42d+9)^2}\sqrt{d} \\ =a^2+\frac{4d(d+3)^4}{(d^2-42d+9)^2} & \mp\frac{4a(d+3)^2}{(d^2-42d+9)}\sqrt{d}. \end{align*} $$
$$ \begin{align*} \frac{16d(d+3)^2(d^2+30d+9)}{3(d^2-42d+9)^2} & \mp\frac{64(d-3)d(d+3)^2}{(d^2-42d+9)^2}\sqrt{d} \\ =a^2+\frac{4d(d+3)^4}{(d^2-42d+9)^2} & \mp\frac{4a(d+3)^2}{(d^2-42d+9)}\sqrt{d}. \end{align*} $$
Comparing the coefficients of
![]() $\sqrt {d}$
,
$\sqrt {d}$
,
Substituting this yields
 $$ \begin{align*} \frac{16d(d+3)^2(d^2+30d+9)}{3(d^2-42d+9)^2}=\frac{16^2d^2(d-3)^2}{(d^2-42d+9)^2}+\frac{4d(d+3)^4}{(d^2-42d+9)^2}. \end{align*} $$
$$ \begin{align*} \frac{16d(d+3)^2(d^2+30d+9)}{3(d^2-42d+9)^2}=\frac{16^2d^2(d-3)^2}{(d^2-42d+9)^2}+\frac{4d(d+3)^4}{(d^2-42d+9)^2}. \end{align*} $$
This is equivalent to
which does not have a solution in
![]() $\mathbb {Q}$
. To deal with the other case:
$\mathbb {Q}$
. To deal with the other case:
 $$ \begin{align*} \overline{\delta}_B\pm\sqrt{\overline{\delta}_B^2+\frac{4d}{3}}\in\mathbb{Q}, \end{align*} $$
$$ \begin{align*} \overline{\delta}_B\pm\sqrt{\overline{\delta}_B^2+\frac{4d}{3}}\in\mathbb{Q}, \end{align*} $$
it suffices to replace
![]() $\sqrt {d}$
by
$\sqrt {d}$
by
![]() $-\sqrt {d}$
in the above computation. Therefore, we arrive at the same conclusion. Hence, if the quartic part of f is reducible over
$-\sqrt {d}$
in the above computation. Therefore, we arrive at the same conclusion. Hence, if the quartic part of f is reducible over
![]() $\mathbb {Q}$
, it is a product of two quadratics, which means that
$\mathbb {Q}$
, it is a product of two quadratics, which means that
![]() $L_d$
is a biquadratic extension of
$L_d$
is a biquadratic extension of
![]() $\mathbb {Q}$
.
$\mathbb {Q}$
.
Suppose that the quartic part is irreducible over
![]() $\mathbb {Q}$
. Its discriminant is
$\mathbb {Q}$
. Its discriminant is
 $$ \begin{align*} \frac{2^{16}}{3^4} \frac{d^6 (d + 3)^{12}}{(d^2 - 42d + 9)^{6}}, \end{align*} $$
$$ \begin{align*} \frac{2^{16}}{3^4} \frac{d^6 (d + 3)^{12}}{(d^2 - 42d + 9)^{6}}, \end{align*} $$
which is a square, and its resolvent is
 $$ \begin{align*} \bigg(x + \frac{\frac{{-2}}{{3}}d^3 - 36d^2 - 6d}{d^2 - 42d + 9} \bigg) \cdot \bigg(x + \frac{{2}}{{3}}d \bigg) \cdot \bigg(x + \frac{\frac{{14}}{{3}}d^3 - 4d^2 + 42d }{d^2 - 42d + 9}\bigg), \end{align*} $$
$$ \begin{align*} \bigg(x + \frac{\frac{{-2}}{{3}}d^3 - 36d^2 - 6d}{d^2 - 42d + 9} \bigg) \cdot \bigg(x + \frac{{2}}{{3}}d \bigg) \cdot \bigg(x + \frac{\frac{{14}}{{3}}d^3 - 4d^2 + 42d }{d^2 - 42d + 9}\bigg), \end{align*} $$
which is reducible. Hence,
![]() $L_d$
is also a biquadratic extension of
$L_d$
is also a biquadratic extension of
![]() $\mathbb {Q}$
. Consequently, we have
$\mathbb {Q}$
. Consequently, we have
![]() $L_d = \mathbb {Q}(\sqrt {d}, \sqrt {3})$
in both cases.
$L_d = \mathbb {Q}(\sqrt {d}, \sqrt {3})$
in both cases.
3.3. Computation of the number field L
Proposition 3.3. Let
![]() $X_d : y^2 = f_d(x)$
be a twist of type
$X_d : y^2 = f_d(x)$
be a twist of type
![]() $V_4^{\bullet }$
with
$V_4^{\bullet }$
with
![]() $\bullet \in \{ A, B, \ldots , G \}$
and
$\bullet \in \{ A, B, \ldots , G \}$
and
![]() $K = \mathbb {Q}(\sqrt {d}, \sqrt {-3})$
. Suppose that
$K = \mathbb {Q}(\sqrt {d}, \sqrt {-3})$
. Suppose that
![]() $f_d(x)$
is decomposed in
$f_d(x)$
is decomposed in
![]() $K(i)$
. Then, L is
$K(i)$
. Then, L is
![]() $K(i)$
or K.
$K(i)$
or K.
Proof. By Lemma 2.1, every isomorphism from
![]() $X_0$
to
$X_0$
to
![]() $X_d$
can be represented by a linear fractional transformation on x. More precisely, an isomorphism is given by
$X_d$
can be represented by a linear fractional transformation on x. More precisely, an isomorphism is given by
 $$ \begin{align*} x \to \frac{mx + n}{px + q}, \quad y \to \frac{(mq-pn)y}{(px+q)^3} \end{align*} $$
$$ \begin{align*} x \to \frac{mx + n}{px + q}, \quad y \to \frac{(mq-pn)y}{(px+q)^3} \end{align*} $$
with
![]() $mq-pn \neq 0$
. Let
$mq-pn \neq 0$
. Let
![]() $\gamma _i$
denote the zeros of
$\gamma _i$
denote the zeros of
![]() $f_d$
. Since the zeros of
$f_d$
. Since the zeros of
![]() $x^6+1$
are
$x^6+1$
are
![]() $\pm i, \pm i\zeta _3, \pm i\zeta _3^2$
, at least one of the linear fractional transformations that satisfy
$\pm i, \pm i\zeta _3, \pm i\zeta _3^2$
, at least one of the linear fractional transformations that satisfy
for
![]() $k_i \in \{ 1, \ldots , 6 \}$
is the isomorphism from
$k_i \in \{ 1, \ldots , 6 \}$
is the isomorphism from
![]() $C_1$
to
$C_1$
to
![]() $C_d$
, which is defined over (possibly a subfield of)
$C_d$
, which is defined over (possibly a subfield of)
![]() $\mathbb {Q}(i, \zeta _3, \gamma _1, \ldots , \gamma _6)$
.
$\mathbb {Q}(i, \zeta _3, \gamma _1, \ldots , \gamma _6)$
.
Let
![]() $L_{\phi }$
be the defining field of this isomorphism so that
$L_{\phi }$
be the defining field of this isomorphism so that
![]() $L_{\phi } \subset \mathbb {Q}(i, \zeta _3, \gamma _1, \ldots , \gamma _6)$
. By assumption,
$L_{\phi } \subset \mathbb {Q}(i, \zeta _3, \gamma _1, \ldots , \gamma _6)$
. By assumption,
![]() $\mathbb {Q}(\gamma _1, \ldots , \gamma _6)$
and also
$\mathbb {Q}(\gamma _1, \ldots , \gamma _6)$
and also
![]() $\mathbb {Q}(i, \zeta _3, \gamma _1, \ldots , \gamma _6)$
are subfields of
$\mathbb {Q}(i, \zeta _3, \gamma _1, \ldots , \gamma _6)$
are subfields of
![]() $K(i)$
. Therefore,
$K(i)$
. Therefore,
 $$ \begin{align*} L = \left\{ \begin{array}{@{}ll} K & \textrm{if } L_{\phi} \subset K, \\ K(i) & \textrm{otherwise}, \end{array} \right. \end{align*} $$
$$ \begin{align*} L = \left\{ \begin{array}{@{}ll} K & \textrm{if } L_{\phi} \subset K, \\ K(i) & \textrm{otherwise}, \end{array} \right. \end{align*} $$
since
![]() $L_{\phi }K = L$
by Lemma 2.8.
$L_{\phi }K = L$
by Lemma 2.8.
Corollary 3.4. Let
![]() $X_d$
be a twist of type
$X_d$
be a twist of type
![]() $V_4^B$
or
$V_4^B$
or
![]() $V_4^C$
. Then, L is
$V_4^C$
. Then, L is
![]() $K(i)$
or K.
$K(i)$
or K.
4. Proof of the main theorem
4.1. Proof for type
 $B, C$
$B, C$
Let E be an elliptic curve. Then, its L-function is a product of L-factors,
![]() $L_p(E/\mathbb {Q}, s) = (1 - a_p(E) p^{-s} + p^{1 - 2s})^{-1}$
, where E has good reduction at p. Here,
$L_p(E/\mathbb {Q}, s) = (1 - a_p(E) p^{-s} + p^{1 - 2s})^{-1}$
, where E has good reduction at p. Here,
![]() $a_p(E)$
is the trace of the Frobenius which is in the interval
$a_p(E)$
is the trace of the Frobenius which is in the interval
![]() $[-2\sqrt {p}, 2\sqrt {p}]$
. Similarly,
$[-2\sqrt {p}, 2\sqrt {p}]$
. Similarly,
when X is a curve of genus
![]() $2$
and has good reduction at p. For an elliptic curve E and a genus
$2$
and has good reduction at p. For an elliptic curve E and a genus
![]() $2$
curve X, we denote by
$2$
curve X, we denote by
![]() $E^{(D)}$
and
$E^{(D)}$
and
![]() $X^{(D)}$
the respective hyperelliptic twists given by the field
$X^{(D)}$
the respective hyperelliptic twists given by the field
![]() $\mathbb {Q}(\sqrt {D})$
. It is also well known that
$\mathbb {Q}(\sqrt {D})$
. It is also well known that
where
![]() $\chi _D$
is the quadratic character attached to the field extension
$\chi _D$
is the quadratic character attached to the field extension
![]() $\mathbb {Q}(\sqrt {D})/\mathbb {Q}$
.
$\mathbb {Q}(\sqrt {D})/\mathbb {Q}$
.
Table 1 The L-factors of
![]() $J_d$
corresponding to
$J_d$
corresponding to
![]() $I(p)$
.
$I(p)$
.

Let
![]() $X_d^B$
and
$X_d^B$
and
![]() $X_d^C$
be the twists of
$X_d^C$
be the twists of
![]() $X_0$
of biquadratic type
$X_0$
of biquadratic type
![]() $B, C$
studied in the previous section. We denote by
$B, C$
studied in the previous section. We denote by
![]() $J_0, J_d^B, J_d^C$
their Jacobians, by
$J_0, J_d^B, J_d^C$
their Jacobians, by
![]() $X_0^{(D)}, X_d^{B, (D)}, X_d^{C, (D)}$
the hyperelliptic twists and by
$X_0^{(D)}, X_d^{B, (D)}, X_d^{C, (D)}$
the hyperelliptic twists and by
![]() $J_0^{(D)}, J_d^{B, (D)}, J_d^{C, (D)}$
the Jacobians of the hyperelliptic twists. Finally, we denote by
$J_0^{(D)}, J_d^{B, (D)}, J_d^{C, (D)}$
the Jacobians of the hyperelliptic twists. Finally, we denote by
![]() $E_0$
the elliptic curve over
$E_0$
the elliptic curve over
![]() $\mathbb {Q}$
defined by the equation
$\mathbb {Q}$
defined by the equation
![]() $y^2 = x^3 + 1$
.
$y^2 = x^3 + 1$
.
We also recall some notation from [Reference Fité and Sutherland5, Section 4]. Let
![]() $M = \mathbb {Q}(\sqrt {-3})$
. The definition of
$M = \mathbb {Q}(\sqrt {-3})$
. The definition of
![]() $L, K$
which depends on the choice of the twist X of
$L, K$
which depends on the choice of the twist X of
![]() $X_0$
is given by Definition 2.7. For a number field F, the residue degree at p in
$X_0$
is given by Definition 2.7. For a number field F, the residue degree at p in
![]() $F/\mathbb {Q}$
is denoted by
$F/\mathbb {Q}$
is denoted by
![]() $f_F(p)$
. We define
$f_F(p)$
. We define
![]() $ I(p) = I(p, C) := (f_L(p), f_K(p), f_M(p))$
.
$ I(p) = I(p, C) := (f_L(p), f_K(p), f_M(p))$
.
Proposition 4.1. Let
![]() $X_d^{\bullet }$
be a twist of
$X_d^{\bullet }$
be a twist of
![]() $X_0$
with
$X_0$
with
![]() $\bullet \in \{ B, C \}$
, and let p be a prime greater than
$\bullet \in \{ B, C \}$
, and let p be a prime greater than
![]() $3$
, where
$3$
, where
![]() $X_d^{\bullet }$
have good reduction at p. Table 1 gives the L-factors for
$X_d^{\bullet }$
have good reduction at p. Table 1 gives the L-factors for
![]() $J_d^{\bullet }$
(with
$J_d^{\bullet }$
(with
![]() $a_p = a_{p}(E_0)$
).
$a_p = a_{p}(E_0)$
).
Proof. This is an application of [Reference Fité and Sutherland5, Proposition 4.9] in our cases, but we note that [Reference Fité and Sutherland5] uses a normalisation
![]() $a_{p, i}(J)/p^{{i}/{2}}$
and
$a_{p, i}(J)/p^{{i}/{2}}$
and
![]() $a_1(E)(p) = -a_p(E_0)$
. (For example, the L-factor of an elliptic curve is
$a_1(E)(p) = -a_p(E_0)$
. (For example, the L-factor of an elliptic curve is
![]() $1 + a_1(E)(p)T + T^2$
in [Reference Fité and Sutherland5, page 555].)
$1 + a_1(E)(p)T + T^2$
in [Reference Fité and Sutherland5, page 555].)
By Corollary 3.4,
![]() $L = K(i)$
or
$L = K(i)$
or
![]() $L = K$
. By [Reference Fité and Sutherland5, Proposition 4.9], the possible values of
$L = K$
. By [Reference Fité and Sutherland5, Proposition 4.9], the possible values of
![]() $I(p)$
are
$I(p)$
are
and
![]() $I(p)$
determines
$I(p)$
determines
![]() $a_{p, 1}$
and
$a_{p, 1}$
and
![]() $a_{p, 2}$
. When
$a_{p, 2}$
. When
![]() $L=K(i)$
, which is a triquadratic field, the first entry of
$L=K(i)$
, which is a triquadratic field, the first entry of
![]() $I(p)$
cannot be
$I(p)$
cannot be
![]() $4$
. This gives the first three columns of Table 1 and the last one can be easily computed.
$4$
. This gives the first three columns of Table 1 and the last one can be easily computed.
When
![]() $L = K$
, the only possible value of
$L = K$
, the only possible value of
![]() $I(p)$
is one of
$I(p)$
is one of
![]() $(1,1,1)$
,
$(1,1,1)$
,
![]() $(2, 2, 1)$
and
$(2, 2, 1)$
and
![]() $(2, 2, 2)$
. The other results are not changed.
$(2, 2, 2)$
. The other results are not changed.
We note that each entry of the fourth column in Table 1 can be factorised, namely
![]() $(1 - a_pp^{-s} + p^{1-2s})^2$
,
$(1 - a_pp^{-s} + p^{1-2s})^2$
,
![]() $(1 + a_pp^{-s} + p^{1-2s})^2$
,
$(1 + a_pp^{-s} + p^{1-2s})^2$
,
![]() $(1 - a_pp^{-s} + p^{1-2s})(1 + a_pp^{-s} + p^{1-2s})$
and
$(1 - a_pp^{-s} + p^{1-2s})(1 + a_pp^{-s} + p^{1-2s})$
and
![]() $(1 + p^{1-2s})^2$
.
$(1 + p^{1-2s})^2$
.
Suppose that
![]() $L = K(i)$
. Since there is no rational prime whose residue degree is
$L = K(i)$
. Since there is no rational prime whose residue degree is
![]() $4$
in a biquadratic extension, the conditions on
$4$
in a biquadratic extension, the conditions on
![]() $I(p)$
are:
$I(p)$
are:
-
•
 $I(p) = (1, 1, 1)$
if and only if p totally splits in L;
$I(p) = (1, 1, 1)$
if and only if p totally splits in L; -
•
 $I(p) = (2, 1, 1)$
if and only if p is inert in
$I(p) = (2, 1, 1)$
if and only if p is inert in
 $\mathbb {Q}(i)$
and totally splits in K;
$\mathbb {Q}(i)$
and totally splits in K; -
•
 $I(p) = (2, 2, 1)$
if and only if p is inert in
$I(p) = (2, 2, 1)$
if and only if p is inert in
 $\mathbb {Q}(\sqrt {d})$
and splits in
$\mathbb {Q}(\sqrt {d})$
and splits in
 $\mathbb {Q}(\sqrt {-3})$
;
$\mathbb {Q}(\sqrt {-3})$
; -
•
 $I(p) = (2, 2, 2)$
if and only if p is inert in
$I(p) = (2, 2, 2)$
if and only if p is inert in
 $\mathbb {Q}(\sqrt {-3})$
.
$\mathbb {Q}(\sqrt {-3})$
.
Let us define
 $$ \begin{align*} &S_1 = \{ p : I(p) = (1, 1, 1) \}, \quad S_2 = \{ p : I(p) = (2, 1, 1) \}, \\ & S_3 = \{ p : I(p) = (2, 2, 1) \}, \quad S_4 = \{ p : I(p) = (2, 2, 2) \}. \end{align*} $$
$$ \begin{align*} &S_1 = \{ p : I(p) = (1, 1, 1) \}, \quad S_2 = \{ p : I(p) = (2, 1, 1) \}, \\ & S_3 = \{ p : I(p) = (2, 2, 1) \}, \quad S_4 = \{ p : I(p) = (2, 2, 2) \}. \end{align*} $$
It follows that half of the primes are in
![]() $S_4$
,
$S_4$
,
![]() $\tfrac 14$
in
$\tfrac 14$
in
![]() $S_3$
and
$S_3$
and
![]() $\tfrac 18$
in each of
$\tfrac 18$
in each of
![]() $S_2, S_1$
.
$S_2, S_1$
.
When
![]() $L = K$
, a rational prime is an element of
$L = K$
, a rational prime is an element of
![]() $S_1$
,
$S_1$
,
![]() $S_3$
or
$S_3$
or
![]() $S_4$
. Also in this case:
$S_4$
. Also in this case:
-
•
 $p \in S_1$
if and only if p totally splits in
$p \in S_1$
if and only if p totally splits in
 $L=K$
;
$L=K$
; -
•
 $p \in S_3$
if and only if p is inert in
$p \in S_3$
if and only if p is inert in
 $\mathbb {Q}(\sqrt {d})$
and splits in
$\mathbb {Q}(\sqrt {d})$
and splits in
 $\mathbb {Q}(\sqrt {-3})$
;
$\mathbb {Q}(\sqrt {-3})$
; -
•
 $p \in S_4$
if and only if p is inert in
$p \in S_4$
if and only if p is inert in
 $\mathbb {Q}(\sqrt {-3})$
.
$\mathbb {Q}(\sqrt {-3})$
.
Hence, in this case, half of the primes are in
![]() $S_4$
and
$S_4$
and
![]() $\tfrac 14$
in each of
$\tfrac 14$
in each of
![]() $S_3, S_1$
.
$S_3, S_1$
.
Proof of Theorem 1.1.
We first consider the case
![]() $L = K(i)$
. By Proposition 4.1,
$L = K(i)$
. By Proposition 4.1,
 $$ \begin{align*} L(J_d^{\bullet}/\mathbb{Q}, s)^{-1} &\sim \prod_{p \in S_1} ({ 1 - a_pp^{-s} + p^{1-2s} })^2 \prod_{p \in S_2} ({ 1 + a_pp^{-s} + p^{1-2s} })^2\\ &\quad \times \prod_{p \in S_3} (1 - a_pp^{-s} + p^{1-2s})(1 + a_p p^{-s} + p^{1-2s}) \prod_{p \in S_4} (1 + p^{1-2s})^2, \end{align*} $$
$$ \begin{align*} L(J_d^{\bullet}/\mathbb{Q}, s)^{-1} &\sim \prod_{p \in S_1} ({ 1 - a_pp^{-s} + p^{1-2s} })^2 \prod_{p \in S_2} ({ 1 + a_pp^{-s} + p^{1-2s} })^2\\ &\quad \times \prod_{p \in S_3} (1 - a_pp^{-s} + p^{1-2s})(1 + a_p p^{-s} + p^{1-2s}) \prod_{p \in S_4} (1 + p^{1-2s})^2, \end{align*} $$
where
![]() $\bullet \in \{ B, C \}$
. Here,
$\bullet \in \{ B, C \}$
. Here,
![]() $\sim $
means that the quantities are the same at unramified primes. We consider L-functions of
$\sim $
means that the quantities are the same at unramified primes. We consider L-functions of
![]() $E_0^{(-1)} \times E_0^{(-d)}$
. Note that
$E_0^{(-1)} \times E_0^{(-d)}$
. Note that
![]() $p\equiv 2\pmod {3}$
if and only if p is inert in
$p\equiv 2\pmod {3}$
if and only if p is inert in
![]() $\mathbb {Q}(\sqrt {-3})$
. Since
$\mathbb {Q}(\sqrt {-3})$
. Since
![]() $a_p = 0$
when
$a_p = 0$
when
![]() $p \equiv 2 \pmod {3}$
,
$p \equiv 2 \pmod {3}$
,
 $$ \begin{align*} L(E_0^{(D)}/\mathbb{Q}, s)^{-1} &= \prod_p (1 - a_p\chi_{D}(p)p^{-s} + p^{1 - 2s} ) \\ &= \prod_{p \in S_1} (1 - a_p\chi_{D}(p)p^{-s} + p^{1 - 2s} ) \prod_{p \in S_2} (1 - a_p\chi_{D}(p)p^{-s} + p^{1 - 2s} )\\ &\quad \times \prod_{p \in S_3} (1 - a_p\chi_{D}(p)p^{-s} + p^{1 - 2s} ) \prod_{p \in S_4} (1 + p^{1 - 2s} ). \end{align*} $$
$$ \begin{align*} L(E_0^{(D)}/\mathbb{Q}, s)^{-1} &= \prod_p (1 - a_p\chi_{D}(p)p^{-s} + p^{1 - 2s} ) \\ &= \prod_{p \in S_1} (1 - a_p\chi_{D}(p)p^{-s} + p^{1 - 2s} ) \prod_{p \in S_2} (1 - a_p\chi_{D}(p)p^{-s} + p^{1 - 2s} )\\ &\quad \times \prod_{p \in S_3} (1 - a_p\chi_{D}(p)p^{-s} + p^{1 - 2s} ) \prod_{p \in S_4} (1 + p^{1 - 2s} ). \end{align*} $$
We have
![]() $\chi _{-1}(p) = \chi _{-d}(p) = 1$
for a prime in
$\chi _{-1}(p) = \chi _{-d}(p) = 1$
for a prime in
![]() $S_1$
and
$S_1$
and
![]() $\chi _{-1}(p) = -1, \chi _{-d}(p) = 1$
for a prime in
$\chi _{-1}(p) = -1, \chi _{-d}(p) = 1$
for a prime in
![]() $S_2$
. In
$S_2$
. In
![]() $S_3$
, there are two subclasses of primes:
$S_3$
, there are two subclasses of primes:
Since
![]() $p \in S_3$
if and only if
$p \in S_3$
if and only if
![]() $\chi _d(p) = -1$
, we have
$\chi _d(p) = -1$
, we have
![]() $\chi _{-d}(p)=\chi _{-1}(p)\chi _d(p)=-\chi _{-1}(p)$
. Hence, for a prime
$\chi _{-d}(p)=\chi _{-1}(p)\chi _d(p)=-\chi _{-1}(p)$
. Hence, for a prime
![]() $p \in S_3$
, p is in
$p \in S_3$
, p is in
![]() $S_{3, 1}$
if and only if
$S_{3, 1}$
if and only if
![]() $\chi _{-1}(p) = 1$
and p is in
$\chi _{-1}(p) = 1$
and p is in
![]() $S_{3, 2}$
if and only if
$S_{3, 2}$
if and only if
![]() $\chi _{-d}(p) = 1$
. Consequently,
$\chi _{-d}(p) = 1$
. Consequently,
![]() $L(E_0^{(-1)}/\mathbb {Q}, s)^{-1}$
is given by
$L(E_0^{(-1)}/\mathbb {Q}, s)^{-1}$
is given by
 $$ \begin{align*} \prod_{p \in S_1 \cup S_{3, 1}} (1 - a_pp^{-s} + p^{1 - 2s} ) \prod_{p \in S_2 \cup S_{3, 2}} (1 + a_pp^{-s} + p^{1 - 2s} ) \prod_{p \in S_4} (1 + p^{1 - 2s} ) \end{align*} $$
$$ \begin{align*} \prod_{p \in S_1 \cup S_{3, 1}} (1 - a_pp^{-s} + p^{1 - 2s} ) \prod_{p \in S_2 \cup S_{3, 2}} (1 + a_pp^{-s} + p^{1 - 2s} ) \prod_{p \in S_4} (1 + p^{1 - 2s} ) \end{align*} $$
and
![]() $L(E_0^{(-d)}/\mathbb {Q}, s)^{-1}$
is given by
$L(E_0^{(-d)}/\mathbb {Q}, s)^{-1}$
is given by
 $$ \begin{align*} \prod_{p \in S_1 \cup S_{3, 2}} (1 - a_pp^{-s} + p^{1 - 2s} ) \prod_{p \in S_2 \cup S_{3, 1}} (1 + a_pp^{-s} + p^{1 - 2s} ) \prod_{p \in S_4} (1 + p^{1 - 2s} ). \end{align*} $$
$$ \begin{align*} \prod_{p \in S_1 \cup S_{3, 2}} (1 - a_pp^{-s} + p^{1 - 2s} ) \prod_{p \in S_2 \cup S_{3, 1}} (1 + a_pp^{-s} + p^{1 - 2s} ) \prod_{p \in S_4} (1 + p^{1 - 2s} ). \end{align*} $$
Therefore,
![]() $L(J_d^{\bullet }/\mathbb {Q}, s) \sim L(E_0^{(-1)}/\mathbb {Q}, s)L(E_0^{(-d)}/\mathbb {Q}, s),$
which means that
$L(J_d^{\bullet }/\mathbb {Q}, s) \sim L(E_0^{(-1)}/\mathbb {Q}, s)L(E_0^{(-d)}/\mathbb {Q}, s),$
which means that
![]() $J_d^{\bullet }$
is isogenous to
$J_d^{\bullet }$
is isogenous to
![]() $E_0^{(-1)} \times E_{0}^{(-d)}$
over
$E_0^{(-1)} \times E_{0}^{(-d)}$
over
![]() $\mathbb {Q}$
. When
$\mathbb {Q}$
. When
![]() $L = K$
, the same argument shows that
$L = K$
, the same argument shows that
![]() $J_d^\bullet $
is isogenous to
$J_d^\bullet $
is isogenous to
![]() $E_0 \times E_0^{(d)}$
.
$E_0 \times E_0^{(d)}$
.
Let
![]() $\rho _{J, \ell }$
be the
$\rho _{J, \ell }$
be the
![]() $\ell $
-adic Galois representation attached to the abelian variety J. It is well known that
$\ell $
-adic Galois representation attached to the abelian variety J. It is well known that
![]() $\rho _{J^{(D)}, \ell } \sim \rho _{J, \ell } \otimes \chi _D$
and
$\rho _{J^{(D)}, \ell } \sim \rho _{J, \ell } \otimes \chi _D$
and
![]() $J_1$
is isogenous to
$J_1$
is isogenous to
![]() $J_2$
if and only if
$J_2$
if and only if
![]() $\rho _{J_1} \sim \rho _{J_2}$
(see [Reference Silverman6, Section III.7]). Hence, for
$\rho _{J_1} \sim \rho _{J_2}$
(see [Reference Silverman6, Section III.7]). Hence, for
![]() $\bullet \in \{ B, C \}$
,
$\bullet \in \{ B, C \}$
,
 $$ \begin{align*} \rho_{J_d^{\bullet, (D)}, \ell} & \sim \rho_{J_d^\bullet, \ell} \otimes \chi_D \sim \rho_{E_0^{(-1)} \times E_0^{(-d)}} \otimes \chi_D = ({\rho_{E_0^{(-1)}} \oplus \rho_{E_0^{(-d)}} }) \otimes \chi_D \\ & = ({ \rho_{E_0^{(-1)}} \otimes \chi_D }) \oplus ({ \rho_{E_0^{(-d)}} \otimes \chi_D }) = \rho_{E_0^{(-D)}} \oplus \rho_{E_0^{(-dD)}}. \end{align*} $$
$$ \begin{align*} \rho_{J_d^{\bullet, (D)}, \ell} & \sim \rho_{J_d^\bullet, \ell} \otimes \chi_D \sim \rho_{E_0^{(-1)} \times E_0^{(-d)}} \otimes \chi_D = ({\rho_{E_0^{(-1)}} \oplus \rho_{E_0^{(-d)}} }) \otimes \chi_D \\ & = ({ \rho_{E_0^{(-1)}} \otimes \chi_D }) \oplus ({ \rho_{E_0^{(-d)}} \otimes \chi_D }) = \rho_{E_0^{(-D)}} \oplus \rho_{E_0^{(-dD)}}. \end{align*} $$
This proves the result.
We give two remarks on Theorem 1.1. Some values of d are naturally excluded in the family
![]() $\{X_d^B\}$
or
$\{X_d^B\}$
or
![]() $\{X_d^C\}$
since they do not define a twist of biquadratic type. Also, Theorem 1.1 shows that two curves in the family
$\{X_d^C\}$
since they do not define a twist of biquadratic type. Also, Theorem 1.1 shows that two curves in the family
![]() $\{X_d^B\}$
(or
$\{X_d^B\}$
(or
![]() $\{X_d^C\}$
), where
$\{X_d^C\}$
), where
![]() $d \in \mathbb {Q}^\times /(\mathbb {Q}^\times )^2$
, are not isogenous.
$d \in \mathbb {Q}^\times /(\mathbb {Q}^\times )^2$
, are not isogenous.
4.2. Remarks for other types
In this section, we give some remarks on the other types
![]() $A, D, E$
and G. Compared with the types B and C, there are two main obstacles.
$A, D, E$
and G. Compared with the types B and C, there are two main obstacles.
-
(i) The Brauer group condition becomes nontrivial.
-
(ii) For each integer d, we have to find a solution
 $x,y\in \mathbb {Q}$
of (4.1)In practice, we should express x and y as rational functions in d.
$x,y\in \mathbb {Q}$
of (4.1)In practice, we should express x and y as rational functions in d. $$ \begin{align} x^2+\frac{3y^2}{v(d)}=u(d). \end{align} $$
$$ \begin{align} x^2+\frac{3y^2}{v(d)}=u(d). \end{align} $$
Note that when we deal with types B and C, we have easy solutions for obstacle (ii).
In some cases, we may restrict d to make obstacle (i) simpler. However, even in this case, obstacle (ii) may remain nontrivial. In the following examples, we numerically verify the expected nonsimplicity for some small d by finding explicit solutions
![]() $(\alpha , \beta )$
of (4.1).
$(\alpha , \beta )$
of (4.1).
Example 4.2. The Brauer group condition
![]() $(d, -3) = 1 \in \operatorname {\mathrm {Br}}_2(\mathbb {Q})$
for type A is equivalent to
$(d, -3) = 1 \in \operatorname {\mathrm {Br}}_2(\mathbb {Q})$
for type A is equivalent to
![]() $d \equiv 1 \pmod {3}$
when d is prime. In this case,
$d \equiv 1 \pmod {3}$
when d is prime. In this case,
![]() $(\alpha , \beta )$
is a solution of
$(\alpha , \beta )$
is a solution of
For example, we have
![]() $(\alpha , \beta ) = (2, 1)$
when
$(\alpha , \beta ) = (2, 1)$
when
![]() $d = 7$
. With the calculations of previous sections, we find the list of
$d = 7$
. With the calculations of previous sections, we find the list of
![]() $(\alpha , \beta )$
and
$(\alpha , \beta )$
and
![]() $f_d$
for primes
$f_d$
for primes
![]() $d < 70$
shown in Table 2.
$d < 70$
shown in Table 2.
Table 2 The solutions
![]() $(\alpha,\beta)$
associated to d and the resulting
$(\alpha,\beta)$
associated to d and the resulting
![]() $f_d$
for type A.
$f_d$
for type A.

By computing the discriminant of each quadratic divisor of
![]() $f_d$
, we have
$f_d$
, we have
![]() $L_d = \mathbb {Q}(\sqrt {3d})$
, which is a subfield of
$L_d = \mathbb {Q}(\sqrt {3d})$
, which is a subfield of
![]() $K(i)$
. By Proposition 3.3,
$K(i)$
. By Proposition 3.3,
![]() $L = K$
or
$L = K$
or
![]() $L = K(i)$
. Hence, the proof of Proposition 4.1, and Theorem 1.1 also works. Consequently,
$L = K(i)$
. Hence, the proof of Proposition 4.1, and Theorem 1.1 also works. Consequently,
![]() $J_d^A$
is not simple for primes
$J_d^A$
is not simple for primes
![]() $d < 70$
satisfying the Brauer group condition.
$d < 70$
satisfying the Brauer group condition.
Example 4.3. In the case of type D and prime d, the Brauer group condition gives
![]() $d \equiv 1 \pmod {4}$
. Since numerical computation is too complicated, we omit a detailed description as in the previous example. For such primes
$d \equiv 1 \pmod {4}$
. Since numerical computation is too complicated, we omit a detailed description as in the previous example. For such primes
![]() $d < 70$
, the factorisation of
$d < 70$
, the factorisation of
![]() $f_d(x)$
over
$f_d(x)$
over
![]() $\mathbb {Q}(i)$
shows that
$\mathbb {Q}(i)$
shows that
![]() $L_d$
is a quartic extension of
$L_d$
is a quartic extension of
![]() $\mathbb {Q}$
whose unique intermediate field is
$\mathbb {Q}$
whose unique intermediate field is
![]() $\mathbb {Q}(i)$
. By the proof of Proposition 3.3,
$\mathbb {Q}(i)$
. By the proof of Proposition 3.3,
![]() $L_{\phi }$
is a subfield of
$L_{\phi }$
is a subfield of
![]() $L_d(i) = L_d$
. Hence, L is a subfield of
$L_d(i) = L_d$
. Hence, L is a subfield of
![]() $L_dK$
with
$L_dK$
with
![]() $[L:K] \leq 2$
. Therefore, we can conclude that
$[L:K] \leq 2$
. Therefore, we can conclude that
![]() $L = K$
or
$L = K$
or
![]() $K(i)$
. This implies that
$K(i)$
. This implies that
![]() $J_d^D$
is not simple for primes
$J_d^D$
is not simple for primes
![]() $d < 70$
.
$d < 70$
.
Example 4.4. For type E, the Brauer group condition makes the prime
![]() $d \equiv 1 \pmod {12}$
. Analogous computation shows that
$d \equiv 1 \pmod {12}$
. Analogous computation shows that
![]() $f_d$
has three quadratic factors over
$f_d$
has three quadratic factors over
![]() $\mathbb {Q}(\sqrt {3})$
and
$\mathbb {Q}(\sqrt {3})$
and
![]() $L_d$
is a quartic extension whose quadratic subfield is
$L_d$
is a quartic extension whose quadratic subfield is
![]() $\mathbb {Q}(\sqrt {3})$
. By the same argument as for type D, we have checked that
$\mathbb {Q}(\sqrt {3})$
. By the same argument as for type D, we have checked that
![]() $J_d^E$
is not simple for primes
$J_d^E$
is not simple for primes
![]() $d < 190$
.
$d < 190$
.
Example 4.5. In the case of type G, a simple computation shows that
![]() $d = -p$
with
$d = -p$
with
![]() $p \equiv 1 \pmod {3}$
satisfies the Brauer group condition. This case may require more effort to find explicit
$p \equiv 1 \pmod {3}$
satisfies the Brauer group condition. This case may require more effort to find explicit
![]() $\alpha , \beta $
.
$\alpha , \beta $
.