Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-27T07:34:48.104Z Has data issue: false hasContentIssue false
  • English
  • Français

CONVERGENCE IN RELAXATION SPECTRUM RECOVERY

Part of: Fredholm integral equations Integral transforms, operational calculus

Published online by Cambridge University Press:  02 November 2016

R. J. LOY ,
F. R. DE HOOG  and
R. S. ANDERSSEN
R. J. LOY
Affiliation:
Mathematical Sciences Institute, Australian National University, John Dedman Building 27, Union Lane, Canberra, ACT 2601, Australia email [email protected]
F. R. DE HOOG
Affiliation:
CSIRO Data61, GPO Box 664, Canberra, ACT 2601, Australia email [email protected]
R. S. ANDERSSEN*
Affiliation:
CSIRO Data61, GPO Box 664, Canberra, ACT 2601, Australia email [email protected]
*
✉ [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Because of its practical and theoretical importance in rheology, numerous algorithms have been proposed and utilised to solve the convolution equation 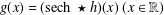$g(x)=(\text{sech}\,\star h)(x)\;(x\in \mathbb{R})$ for 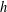$h$ , given 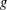$g$ . There are several approaches involving the use of series expansions of derivatives of $g$ , which are then truncated to a small number of terms for practical application. Such truncations can only be expected to be valid if the infinite series converge. In this note, we examine two specific truncations and provide a rigorous analysis to obtain sufficient conditions on 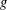$g$ (and equivalently on 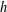$h$ ) for the convergence of the series concerned.

Keywords

relaxation spectrumconvolution equation

MSC classification

Primary: 44A35: Convolution
Secondary: 45B05: Fredholm integral equations
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 95 , Issue 1 , February 2017 , pp. 121 - 132
Copyright
© 2016 Australian Mathematical Publishing Association Inc. 

References

Anderssen, R. S., Davies, A. R., de Hoog, F. R. and Loy, R. J., ‘Derivative based algorithms for continuous relaxation spectrum’, J. Non-Newtonian Fluid Mech. 222 (2015), 132–140.Google Scholar
Anderssen, R. S., Davies, A. R., de Hoog, F. R. and Loy, R. J., ‘Simple joint inversion localized formulas for relaxation spectrum recovery’, ANZIAM J. 58 (2016), 1–9.Google Scholar
Boas, R. P., Entire functions (Academic Press, New York, 1954).Google Scholar
Boyadzhiev, K., ‘Derivative polynomials for tanh, tan, sech, and sec in explicit form’, Fibonacci Quart. 45(4) (2007), 291–303.Google Scholar
Ferry, J., Viscoelastic Properties of Polymers (Wiley, New York, 1980).Google Scholar
Gradsteyn, I. S. and Ryzhik, I. M., Tables of Integrals, Series and Products (Elsevier Academic Press, San Diego, 2007).Google Scholar
Guyerev, T. E., Nesterets, Y. I., Stevenson, A. W. and Wilkins, S. W., ‘A method for local deconvolution’, Appl. Optics 42 (2003), 6488–6494.Google Scholar
Hewitt, E. and Stromberg, K., Real and Abstract Analysis (Springer, New York, 1975).Google Scholar
Schwarzl, F. and Staverman, A. J., ‘Higher approximation methods for the relaxation spectrum from static and dynamic measurements of visco-elastic materials’, Appl. Sci. Res. A 4 (1953), 127–141.CrossRefGoogle Scholar
Schwarzl, F. and Staverman, A. J., ‘Linear deformation behaviour of high polymers’, in: Die Physik der Hochpolmeren, 4: Theorie und molekulare Deutung technologischer Eigenschaften von Hochpolymeren Werkstoffen (ed. Stuart, H. A.) (Springer, New York, 1956).Google Scholar