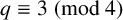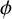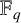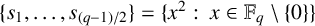A CONJECTURE OF ZHI-WEI SUN ON MATRICES CONCERNING MULTIPLICATIVE SUBGROUPS OF FINITE FIELDS

Abstract

Motivated by the recent work of Zhi-Wei Sun [‘Problems and results on determinants involving Legendre symbols’, Preprint, arXiv:2405.03626], we study some matrices concerning subgroups of finite fields. For example, let $q\equiv 3\pmod 4$ be an odd prime power and let $\phi $ be the unique quadratic multiplicative character of the finite field $\mathbb {F}_q$. If the set $\{s_1,\ldots ,s_{(q-1)/2}\}=\{x^2:\ x\in \mathbb {F}_q\setminus \{0\}\}$, then we prove that

$$ \begin{align*}\det[t+\phi(s_i+s_j)+\phi(s_i-s_j)]_{1\le i,j\le (q-1)/2}=\bigg(\frac{q-1}{2}t-1\bigg)q^{{(q-3)}/{4}}.\end{align*} $$

This confirms a conjecture of Zhi-Wei Sun.

1. Introduction

Let p be an odd prime. Research on determinants involving the Legendre symbol $(\frac {\cdot }{p})$ can be traced back to Lehmer [4], Carlitz [1] and Chapman [2]. For example, Carlitz [1, Theorem 4] studied the determinant

$$ \begin{align*} \det C(t):=\det\bigg[t+\bigg(\frac{i-j}{p}\bigg)\bigg]_{1\le i,j\le p-1} \end{align*} $$

and showed that

$$ \begin{align*}\det C(t)=(-1)^{{(p-1)}/{2}}p^{{(p-3)}/{2}}((p-1)t+(-1)^{{(p-1)}/{2}}).\end{align*} $$

Chapman [2] investigated some variants of $\det C(t)$ . For instance, Chapman considered

$$ \begin{align*}\det C_1(t):=\det \bigg[t+\bigg(\frac{i+j-1}{p}\bigg)\bigg]_{1\le i,j\le (p-1)/2}.\end{align*} $$

If we let $\varepsilon _p>1$ and $h_p$ be the fundamental unit and the class number of $\mathbb {Q}(\sqrt {p})$ , respectively, then Chapman [2] proved that

$$ \begin{align*}\det C_1(t)=\begin{cases} (-1)^{{(p-1)}/{4}}2^{{(p-1)}/{2}}(-a_pt+b_p) & \text{if}\ p\equiv 1\pmod4,\\ -2^{{(p-1)}/{2}}t & \text{if}\ p\equiv 3\pmod4, \end{cases}\end{align*} $$

where $a_p,b_p\in \mathbb {Q}$ are defined by the equality

$$ \begin{align*}\varepsilon_p^{h_p}=a_p+b_p\sqrt{p}.\end{align*} $$

In 2019, Sun [5] initiated the study of determinants involving the Legendre symbol and binary quadratic forms. For example, Sun considered the determinant

$$ \begin{align*}\det S_p:=\det\bigg[\bigg(\frac{i^2+j^2}{p}\bigg)\bigg]_{1\le i,j\le (p-1)/2}.\end{align*} $$

Sun [5, Theorem 1.2] showed that $-\det S_p$ is always a quadratic residue modulo p. See also [3, 7] for recent work on this topic.

Recently, Sun [6] posed many interesting conjectures on determinants related to the Legendre symbol. We give one example.

Conjecture 1.1 (Sun; [6, Conjecture 1.1])

Let $p\equiv 3\pmod 4$ be a prime. Then,

$$ \begin{align*}\det\bigg[t+\bigg(\frac{i^2+j^2}{p}\bigg)+\bigg(\frac{i^2-j^2}{p}\bigg)\bigg]_{1\le i,j\le (p-1)/2}=\bigg(\frac{p-1}{2}t-1\bigg)p^{{(p-3)}/{4}}.\end{align*} $$

Motivated by these results, we will study some determinants involving the quadratic multiplicative character of a finite field. We first introduce some notation.

Let $q=p^s$ be an odd prime power with p prime and $s\in \mathbb {Z}^+$ and let $\mathbb {F}_q$ be the finite field of q elements. Let $\mathbb {F}_q^{\times }$ be the cyclic group of all nonzero elements of $\mathbb {F}_q$ . For any positive integer k which divides $q-1$ , let

$$ \begin{align*}D_k:=\{a_1,a_2,\ldots,a_{(q-1)/k}\}=\{x^k:\ x\in\mathbb{F}_q^{\times}\}\end{align*} $$

be the subgroup of all nonzero kth powers in $\mathbb {F}_q$ .

Let $\widehat {\mathbb {F}_q^{\times }}$ be the cyclic group of all multiplicative characters of $\mathbb {F}_q$ . Throughout this paper, for any $\psi \in \widehat {\mathbb {F}_q^{\times }}$ , we extend $\psi $ to $\mathbb {F}_q$ by setting $\psi (0)=0$ . Also, if $2\nmid q$ , we use the symbol $\phi $ to denote the unique quadratic multiplicative character of $\mathbb {F}_q$ , that is,

$$ \begin{align*} \phi(x)=\begin{cases} 1 & \text{if}\ x\in D_2,\\ 0 & \text{if}\ x=0,\\ -1 & \text{otherwise.} \end{cases} \end{align*} $$

Inspired by the above results, we define the matrix $A_k(t)$ by

$$ \begin{align*}A_k(t):=[t+\phi(a_i+a_j)+\phi(a_i-a_j)]_{1\le i,j\le (q-1)/k}.\end{align*} $$

The integers $c_k$ and $d_k$ , which are related to the number of $\mathbb {F}_q$ -rational points of certain hyperelliptic curves over $\mathbb {F}_q$ , are defined by

(1.1) $$ \begin{align} |\{\infty\}\cup\{(x,y)\in\mathbb{F}_q\times\mathbb{F}_q:\ y^2=x^k+1\}|=q+1-c_k \end{align} $$

and

(1.2) $$ \begin{align} |\{\infty\}\cup\{(x,y)\in\mathbb{F}_q\times\mathbb{F}_q:\ y^2=x^k-1\}|=q+1-d_k. \end{align} $$

Now we state the main results of this paper.

Theorem 1.2. Let $q=p^s$ be an odd prime power with p prime and $s\in \mathbb {Z}^+$ . Then, for any positive integer k which divides $q-1$ , the following results hold.

1. (i) Suppose $q\equiv 1\pmod {2k}$ . Then $\det A_k(t)=0$ . In particular, if $q\equiv 1\pmod 4$ , then $\det A_2(t)=0$ .

2. (ii) If $q\equiv 3\pmod 4$ , then

   $$ \begin{align*}\det A_2(t)=\bigg(\frac{q-1}{2}t-1\bigg)q^{{(q-3)}/{4}}.\end{align*} $$

3. (iii) Suppose $q\equiv 1\pmod 4$ and $q\not \equiv 1\pmod {2k}$ . Then there is an integer $u_k$ such that

   $$ \begin{align*}\det A_k(t)=\bigg(\frac{q-1}{k}t-\frac{1}{k}(c_k+d_k+2)\bigg)\cdot u_k^2.\end{align*} $$

Remark 1.3. (i) Theorem 1.2(i) generalises [6, Theorem 1.1] to an arbitrary finite field with odd characteristic. In the case where $q=p$ is an odd prime, Theorem 1.2(ii) confirms Conjecture 1.1 posed by Zhi-Wei Sun.

(ii) For any k with $3\le k<q-1$ , $k\mid q-1$ and $q-1\not \equiv 0\pmod {2k}$ , we can also obtain the explicit value of $\det A_k(t)$ . However, finding a simple expression for $\det A_k(t)$ seems very difficult.

We will prove our main results in Section 2.

2. Proof of Theorem 1.2

Throughout this section, we let $\chi $ be a generator of $\widehat {\mathbb {F}_q^{\times }}$ . Also, for any $\chi ^i,\chi ^j\in \widehat {\mathbb {F}_q^{\times }}$ , the Jacobi sum of $\chi ^i$ and $\chi ^j$ is defined by

$$ \begin{align*}J(\chi^i,\chi^j)=\sum_{x\in\mathbb{F}_q}\chi^i(x)\chi^j(1-x).\end{align*} $$

We begin with a known result in linear algebra.

Lemma 2.1. Let n be a positive integer and let M be an $n\times n$ complex matrix. Let $\lambda _1,\ldots ,\lambda _n\in \mathbb {C}$ , and let $\mathbf{v}_1,\ldots ,\mathbf{v}_n\in \mathbb {C}^n$ be column vectors. Suppose that

$$ \begin{align*}M\mathbf{v}_i=\lambda_i\mathbf{v}_i\end{align*} $$

for $1\le i\le n$ and that the vectors $\mathbf{v}_1,\ldots ,\mathbf{v}_n$ are linearly independent over $\mathbb {C}$ . Then $\lambda _1,\ldots ,\lambda _n$ are exactly all the eigenvalues of M (counting multiplicity).

Before the proof of our main results, we first introduce the definition of circulant matrices. Let R be a commutative ring and let $b_0,b_1,\ldots ,b_{n-1}\in R$ . Then the circulant matrix of the tuple $(b_0,b_1,\ldots ,b_{n-1})$ is defined by

$$ \begin{align*}C(b_0,b_1,\ldots,b_{n-1}):=[b_{i-j}]_{0\le i,j\le n-1},\end{align*} $$

where the indices are cyclic modulo n.

The second author [7, Lemma 3.4] proved the following result.

Lemma 2.2. Let $n\ge 1$ be an odd integer. Let R be a commutative ring and let $b_0,\ldots ,b_{n-1}\in R$ such that $b_i=b_{n-i}$ for $1\le i\le n-1$ . Then there is an element $u\in R$ such that

$$ \begin{align*}\det C(b_0,b_1,\ldots,b_{n-1})=\bigg(\sum_{i=0}^{n-1}b_i\bigg)u^2.\end{align*} $$

Now we are in a position to prove our main results. For simplicity, we set ${n=(q-1)/k}$ .

Proof of Theorem 1.2

(i) Suppose $q-1\equiv 0\pmod {2k}$ . Let $\xi _{2k}\in \mathbb {F}_q$ be a primitive $2k$ th root of unity. Then $-1=\xi _{2k}^k\in D_k$ . Thus, for any j with $1\le j\le n$ , there exists an integer $j'$ with $1\le j'\le n$ such that $a_{j'}=-a_j$ and $j\neq j'$ . This implies that the jth column of $A_k(t)$ is the same as the $j'$ th column of $A_k(t)$ and hence $\det A_k(t)=0$ .

(ii) Suppose now $q-1\not \equiv 0\pmod {2k}$ . Then, clearly k is even. For any integers $m,n$ with $0\le m\le n-1$ and $1\le i\le n$ ,

$$ \begin{align*} \sum_{1\le j\le n} (\phi(a_i+a_j) +\phi(a_i-a_j))\chi^m(a_j) & =\sum_{1\le j\le n}\bigg(\phi\bigg(1+\frac{a_j}{a_i}\bigg)+\phi\bigg(1-\frac{a_j}{a_i}\bigg)\bigg)\ \chi^m\bigg(\frac{a_j}{a_i}\bigg)\ \chi^m(a_i)\\ & =\sum_{1\le j\le n}(\phi(1+a_j)+\phi(1-a_j))\chi^m(a_j)\chi^m(a_i). \end{align*} $$

Let

$$ \begin{align*}\mathbf{v}_m=(\chi^m(a_1),\chi^m(a_2),\ldots,\chi^m(a_n))^T \quad\text{and}\quad \lambda_m=\sum_{1\le j\le n}(\phi(1+a_j)+\phi(1-a_j))\chi^m(a_j).\end{align*} $$

By the above results,

$$ \begin{align*} A_k(0)\mathbf{v}_m=\lambda_m\mathbf{v}_m \quad\text{for } 0\le m\le n-1. \end{align*} $$

Since

$$ \begin{align*}\det [\chi^i(a_j)]_{0\le i\le n-1,1\le j\le n}=\prod_{1\le i<j\le n}(\chi(a_j)-\chi(a_i))\neq 0,\end{align*} $$

the vectors $\mathbf{v}_0,\ldots ,\mathbf{v}_{n-1}$ are linearly independent over $\mathbb {C}$ and hence by Lemma 2.1, the complex numbers $\lambda _0,\ldots ,\lambda _{n-1}$ are exactly all the eigenvalues of $A_k(0)$ .

Now let $k=2$ . Then clearly $q\equiv 3\pmod 4$ and n is odd in this case. We first evaluate $\det A_2(0)$ . By the above,

(2.1) $$ \begin{align} \det A_2(0)=\lambda_0\prod_{1\le m\le n-1}\lambda_m=\lambda_0\prod_{1\le m\le (n-1)/2}|\lambda_{2m}|^2. \end{align} $$

The last equality follows from $\overline {\lambda _m}=\lambda _{n-m}$ for $1\le m\le n-1$ . For $\lambda _0$ ,

(2.2) $$ \begin{align} \lambda_0 =\sum_{1\le j\le n}(\phi(1+a_j)+\phi(1-a_j)) =\frac{1}{2}\sum_{x\in\mathbb{F}_q^{\times}}\phi(1+x^2)-\frac{1}{2}\sum_{x\in\mathbb{F}_q^{\times}}\phi(x^2-1) =-1. \end{align} $$

The last equality follows from

$$ \begin{align*}\sum_{x\in\mathbb{F}_q}\phi(x^2\pm 1)=-1.\end{align*} $$

For $\lambda _{2m}$ with $1\le m\le (n-1)/2$ , one can verify that

(2.3) $$ \begin{align} \lambda_{2m} &=\sum_{1\le j\le n}(\phi(1+a_j)+\phi(1-a_j))\chi^{2m}(a_j) \notag \\ &=\frac{1}{2}\sum_{x\in\mathbb{F}_q}\phi(1+x^2)\chi^{2m}(x^2)+\frac{1}{2}\sum_{x\in\mathbb{F}_q}\phi(1-x^2)\chi^{2m}(-x^2) \notag \\ &=\sum_{x\in\mathbb{F}_q}\phi(1+x)\chi^{2m}(x) \notag \\ &=\sum_{x\in\mathbb{F}_q}\phi(1+x)\chi^{2m}(-x) =J(\phi,\chi^{2m}). \end{align} $$

Combining (2.2) and (2.3) with (2.1),

$$ \begin{align*}\det A_2(0)=-\prod_{1\le m\le (n-1)/2}|J(\phi,\chi^{2m})|^2=-q^{{(q-3)}/{4}}.\end{align*} $$

Now we turn to $\det A_2(t)$ . By (2.2) for $1\le j\le n$ ,

$$ \begin{align*} \sum_{1\le i\le n}(t+\phi(a_i+a_j)+\phi(a_i-a_j)) & = nt+\sum_{1\le i\le n}(\phi(1+a_j/a_i)+\phi(1-a_j/a_i))\\ & = nt+\sum_{1\le i\le n}(\phi(1+a_i)+\phi(1-a_i))\\ & = nt-1. \end{align*} $$

This implies that $(nt-1)\mid \det A_2(t)$ . Noting that $\det A_2(t)\in \mathbb {Z}[t]$ with degree $\le 1$ ,

$$ \begin{align*}\det A_2(t)=-\det A_2(0)\cdot(nt-1)=q^{{(q-3)}/{4}}\bigg(\frac{q-1}{2}t-1\bigg).\end{align*} $$

(iii) Suppose $q\equiv 1\pmod 4$ and $q\not \equiv 1\pmod {2k}$ . Clearly, $k\equiv 0\pmod 2$ in this case. Let $g\in \mathbb {F}_q$ be a generator of the cyclic group $\mathbb {F}_q^{\times }$ . Then one can verify that

$$ \begin{align*} \det A_k(t) &=\det[t+\phi(a_i+a_j)+\phi(a_i-a_j)]_{1\le i,j\le n}\\ &=\det [t+\phi(g^{k(i-j)}+1)+\phi(g^{k(i-j)}-1)]_{0\le i,j\le n-1}. \end{align*} $$

For $0\le i\le n-1$ , let

$$ \begin{align*}b_i=t+\phi(g^{ki}+1)+\phi(g^{ki}-1).\end{align*} $$

Then one can easily verify that

$$ \begin{align*}\det A_k(t)=\det C(b_0,b_1,\ldots,b_{n-1})\end{align*} $$

and that $b_i=b_{n-i}$ for $1\le i\le n-1$ . Now applying Lemma 2.2, we see that there is an element $u_k\in \mathbb {Z}[t]$ such that

$$ \begin{align*}\det A_k(t)=\bigg(\sum_{i=0}^{n-1}b_i\bigg)\cdot u_k^2.\end{align*} $$

One can verify that

$$ \begin{align*} \sum_{i=0}^{n-1}b_i &=nt+\sum_{1\le j\le n}(\phi(a_i+1)+\phi(a_i-1))\\ &=nt+\frac{1}{k}\sum_{x\in\mathbb{F}_q^{\times}}(\phi(x^k+1)+\phi(x^k-1))\\ &=nt-\frac{1}{k}(c_k+d_k+2), \end{align*} $$

where $c_k$ and $d_k$ are defined by (1.1) and (1.2), and the last equality follows from

$$ \begin{align*}\sum_{x\in\mathbb{F}_q^{\times}}\phi(x^k+1)=-c_k-1 \quad\text{and}\quad \sum_{x\in\mathbb{F}_q^{\times}}\phi(x^k-1)=-d_k-1.\end{align*} $$

As $\det A_k(t)\in \mathbb {Z}[t]$ with degree $\le 1$ , by the above, we see that $u_k\in \mathbb {Z}$ . Hence,

$$ \begin{align*}\det A_k(t)=\bigg(\frac{q-1}{k}t-\frac{1}{k}(c_k+d_k+2)\bigg)\cdot u_k^2.\end{align*} $$

In view of the above, we have completed the proof of Theorem 1.2.