Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Raatikainen, Panu
2003.
Some strongly undecidable natural arithmetical problems, with an application to intuitionistic theories.
Journal of Symbolic Logic,
Vol. 68,
Issue. 1,
p.
262.
Downey, Rod
and
Montalbán, Antonio
2008.
The isomorphism problem for torsion-free Abelian groups is analytic complete.
Journal of Algebra,
Vol. 320,
Issue. 6,
p.
2291.
BELEGRADEK, IGOR
and
SZCZEPAŃSKI, ANDRZEJ
2008.
ENDOMORPHISMS OF RELATIVELY HYPERBOLIC GROUPS.
International Journal of Algebra and Computation,
Vol. 18,
Issue. 01,
p.
97.
MELNIKOV, ALEXANDER G.
2014.
COMPUTABLE ABELIAN GROUPS.
The Bulletin of Symbolic Logic,
Vol. 20,
Issue. 3,
p.
315.
Bilanovic, Iva
Chubb, Jennifer
and
Roven, Sam
2020.
Detecting properties from descriptions of groups.
Archive for Mathematical Logic,
Vol. 59,
Issue. 3-4,
p.
293.
Wu, Huishan
2022.
The computational complexity of module socles.
Annals of Pure and Applied Logic,
Vol. 173,
Issue. 5,
p.
103089.
Wu, Huishan
2023.
The Complexity of Decomposability of Computable Rings.
Notre Dame Journal of Formal Logic,
Vol. 64,
Issue. 1,
Wu, Huishan
2024.
The Computational Complexity of Subclasses of Semiperfect Rings.
Mathematics,
Vol. 12,
Issue. 22,
p.
3608.
Harrison-Trainor, Matthew
and
Melnikov, Alexander
2024.
An arithmetic analysis of closed surfaces.
Transactions of the American Mathematical Society,
Wu, Huishan
2025.
Computably Enumerable Semisimple Rings.
Mathematics,
Vol. 13,
Issue. 3,
p.
337.


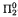 -complete. This implies in particular that there is no effective listing of all torsion-free finitely presented groups, or of all non-torsion-free finitely presented groups.
-complete. This implies in particular that there is no effective listing of all torsion-free finitely presented groups, or of all non-torsion-free finitely presented groups.