No CrossRef data available.
Article contents
BASE SHIFTING SEQUENCES AND THE PALINDROME PROPERTY
Published online by Cambridge University Press: 10 January 2025
Abstract
For  $M,N,m\in \mathbb {N}$ with
$M,N,m\in \mathbb {N}$ with  $M\geq 2, N\geq 1$ and
$M\geq 2, N\geq 1$ and  $m \geq 2$, we define two families of sequences,
$m \geq 2$, we define two families of sequences, 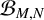 $\mathcal {B}_{M,N}$ and
$\mathcal {B}_{M,N}$ and 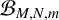 $\mathcal {B}_{M,N,m}$. The nth term of
$\mathcal {B}_{M,N,m}$. The nth term of 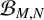 $\mathcal {B}_{M,N}$ is obtained by expressing n in base M and recombining those digits in base N. The nth term of
$\mathcal {B}_{M,N}$ is obtained by expressing n in base M and recombining those digits in base N. The nth term of 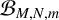 $\mathcal {B}_{M,N,m}$ is defined by
$\mathcal {B}_{M,N,m}$ is defined by  $\mathcal {B}_{M,N,m}(n):=\mathcal {B}_{M,N}(n) \; (\textrm {mod}\;m)$. The special case
$\mathcal {B}_{M,N,m}(n):=\mathcal {B}_{M,N}(n) \; (\textrm {mod}\;m)$. The special case 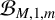 $\mathcal {B}_{M,1,m}$, where
$\mathcal {B}_{M,1,m}$, where  $N=1$, yields the digit sum sequence
$N=1$, yields the digit sum sequence 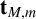 $\mathbf {t}_{M,m}$ in base M mod m. We prove that
$\mathbf {t}_{M,m}$ in base M mod m. We prove that  $\mathcal {B}_{M,N}$ is the fixed point of a morphism
$\mathcal {B}_{M,N}$ is the fixed point of a morphism 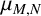 $\mu _{M,N}$ at letter
$\mu _{M,N}$ at letter 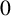 $0$, similar to a property of
$0$, similar to a property of  $\mathbf {t}_{M,m}$. Additionally, we show that
$\mathbf {t}_{M,m}$. Additionally, we show that  $\mathcal {B}_{M,N,m}$ contains arbitrarily long palindromes if and only if
$\mathcal {B}_{M,N,m}$ contains arbitrarily long palindromes if and only if  $m=2$, mirroring the behaviour of the digit sum sequence. When
$m=2$, mirroring the behaviour of the digit sum sequence. When 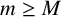 $m\geq M$ and N, m are coprime, we establish that
$m\geq M$ and N, m are coprime, we establish that 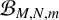 $\mathcal {B}_{M,N,m}$ contains no overlaps.
$\mathcal {B}_{M,N,m}$ contains no overlaps.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Author(s), 2025. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.



