Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-28T10:02:12.777Z Has data issue: false hasContentIssue false
  • English
  • Français

BARRELLED SPACES WITH(OUT) SEPARABLE QUOTIENTS

Part of: Maps and general types of spaces defined by maps Topological linear spaces and related structures

Published online by Cambridge University Press:  13 June 2014

JERZY KĄKOL ,
STEPHEN A. SAXON  and
AARON R. TODD
JERZY KĄKOL
Affiliation:
Faculty of Mathematics and Informatics, A. Mickiewicz University, 60-769 Poznań, Matejki 48–49,Poland email [email protected]
STEPHEN A. SAXON*
Affiliation:
Department of Mathematics, University of Florida, PO Box 118105, Gainesville, FL 32611-8105,USA email [email protected]
AARON R. TODD
Affiliation:
Department of Mathematics, Baruch College, CUNY, New York 10010,USA email [email protected]
*
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

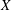While the separable quotient problem is famously open for Banach spaces, in the broader context of barrelled spaces we give negative solutions. Obversely, the study of pseudocompact $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}X$ and Warner bounded 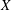$X$ allows us to expand Rosenthal’s positive solution for Banach spaces of the form 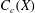$ C_{c}(X) $ to barrelled spaces of the same form, and see that strong duals of arbitrary 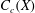$C_{c}(X) $ spaces admit separable quotients.

Keywords

barrelled spacesseparable quotientscompact-open topology

MSC classification

Secondary: 46A08: Barrelled spaces, bornological spaces 54C35: Function spaces
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 90 , Issue 2 , October 2014 , pp. 295 - 303
Copyright
Copyright © 2014 Australian Mathematical Publishing Association Inc. 

References

Argyros, S. A., Dodos, P. and Kanellopoulos, V., ‘Unconditional families in Banach spaces’, Math. Ann. 341 (2008), 15–38.Google Scholar
Pérez Carreras, P. and Bonet, J., ‘Una nota sobre un resultado de Eidelheit’, Collect. Math. 33 (1982), 195–199.Google Scholar
Pérez Carreras, P. and Bonet, J., Barrelled Locally Convex Spaces, Mathematics Studies, 131 (North-Holland, Amsterdam, 1987).Google Scholar
Eberhardt, V. and Roelcke, W., ‘Über einen Graphensatz für lineare Abbildungen mit metrisierbarem Zielraum’, Manuscripta Math. 13 (1974), 53–68.CrossRefGoogle Scholar
Eidelheit, M., ‘Zur Theorie der Systeme linearer Gleichungen’, Studia Math. 6 (1936), 130–148.Google Scholar
Ferrando, J. C., Kąkol, J. and Saxon, S. A., ‘The dual of the locally convex space C p(X)’, Funct. Approx. Comment. Math. 50(2) (2014), 1–11.Google Scholar
Kąkol, J., Kubiś, W. and López-Pellicer, M., Descriptive Topology in Selected Topics of Functional Analysis (Springer, New York, 2011).CrossRefGoogle Scholar
Kąkol, J., Saxon, S. A. and Todd, A. R., ‘Pseudocompact spaces X and d f-spaces C c(X)’, Proc. Amer. Math. Soc. 132 (2004), 1703–1712.Google Scholar
Kąkol, J., Saxon, S. A. and Todd, A. R., ‘The analysis of Warner boundedness’, Proc. Edinb. Math. Soc. 47 (2004), 625–631.Google Scholar
Kąkol, J., Saxon, S. A. and Todd, A. R., ‘Weak barrelledness for C (X) spaces’, J. Math. Anal. Appl. 297 (2004), 495–505.Google Scholar
Kąkol, J. and Śliwa, W., ‘Remarks concerning the separable quotient problem’, Note Mat. XIII (1993), 277–282.Google Scholar
Köthe, G., Topological Vector Spaces I (Springer, New York, 1969).Google Scholar
Lehner, W., Über die Bedeutung gewisser Varianten des Baire’schen Kategorienbegriffs für die Funktionenräume C c(T), Dissertation (Ludwig Maximilians University, Munich, 1979).Google Scholar
Morris, P. D. and Wulbert, D. E., ‘Functional representations of topological algebras’, Pacific J. Math. 22 (1967), 323–337.Google Scholar
Popov, M. M., ‘On the codimension of subspaces of L p(μ), 0 < p < 1’, Funkt. Anal. Prilozen 18 (1984), 94–95 (in Russian).Google Scholar
Robertson, W. J., ‘On properly separable quotients of strict (LF) spaces’, J. Aust. Math. Soc. 47 (1989), 307–312.Google Scholar
Rosenthal, H. P., ‘On quasi-complemented subspaces of Banach spaces, with an appendix on compactness of operators from ℓ p(μ) to ℓ r(ν)’, J. Funct. Anal. 4 (1969), 176–214.CrossRefGoogle Scholar
Saxon, S. A., ‘Nuclear and product spaces, Baire-like spaces, and the strongest locally convex topology’, Math. Ann. 197 (1972), 87–106.CrossRefGoogle Scholar
Saxon, S. A., ‘Every countable-codimensional subspace of an infinite-dimensional [non-normable] Fréchet space has an infinite-dimensional Fréchet quotient [isomorphic to ω ]’, Bull. Pol. Acad. Sci. 39 (1991), 161–166.Google Scholar
Saxon, S. A., ‘All separable Banach spaces are quotients of any countable-codimensional subspace of ℓ 1’, Bull. Pol. Acad. Sci. 39 (1991), 167–173.Google Scholar
Saxon, S. A. and Levin, M., ‘Every countable-codimensional subspace of a barrelled space is barrelled’, Proc. Amer. Math. Soc. 29 (1971), 91–96.CrossRefGoogle Scholar
Saxon, S. A. and Narayanaswami, P. P., ‘Metrizable (L F)-spaces, (d b)-spaces and the separable quotient problem’, Bull. Aust. Math. Soc. 23 (1981), 65–80.CrossRefGoogle Scholar
Saxon, S. A. and Narayanaswami, P. P., ‘(L F)-spaces, quasi-Baire spaces, and the strongest locally convex topology’, Math. Ann. 274 (1986), 627–641.Google Scholar
Saxon, S. A. and Sánchez Ruiz, L. M., ‘Dual local completeness’, Proc. Amer. Math. Soc. 125 (1997), 1063–1070.Google Scholar
Saxon, S. A. and Tweddle, I., ‘The fit and flat components of barrelled spaces’, Bull. Aust. Math. Soc. 51 (1995), 521–528.Google Scholar
Saxon, S. A. and Wilansky, A., ‘The equivalence of some Banach space problems’, Colloq. Math. XXXVII (1977), 217–226.Google Scholar
Schaefer, H. H., Topological Vector Spaces (Springer, New York, 1971).CrossRefGoogle Scholar
Tweddle, I. and Yeomans, F. E., ‘On the stability of barrelled topologies II’, Glasg. Math. J. 21 (1980), 91–95.Google Scholar
Warner, S., ‘The topology of compact convergence on continuous function spaces’, Duke Math. J. 25 (1958), 265–282.Google Scholar
Wilansky, A., Modern Methods in Topological Vector Spaces (McGraw-Hill, New York, 1978).Google Scholar