Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-27T23:06:54.551Z Has data issue: false hasContentIssue false
  • English
  • Français

ARITHMETIC PROPERTIES OF PARTITION QUADRUPLES WITH ODD PARTS DISTINCT

Part of: Combinatorics Additive number theory; partitions

Published online by Cambridge University Press:  08 July 2015

LIUQUAN WANG
LIUQUAN WANG*
Affiliation:
Department of Mathematics, National University of Singapore, Singapore 119076, Singapore email [email protected], [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let $\text{pod}_{-4}(n)$ denote the number of partition quadruples of $n$ where the odd parts in each partition are distinct. We find many arithmetic properties of 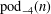$\text{pod}_{-4}(n)$ including the following infinite family of congruences: for any integers 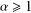${\it\alpha}\geq 1$ and $n\geq 0$,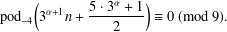

$$\begin{eqnarray}\text{pod}_{-4}\biggl(3^{{\it\alpha}+1}n+\frac{5\cdot 3^{{\it\alpha}}+1}{2}\biggr)\equiv 0~(\text{mod}~9).\end{eqnarray}$$
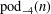We also establish some internal congruences and some Ramanujan-type congruences modulo 2, 5 and 8 satisfied by $\text{pod}_{-4}(n)$.

Keywords

congruencespartition quadruplesdistinct odd partstheta functions

MSC classification

Primary: 05A17: Partitions of integers
Secondary: 11P83: Partitions; congruences and congruential restrictions
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 92 , Issue 3 , December 2015 , pp. 353 - 364
Copyright
© 2015 Australian Mathematical Publishing Association Inc. 

References

Berndt, B. C., Number Theory in the Spirit of Ramanujan (American Mathematical Society, Providence, RI, 2006).CrossRefGoogle Scholar
Chen, W. Y. C. and Lin, B. L. S., ‘Congruences for bipartitions with odd parts distinct’, Ramanujan J. 25(2) (2011), 277–293.CrossRefGoogle Scholar
Hirschhorn, M. D. and Sellers, J. A., ‘Arithmetic properties of partitions with odd parts distinct’, Ramanujan J. 22 (2010), 273–284.CrossRefGoogle Scholar
Radu, S. and Sellers, J. A., ‘Congruence properties modulo 5 and 7 for the pod function’, Int. J. Number Theory 07 (2011), 2249–2259.CrossRefGoogle Scholar
Toh, P. C., ‘Ramanujan type identities and congruences for partition pairs’, Discrete Math. 312 (2012), 1244–1250.CrossRefGoogle Scholar
Wang, L., ‘Arithmetic properties of partition triples with odd parts distinct’, Int. J. Number Theory, to appear. arXiv:1407.5433.Google Scholar
Wang, L., ‘New congruences for partitions where the odd parts are distinct’, J. Integer Seq. (2015), article 15.4.2.Google Scholar