No CrossRef data available.
Article contents
Analytic iterations on Riemann surfaces
Published online by Cambridge University Press: 17 April 2009
Abstract
A complex analytic family of mappings P → M(α, P) from an abstract Riemann surface (analytic manifold) into itself is studied. The mapping M(α, P) is assumed to satisfy in local coordinates the autonomous differential equation  = L(w), and the condition M(O, P) = P. Under certain assumptions of regularity of the reciprocal differential L in a domain D ⊂ S, we prove that for every fixed α, ∣a∣ < α
= L(w), and the condition M(O, P) = P. Under certain assumptions of regularity of the reciprocal differential L in a domain D ⊂ S, we prove that for every fixed α, ∣a∣ < α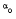 , the mapping M(α, P) is conformal and one to one in D. Moreover, it is shown that the family of mappings M(α, P) satisfies the iteration equation M[a, M(b, P)] = M(a + b, P) and hence is an analytic group (analytic iteration).
, the mapping M(α, P) is conformal and one to one in D. Moreover, it is shown that the family of mappings M(α, P) satisfies the iteration equation M[a, M(b, P)] = M(a + b, P) and hence is an analytic group (analytic iteration).
- Type
- Research Article
- Information
- Copyright
- Copyright © Australian Mathematical Society 1969


