Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-26T16:00:08.217Z Has data issue: false hasContentIssue false
  • English
  • Français

AN ANALOGUE OF HUPPERT’S CONJECTURE FOR CHARACTER CODEGREES

Part of: Representation theory of groups Graph theory

Published online by Cambridge University Press:  08 February 2021

A. BAHRI Open the ORCID record for A. BAHRI [Opens in a new window] ,
Z. AKHLAGHI Open the ORCID record for Z. AKHLAGHI [Opens in a new window]  and
B. KHOSRAVI Open the ORCID record for B. KHOSRAVI [Opens in a new window]
A. BAHRI
Affiliation:
Faculty of Mathematics and Computer Science, Amirkabir University of Technology (Tehran Polytechnic), 15914Tehran, Iran e-mail: [email protected]
Z. AKHLAGHI*
Affiliation:
Faculty of Mathematics and Computer Science, Amirkabir University of Technology (Tehran Polytechnic), 15914Tehran, Iran and School of Mathematics, Institute for Research in Fundamental Science (IPM), PO Box 19395-5746, Tehran, Iran
B. KHOSRAVI
Affiliation:
Faculty of Mathematics and Computer Science, Amirkabir University of Technology (Tehran Polytechnic), 15914 Tehran, Iran e-mail: [email protected]
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

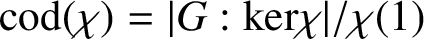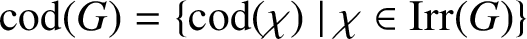Let G be a finite group, let ${\mathrm{Irr}}(G)$ be the set of all irreducible complex characters of G and let $\chi \in {\mathrm{Irr}}(G)$ . Define the codegrees, ${\mathrm{cod}}(\chi ) = |G: {\mathrm{ker}}\chi |/\chi (1)$ and ${\mathrm{cod}}(G) = \{{\mathrm{cod}}(\chi ) \mid \chi \in {\mathrm{Irr}}(G)\} $ . We show that the simple group ${\mathrm{PSL}}(2,q)$ , for a prime power 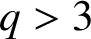$q>3$ , is uniquely determined by the set of its codegrees.

Keywords

characterisationcodegreesimple group

MSC classification

Primary: 20C15: Ordinary representations and characters
Secondary: 05C25: Graphs and abstract algebra (groups, rings, fields, etc.)
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 104 , Issue 2 , October 2021 , pp. 278 - 286
Check for updates
Copyright
© 2021 Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The research of the second author was in part supported by a grant from IPM (No. 99200028).

References

Ahanjideh, N., ‘Finite groups with the same conjugacy class sizes as a finite simple group’, Int. J. Group Theory 8(1) (2019), 23–33.Google Scholar
Akhlaghi, Z. and Khatami, M., ‘Improving Thompson’s conjecture for Suzuki groups’, Comm. Algebra 44 (2016), 3927–3932.CrossRefGoogle Scholar
Akhlaghi, Z., Le, T., Khatami, M., Moori, J. and Tong-Viet, H. P., ‘A dual version of Huppert’s conjecture on conjugacy class sizes’, J. Group Theory 18(1) (2015), 115–131.CrossRefGoogle Scholar
Alizadeh, F., Behravesh, H., Ghaffarzadeh, M., Ghasemi, M. and Hekmatara, S., ‘Groups with few codegrees of irreducible characters’, Comm. Algebra 47 (2019), 1147–1152.CrossRefGoogle Scholar
Casolo, C., ‘Some linear actions of finite groups with ${q}^{\prime }$ -orbits’, J. Group Theory 13(4) (2010), 503–534.CrossRefGoogle Scholar
Chillag, D., Mann, A. and Manz, O., ‘The co-degrees of irreducible characters’, Israel J. Math. 73(2) (1991), 207–223.CrossRefGoogle Scholar
Conway, J. H., Curtis, R. T., Norton, S. P., Parker, R. A. and Wilson, R. A., Atlas of Finite Groups (Oxford University Press, Oxford, 1985).Google Scholar
Dickson, L. E., Linear Groups: With an Exposition of the Galois Field Theory (Teubner, Leipzig, 1901).Google Scholar
Du, N. and Lewis, M. L., ‘Codegrees and nilpotence class of $p$ -groups’, J. Group Theory 19 (2016), 561–567.CrossRefGoogle Scholar
Isaacs, I. M., Character Theory of Finite Groups (Academic Press, New York, 1976).Google Scholar
Liu, Y. and Lu, Z. Q., ‘Nonsolvable ${D}_2$ -groups’, Acta Math. Sin. (Engl. Ser.) 31(11) (2015), 1683–1702.CrossRefGoogle Scholar
Malle, G. and Moretó, A., ‘Nonsolvable groups with few character degrees’, J. Algebra 294(1) (2005), 117–126.CrossRefGoogle Scholar
Qian, G., Wang, Y. and Wei, H., ‘Codegree of irreducible characters in finite groups’, J. Algebra 312 (2007), 946–955.CrossRefGoogle Scholar
Sayanjali, Z., Akhlaghi, Z. and Khosravi, B., ‘On the codegrees of finite groups’, Comm. Algebra 48(3) (2019), 1327–1332.CrossRefGoogle Scholar
White, D. L., ‘Character degrees of extensions of ${\rm PSL}\left(2,q\right)$ and ${\rm SL}\left(2,q\right)$ ’, J. Group Theory 16(1) (2013), 1–33.CrossRefGoogle Scholar