Article contents
THE ABSOLUTE 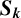 $\boldsymbol {S_k}$-MEASURE OF TOTALLY POSITIVE ALGEBRAIC INTEGERS
$\boldsymbol {S_k}$-MEASURE OF TOTALLY POSITIVE ALGEBRAIC INTEGERS
Published online by Cambridge University Press: 11 August 2022
Abstract
Let
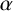 $\alpha $
be a totally positive algebraic integer of degree d, with conjugates
$\alpha $
be a totally positive algebraic integer of degree d, with conjugates
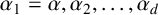 $\alpha _1=\alpha , \alpha _2, \ldots , \alpha _d$
. The absolute
$\alpha _1=\alpha , \alpha _2, \ldots , \alpha _d$
. The absolute
 $S_k$
-measure of
$S_k$
-measure of
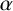 $\alpha $
is defined by
$\alpha $
is defined by
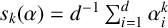 $s_k(\alpha )= d^{-1} \sum _{i=1}^{d}\alpha _i^k$
. We compute the lower bounds
$s_k(\alpha )= d^{-1} \sum _{i=1}^{d}\alpha _i^k$
. We compute the lower bounds
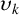 $\upsilon _k$
of
$\upsilon _k$
of
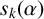 $s_k(\alpha )$
for each integer in the range
$s_k(\alpha )$
for each integer in the range
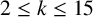 $2\leq k \leq 15$
and give a conjecture on the results for integers
$2\leq k \leq 15$
and give a conjecture on the results for integers
 $k>15$
. Then we derive the lower bounds of
$k>15$
. Then we derive the lower bounds of
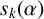 $s_k(\alpha )$
for all real numbers
$s_k(\alpha )$
for all real numbers
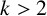 $k>2$
. Our computation is based on an improvement in the application of the LLL algorithm and analysis of the polynomials in the explicit auxiliary functions.
$k>2$
. Our computation is based on an improvement in the application of the LLL algorithm and analysis of the polynomials in the explicit auxiliary functions.
Keywords
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.
Footnotes
This work was supported in part by the National Natural Science Foundation of China (grant no. 12071375).
References
- 1
- Cited by



