Hostname: page-component-6587cd75c8-r56mn Total loading time: 0 Render date: 2025-04-23T15:30:20.505Z Has data issue: false hasContentIssue false
  • English
  • Français

A NOTE ON REGULAR SETS IN CAYLEY GRAPHS

Part of: Theory of error-correcting codes and error-detecting codes Algebraic combinatorics Graph theory

Published online by Cambridge University Press:  09 February 2023

JUNYANG ZHANG Open the ORCID record for JUNYANG ZHANG [Opens in a new window]  and
YANHONG ZHU Open the ORCID record for YANHONG ZHU [Opens in a new window]
JUNYANG ZHANG
Affiliation:
School of Mathematical Sciences, Chongqing Normal University, Chongqing 401331, PR China e-mail: [email protected]
YANHONG ZHU*
Affiliation:
School of Mathematical Sciences, Liaocheng University, Liaocheng 252000, PR China
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A subset R of the vertex set of a graph 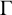$\Gamma $ is said to be 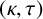$(\kappa ,\tau )$-regular if R induces a 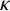$\kappa $-regular subgraph and every vertex outside R is adjacent to exactly 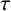$\tau $ vertices in R. In particular, if R is a 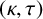$(\kappa ,\tau )$-regular set of some Cayley graph on a finite group G, then R is called a 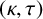$(\kappa ,\tau )$-regular set of G. Let H be a nontrivial normal subgroup of G, and 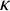$\kappa $ and $\tau $ a pair of integers satisfying 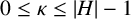$0\leq \kappa \leq |H|-1$, 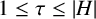$1\leq \tau \leq |H|$ and 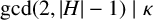$\gcd (2,|H|-1)\mid \kappa $. 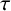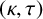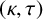It is proved that (i) if $\tau $ is even, then H is a $(\kappa ,\tau )$-regular set of G; (ii) if $\tau $ is odd, then H is a $(\kappa ,\tau )$-regular set of G if and only if it is a 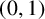$(0,1)$-regular set of G.

Keywords

regular setperfect codeCayley graphfinite group

MSC classification

Primary: 05C25: Graphs and abstract algebra (groups, rings, fields, etc.)
Secondary: 05E18: Group actions on combinatorial structures 94B25: Combinatorial codes
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 109 , Issue 1 , February 2024 , pp. 1 - 5
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

Footnotes

The first author was supported by the Natural Science Foundation of Chongqing (CSTB2022NSCQ- MSX1054) and the Foundation of Chongqing Normal University (21XLB006).

References

Anđelić, M., Cardoso, D. M. and Simić, S. K., ‘Relations between $\left(\kappa, \tau \right)$ -regular sets and star complements’, Czechoslovak Math. J. 63(138) (2013), 73–90.CrossRefGoogle Scholar
Cardoso, D. M., ‘An overview of $\left(\kappa, \tau \right)$ -regular sets and their applications’, Discrete Appl. Math. 269 (2019), 2–10.CrossRefGoogle Scholar
Cardoso, D. M. and Rama, P., ‘Equitable bipartitions of graphs and related results’, J. Math. Sci. 120(1) (2004), 869–880.CrossRefGoogle Scholar
Cardoso, D. M. and Rama, P., ‘Spectral results on graphs with regularity constraints’, Linear Algebra Appl. 423 (2007), 90–98.CrossRefGoogle Scholar
Cardoso, D. M., Sciriha, I. and Zerafa, C., ‘Main eigenvalues and $\left(\kappa, \tau \right)$ -regular sets’, Linear Algebra Appl. 423 (2010), 2399–2408.CrossRefGoogle Scholar
Green, H. M. and Liebeck, M. W., ‘Some codes in symmetric and linear groups’, Discrete Math. 343(8) (2020), Article no. 111719.CrossRefGoogle Scholar
Huang, H., Xia, B. Z. and Zhou, S. M., ‘Perfect codes in Cayley graphs’, SIAM J. Discrete Math. 32 (2018), 548–559.CrossRefGoogle Scholar
Wang, Y., Xia, B. Z. and Zhou, S. M., ‘Subgroup regular sets in Cayley graphs’, Discrete Math. 345(11) (2022), Article no. 113023.CrossRefGoogle Scholar
Wang, Y., Xia, B. Z. and Zhou, S. M., ‘Regular sets in Cayley graphs’, J. Algebr. Comb., to appear. Published online (26 October 2022).CrossRefGoogle Scholar