NONRELATIVISTIC LIMIT FOR THE TRAVELLING WAVES OF THE PSEUDORELATIVISTIC HARTREE EQUATION

Abstract

We consider the pseudorelativistic Hartree equation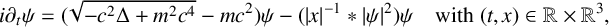

$$ \begin{align*} i\partial_t\psi=(\sqrt{-c^2\Delta +m^2c^4}-mc^2)\psi-(|x|^{-1}*|\psi|^2)\psi\quad \text{with } (t,x)\in\mathbb{R}\times\mathbb{R}^3, \end{align*} $$

which describes the dynamics of pseudorelativistic boson stars in the mean-field limit. We study the travelling waves of the form 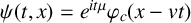$\psi (t,x)=e^{it\mu }\varphi _{c}(x-vt)$, where 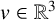$v\in \mathbb {R}^3$ denotes the travelling velocity. 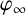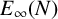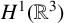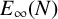We prove that $\varphi _{c}$ converges strongly to the minimiser $\varphi _{\infty }$ of the limit energy $E_{\infty }(N)$ in $H^1(\mathbb {R}^3)$ as the light speed $c\to \infty $, where $E_{\infty }(N)$ is the corresponding energy for the limit equation

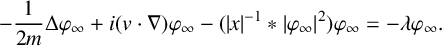$$ \begin{align*} -\frac{1}{2m}\Delta\varphi_{\infty}+i(v\cdot\nabla)\varphi_{\infty}-({|x|^{-1}}*|\varphi_{\infty}|^2)\varphi_{\infty}=-\lambda\varphi_{\infty}. \end{align*} $$

Since the operator 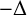$-\Delta $ is the classical kinetic operator, we call this the nonrelativistic limit. We prove the existence of the minimiser for the limit energy $E_{\infty }(N)$ by using concentration-compactness arguments.