Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-30T16:58:15.104Z Has data issue: false hasContentIssue false
  • English
  • Français

The Stable core

Published online by Cambridge University Press:  15 January 2014

Sy-david Friedman
Sy-david Friedman*
Affiliation:
Kurt Gödel Research Center, Währinger Strasse 22, A-1090 Vienna, AustriaE-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Vopěnka proved long ago that every set of ordinals is set-generic over HOD, Gödel's inner model of hereditarily ordinal-definable sets. Here we show that the entire universe V is class-generic over (HOD,S), and indeed over the even smaller inner model 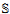=(L[S],S), where S is the Stability predicate. We refer to the inner model 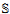as the Stable Core of V. The predicate S has a simple definition which is more absolute than any definition of HOD; in particular, it is possible to add reals which are not set-generic but preserve the Stable Core (this is not possible for HOD by Vopěnka's theorem).

Type
Research Article
Information
Bulletin of Symbolic Logic , Volume 18 , Issue 2 , June 2012 , pp. 261 - 267
Copyright
Copyright © Association for Symbolic Logic 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Friedman, S., Fine structure and class forcing , de Gruyter Series in Logic and its Applications, vol. 3, de Gruyter, 2000.CrossRefGoogle Scholar
[2] Vopěnka, P. and Hájek, P., The theory of semisets, Academia (Publishing house of the Czech Academy of Sciences), Prague, 1972.Google Scholar