Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-12-03T19:05:04.313Z Has data issue: false hasContentIssue false
  • English
  • Français

Optimal Proofs of Determinacy

Published online by Cambridge University Press:  15 January 2014

Itay Neeman
Itay Neeman*
Affiliation:
Department of Mathematics, University of California, Los Angeles, CA 90024. E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Extract

In this paper I shall present a method for proving determinacy from large cardinals which, in many cases, seems to yield optimal results. One of the main applications extends theorems of Martin, Steel and Woodin about determinacy within the projective hierarchy. The method can also be used to give a new proof of Woodin's theorem about determinacy in L(ℝ).

The reason we look for optimal determinacy proofs is not only vanity. Such proofs serve to tighten the connection between large cardinals and descriptive set theory, letting us bring our knowledge of one subject to bear on the other, and thus increasing our understanding of both. A classic example of this is the Harrington-Martin proof that -determinacy implies 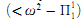-determinacy. This is an example of a transfer theorem, which assumes a certain determinacy hypothesis and proves a stronger one. While the statement of the theorem makes no mention of large cardinals, its proof goes through 0#, first proving that

-determinacy ⇒ 0# exists,

and then that

0# exists ⇒ 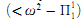-determinacy

More recent examples of the connection between large cardinals and descriptive set theory include Steel's proof that

ADL(ℝ) ⇒ HODL(ℝ) ⊨ GCH,

see [9], and several results of Woodin about models of AD+, a strengthening of the axiom of determinacy AD which Woodin has introduced. These proofs not only use large cardinals, but also reveal a deep, structural connection between descriptive set theoretic notions and notions related to large cardinals.

Type
Research Article
Information
Bulletin of Symbolic Logic , Volume 1 , Issue 3 , June 1995 , pp. 327 - 339
Copyright
Copyright © Association for Symbolic Logic 1995

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Harrington, Leo, Analytic determinacy and 0# , The Journal of Symbolic Logic, vol. 43 (1978), pp. 685–693.Google Scholar
[2] Kechris, A. S. and Woodin, W. H., The equivalence of partition properties and determinacy, Proceedings of the National Academy od Sciences, vol. 80 (1983), pp. 1783–1786.Google Scholar
[3] Martin, D. A., Proving determinacy, forthcoming.Google Scholar
[4] Martin, D. A., The largest countable this, that, and the other, Cabal Seminar 79–81 (Martin, D. A. Kechris, A. S. and Moschovakis, Y N., editors), Lecture Notes in Mathematics, no. 1019, Springer-Verlag, 1983, pp. 97–106.CrossRefGoogle Scholar
[5] Martin, D. A. and Steel, J. R., A proof of projective determinacy, Journal of the American Mathematical Society, vol. 2 (1989), pp. 71–125.Google Scholar
[6] Martin, D. A. and Steel, J. R., Iteration trees, Journal of the American Mathematical Society, vol. 7 (1994), pp. 1–73.Google Scholar
[7] Moschovakis, Y. N., Descriptive set theory, North Holland, Amsterdam, New York, Oxford, 1980.Google Scholar
[8] Steel, John, Inner models with many Woodin cardinals, Annals of Pure and Applied Logic, vol. 65 (1993), pp. 185–209.Google Scholar
[9] Steel, John, HOD L[ℝ] is a core model , this Bulletin, vol. 1 (1995), pp. 75–84.Google Scholar
[10] Woodin, W. H., unpublished.Google Scholar