Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-25T08:12:11.140Z Has data issue: false hasContentIssue false
  • English
  • Français

SELECTIVE WITHDRAWAL OF A TWO-LAYER VISCOUS FLUID

Published online by Cambridge University Press:  08 February 2013

JASON M. COSGROVE  and
LAWRENCE K. FORBES
JASON M. COSGROVE*
Affiliation:
School of Mathematics and Physics, University of Tasmania, Private Bag 37, Hobart, Tasmania 7001, Australia
LAWRENCE K. FORBES*
Affiliation:
School of Mathematics and Physics, University of Tasmania, Private Bag 37, Hobart, Tasmania 7001, Australia
*
✉[email protected]
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Selective withdrawal of a two-layer fluid is considered. The fluid layers are weakly compressible, miscible and viscous and therefore flow rotationally. The lower, denser fluid flows with constant velocity out through one or more drain holes in the bottom of a rectangular tank. The drain is opened impulsively and the subsequent draw-down produces waves in the interface which travel outward to the edges of the tank and are reflected back with a 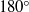$18{0}^{\circ } $ change of phase. The points on the interface that have the highest absolute gradient form regions of high vorticity in the tank, enabling mixing of the fluids. An inviscid linearized interface is computed and compared to contour plots of density for the viscous solution. The two agree closely at early times in the withdrawal process, but as time increases, nonlinear and viscous effects take over. The time at which the lighter fluid starts to flow out of the tank is dependent on the number of drains, their width, and the fluid flow rate and density, and is investigated here.

Keywords

selective withdrawalmultiple drainsviscous fluidsspectral methodsnonlinear effectstwo-layered fluids
Type
Research Article
Information
The ANZIAM Journal , Volume 53 , Issue 4 , April 2012 , pp. 253 - 277
Copyright
Copyright ©2013 Australian Mathematical Society

References

Ahmed, M., Hassan, I. and Esmail, N., “The onset of gas pull-through during dual discharge from a stratified two-phase region: theoretical analysis”, Phys. Fluids 16 (2004) 3385–3392; doi:10.1063/1.1771619.CrossRefGoogle Scholar
Farrow, D. E. and Hocking, G. C., “A numerical model for withdrawal from a two-layer fluid”, J. Fluid Mech. 549 (2006) 141–157; doi:10.1017/S0022112005007561.CrossRefGoogle Scholar
Forbes, L. K., “The Rayleigh–Taylor instability for inviscid and viscous fluids”, J. Engrg. Math. 65 (2009) 273–290; doi:10.1007/s10665-009-9288-9.CrossRefGoogle Scholar
Forbes, L. K. and Hocking, G. C., “Unsteady draining flows from a rectangular tank”, Phys. Fluids 19 (2007) 082104; doi:10.1063/1.2759891.CrossRefGoogle Scholar
Hocking, G. C., “Critical withdrawal from a two-layer fluid through a line sink”, J. Engrg. Math. 25 (1991) 1–11; doi:10.1007/BF00036598.CrossRefGoogle Scholar
Hocking, G. C. and Zhang, H., “A note on withdrawal from a two-layer fluid through a line sink in a porous medium”, ANZIAM J. 50 (2008) 101–110; doi:10.1017/S144618110800028X.CrossRefGoogle Scholar
Imberger, J. and Hamblin, P. F., “Dynamics of lakes, reservoirs and cooling ponds”, Annu. Rev. Fluid Mech. 14 (1982) 153–187; doi:10.1146/annurev.fl.14.010182.001101.CrossRefGoogle Scholar
Imberger, J., Thompson, R. and Fandry, C., “Selective withdrawal from a finite rectangular tank”, J. Fluid Mech. 78 (1976) 489–512; doi:10.1017/S0022112076002577.CrossRefGoogle Scholar
Lister, J. R., “Selective withdrawal from a viscous two-layer system”, J. Fluid Mech. 198 (1989) 231–254; doi:10.1017/S002211208900011X.CrossRefGoogle Scholar
Pope, S. B., Turbulent flows (Cambridge University Press, Cambridge, 2000).CrossRefGoogle Scholar
Stokes, T. E., Hocking, G. C. and Forbes, L. K., “Unsteady flow induced by a withdrawal point beneath a free surface”, ANZIAM J. 47 (2005) 185–202; doi:10.1017/S1446181100009986.CrossRefGoogle Scholar
Wood, I. R., “Selective withdrawal from a stably stratified fluid”, J. Fluid Mech. 32 (1968) 209–223; doi:10.1017/S0022112068000686.CrossRefGoogle Scholar