Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-27T15:42:38.603Z Has data issue: false hasContentIssue false
  • English
  • Français

Multiple-term approximations for Appell's F1 function

Published online by Cambridge University Press:  17 February 2009

Magdi A. El-Gabali
Magdi A. El-Gabali
Affiliation:
Kuwait University, Department of Mathematics and Computer Science, P.O. Box 5969 Safat 13060, Kuwait
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper computational issues of Appell's F1 function

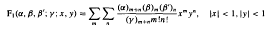
are addressed. A novel technique is used in the derivation of highly efficient multiple-term approximations of this function (including asymptotic ones). Simple structured single- and double-term approximations, as the first two candidates of this multiple-term representa-tion, are developed in closed form. Error analysis shows that- the developed algorithms are superior to existing approximations for the same number of terms. The formulation presented is highly efficient and could be extended to a wide class of special functions.

Type
Research Article
Information
The ANZIAM Journal , Volume 39 , Issue 1 , July 1997 , pp. 135 - 148
Copyright
Copyright © Australian Mathematical Society 1997

References

[1] Erdelyi, A., Higher transcendental functions, Volume 1 (McGraw-Hill, New York, 1953).Google Scholar
[2] Exton, H., Multiple hypergeometric functions and applications (Wiley & Sons, New York, 1976).Google Scholar
[3] El-Gabali, M., “Single term asymptotic approximations for the generalized elliptic-type integrals”, J. Revista de la Accidentia de Ciencias 6 (1994) 105–118.Google Scholar
[4] El-Gabali, M., “Efficient multiple term approximations for the generalized elliptic-type integrals”, J. Austral. Math. Soc. Ser. B 38 (1997) 411–126.CrossRefGoogle Scholar
[5] Galue, L., Kalla, S. and Leubner, C., “Algorithms for the approximation of the generalized Hubbell rectangular source integrals”, Radiat. Phys. Chem. 43 (1994) 497–502.CrossRefGoogle Scholar
[6] Galue, L. and Kiryakova, V., “Further results on a family of generalized radiation integrals”, Radiat. Phys. Chem. 43 (1994) 573–579.CrossRefGoogle Scholar
[7] Glasser, M. L., “Solution of the problem 83 – 6 by J. H. Hubbell”, SIAM Rev. 26 (1984) 276–278.CrossRefGoogle Scholar
[8] Kalla, S. L., “The Hubbell rectangular source integral and its generalizations”, Radiat. Phys. Chem. 41 (1993) 775–781.CrossRefGoogle Scholar
[9] Kalla, S. L. and Galue, L., “Simple algorithms for the approximation of the hypergeometric functions”, J. Alg. Groups and Geom. 11 (1994) 347–359.Google Scholar
[10] Kalla, S. L., Leubner, C. and Hubbell, J. H., “Further results on generalized elliptic-type integrals”, Appl. Anal. 25 (1987) 269–274.CrossRefGoogle Scholar
[11] Kalla, S. L., Al-Sagabi, B. and Conde, S., “Some results related to radiation field problem”, Hadronic Journal 10 (1987) 221–230.Google Scholar
[12] Lebedev, N. N., Special functions and their applications (Dover Publications, New York, 1972).Google Scholar
[13] Mathai, A. M. and Saxena, R. A., Generalized hypergeometric functions with applications in Statistics and Physical Sciences, Volume 348 of Lecture notes in mathematics (Springer-Verlag, Berlin, 1973).Google Scholar
[14] Seaborn, J., Hypergeometric functions and their applications, Volume 1 (Springer-Verlag, New York, 1991).CrossRefGoogle Scholar
[15] Timus, D. M., “Some further results on the Hubbell rectangular source integral”, J. Comput. Appl. Math. 45 (1993) 331–338.CrossRefGoogle Scholar