Article contents
Stability Derivatives of Blunt Slender Cones at High Mach Numbers
Published online by Cambridge University Press: 07 June 2016
Summary
This paper presents a semi-empirical theoretical model for calculating the effect of nose bluntness on the stability derivatives of oscillating slender cones at hypersonic Mach numbers. It is based on a hybrid blast wave analogy/shock-expansion flow model and is used to obtain closed form analytic expressions for the derivatives 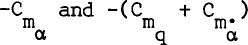 for oscillating slender cones. Two models based on zero thickness and finite thickness entropy layers are proposed which are seen to be appropriate to the cases of very small and large nose bluntnesses, respectively. The results are compared with new and existing experimental data and with the predictions of previous theoretical methods.
for oscillating slender cones. Two models based on zero thickness and finite thickness entropy layers are proposed which are seen to be appropriate to the cases of very small and large nose bluntnesses, respectively. The results are compared with new and existing experimental data and with the predictions of previous theoretical methods.
- Type
- Research Article
- Information
- Copyright
- Copyright © Royal Aeronautical Society. 1979
References
- 12
- Cited by


