Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Embrechts, P.
and
Klüppelberg, C.
1994.
Some Aspects of Insurance Mathematics.
Theory of Probability & Its Applications,
Vol. 38,
Issue. 2,
p.
262.
Klüppelberg, C.
and
Mikosch, T.
1995.
Delay in claim settlement and ruin probability approximations.
Scandinavian Actuarial Journal,
Vol. 1995,
Issue. 2,
p.
154.
Embrechts, Paul
1995.
Risk theory of the second and third kind.
Scandinavian Actuarial Journal,
Vol. 1995,
Issue. 1,
p.
35.
Cairns, Andrew J. G.
1995.
The Present Value of a Series of Cashflows: Convergence in a Random Environment.
ASTIN Bulletin,
Vol. 25,
Issue. 2,
p.
81.
Grübel, Rudolf
and
Pitts, Susan M
2000.
Statistical Aspects of Perpetuities.
Journal of Multivariate Analysis,
Vol. 75,
Issue. 1,
p.
143.
Embrechts, Paul
and
Walk, Harro
2000.
Recursive estimation of distributional fix-points.
Journal of Applied Probability,
Vol. 37,
Issue. 1,
p.
73.
Embrechts, Paul
and
Walk, Harro
2000.
Recursive estimation of distributional fix-points.
Journal of Applied Probability,
Vol. 37,
Issue. 1,
p.
73.
Pitts, Susan M.
2004.
Encyclopedia of Actuarial Science.
Brazauskas, Vytaras
2004.
Encyclopedia of Actuarial Science.
Silvestrov, Dmitrii S.
and
Teugels, Jozef L.
2004.
Limit theorems for mixed max–sum processes with renewal stopping.
The Annals of Applied Probability,
Vol. 14,
Issue. 4,
Pitts, Susan M.
2014.
Wiley StatsRef: Statistics Reference Online.
Brazauskas, Vytaras
2014.
Wiley StatsRef: Statistics Reference Online.
Castaño-Martínez, A.
Pigueiras, G.
and
Sordo, M.A.
2019.
On a family of risk measures based on largest claims.
Insurance: Mathematics and Economics,
Vol. 86,
Issue. ,
p.
92.
Artikis, Constantinos T.
and
Artikis, Panagiotis T.
2020.
Uniform contraction of a stochastic integral in strategic operations.
Journal of Interdisciplinary Mathematics,
Vol. 23,
Issue. 3,
p.
727.
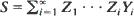 (‘perpetuity’) and
(‘perpetuity’) and 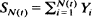 (‘aggregate claim amount’), where the (Yi), (Zi) are independent i.i.d. sequences and (N(t)) is a general point process, is a key question in insurance mathematics. In this paper, we show how suitably chosen metrics provide a theoretical justification for bootstrap estimation in these cases. In the perpetuity case, we also give a detailed discussion of how the method works in practice.
(‘aggregate claim amount’), where the (Yi), (Zi) are independent i.i.d. sequences and (N(t)) is a general point process, is a key question in insurance mathematics. In this paper, we show how suitably chosen metrics provide a theoretical justification for bootstrap estimation in these cases. In the perpetuity case, we also give a detailed discussion of how the method works in practice.

