Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-25T07:49:19.006Z Has data issue: false hasContentIssue false
  • English
  • Français

A flow conservation law for surface processes

Part of: Stochastic processes Geometric probability and stochastic geometry

Published online by Cambridge University Press:  01 July 2016

G. Last  and
R. Schassberger
G. Last*
Affiliation:
Technical University of Braunschweig
R. Schassberger*
Affiliation:
Technical University of Braunschweig
*
* Postal address for both authors: Institut für Mathematische Stochastik, Technische Universität Braunschweig, Pockelsstrasse 14, Postfach 3329, D-38106 Braunschweig, Germany.
* Postal address for both authors: Institut für Mathematische Stochastik, Technische Universität Braunschweig, Pockelsstrasse 14, Postfach 3329, D-38106 Braunschweig, Germany.
Get access Rights & Permissions [Opens in a new window]

Abstract

The object studied in this paper is a pair (Φ, Y), where Φ is a random surface in 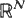and Y a random vector field on . The pair is jointly stationary, i.e. its distribution is invariant under translations. The vector field Y is smooth outside Φ but may have discontinuities on Φ. Gauss' divergence theorem is applied to derive a flow conservation law for Y. For 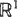this specializes to a well-known rate conservation law for point processes. As an application, relationships for the linear contact distribution of Φ are derived.

Keywords

RANDOM SURFACEGAUSS'DIVERGENCE THEOREMSTOCHASTIC GEOMETRYRANDOM MEASUREPALM PROBABILITYCONSERVATION LAWLINEAR CONTACT DISTRIBUTIONPOINT PROCESS

MSC classification

Primary: 60G57: Random measures
Secondary: 60D05: Geometric probability and stochastic geometry
Type
Stochastic Geometry amd Statistical Applications
Information
Advances in Applied Probability , Volume 28 , Issue 1 , March 1996 , pp. 13 - 28
Copyright
Copyright © Applied Probability Trust 1996 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Baccelli, F. and Brémaud, P. (1994) Elements of Queueing Theory. Springer, New York.Google Scholar
Bardhan, I. and Sigman, K. (1993) Rate conservation law for stationary semimartingales. Prob. Inf Eng. Sci. 7, 1–17.Google Scholar
Brémaud, P. (1991) An elementary proof of Sengupta's invariance relation and a remark on Miyazawa's conservation principle. J. Appl. Prob. 28, 950–954.Google Scholar
Federer, H. (1969) Geometric Measure Theory. Springer, Berlin.Google Scholar
Ferrandiz, J. M. and Lazar, A. (1991) Rate conservation for stationary processes. J. Appl. Prob. 28, 146–158.Google Scholar
Franken, P., König, D., Arndt, U. and Schmidt, V. (1981) Queues and Point Processes. Akademie, Berlin.Google Scholar
Hilliard, J. E. (1962) Specification and measurement of microstructural anisotropy. Trans. Metall. Soc. ?IM? 224, 1201–1211.Google Scholar
Kallenberg, O. (1983) Random Measures. Akademie, Berlin.Google Scholar
König, H. (1964) Ein einfacher Beweis des Integralsatzes von Gauß. Jahresbericht. DMV 66, 119–138.Google Scholar
Lang, S. (1985) Differential Manifolds. Springer, New York.Google Scholar
Matheron, G. (1975) Random Sets and Integral Geometry. Wiley, New York.Google Scholar
Mazumdar, R., Kannurpatti, R. and Rosenberg, C. (1990) On rate conservation law for non-stationary processes. J. Appl. Prob. 28, 762–770.Google Scholar
Mecke, J. (1967) Stationäre zufällige Maße auf lokalkompakten Abelschen Gruppen. Z. Wahrscheinlichkeitsth. 9, 36–58.Google Scholar
Mecke, J. (1981) Stereological formulas for manifold processes. Prob. Math. Statist. 2, 31–35.Google Scholar
Miyazawa, M. (1983) The derivation of invariance relations in complex queueing systems with stationary inputs. Adv. Appl. Prob. 15, 874–885.Google Scholar
Miyazawa, M. (1985) The intensity conservation law for queues with randomly changed service rate. J. Appl. Prob. 22, 408–418.Google Scholar
Miyazawa, M. (1994) Rate conservation laws: a survey. Queueing Systems 15, 1–58.Google Scholar
Neveu, J. (1977) Processus ponctuels. Lecture Notes in Mathematics 598. pp. 249–445. Springer, Berlin.Google Scholar
Nguyen, X. X. and Zessin, H. (1976) Punktprozesse mit Wechselwirkung. Z. Wahrscheinlichkeitsth. 37, 91–126.Google Scholar
Pohlmann, S., Mecke, J. and Stoyan, D. (1981) Stereological formulas for stationary surface processes. Math. Operat. Statist. 12, 429–440.Google Scholar
Rataj, J. (1993) Random distances and edge correction. Statistics 24, 377–385.Google Scholar
Saxl, I. (1993) Contact distances and random free paths. J. Microsc. 170, 53–64.CrossRefGoogle Scholar
Schmidt, V. and Serfozo, R. F. (1994) Campbell's formula and applications to queueing. In: Frontiers in Queueing: Models, Methods and Problems. ed. Dshalalow, J. H. CRC Press, Melbourne.Google Scholar
Serra, J. P. (1982) Image Analysis and Mathematical Morphology. Academic Press, London.Google Scholar
Simmons, G. F. (1963) Introduction to Topology and Modern Analysis. McGraw-Hill, New York.Google Scholar
Stoyan, D., Kendall, W. S. and Mecke, J. (1987) Stochastic Geometry and Its Applications. Akademie, Berlin.Google Scholar
Zahle, M. (1982) Random processes of Hausdorff rectifiable closed sets. Math. Nachr. 108, 49–72.Google Scholar
Zahle, U. (1984) Local interpretation of Palm distributions of surface measures. Math. Nachr. 119, 341–356.Google Scholar