Article contents
Prediction of level crossings for normal processes containing deterministic components
Published online by Cambridge University Press: 01 July 2016
Abstract
A level crossing predictor is a predictor process Y(t) which can be used to predict whether a specific process X(t) will cross a predetermined level or not. A natural criterion of how good a predictor is can be the probability that a crossing is detected a sufficient time ahead, and the number of times the predictor makes a false alarm.
Suppose the process X(t) consists of a deterministic part A(t) which can be calculated with sufficient accuracy, and a stochastic part Xe(t) which can be predicted by some statistically based predictor 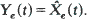 An example of this is the prediction of water level near a coast, when A(t) is a sum of known tide components.
An example of this is the prediction of water level near a coast, when A(t) is a sum of known tide components.
The paper develops a tool to handle the detection properties of such predictor processes when used to predict level crossings for the case when A(t) is periodic or is a sum of such functions.
- Type
- Research Article
- Information
- Copyright
- Copyright © Applied Probability Trust 1979
References
- 12
- Cited by


