Article contents
Asymptotic behavior of a generalization of Bailey's simple epidemic
Published online by Cambridge University Press: 01 July 2016
Extract
It has been shown that for many epidemic models, the stochastic theory leads to essentially the same results as the deterministic theory provided that one identifies mean values with the functions calculated from the deterministic differential equations (cf. [1]). If one considers a generalization of Bailey's simple epidemic for a fixed population of size N, represented schematically by 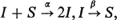 where I refers to an infected, S refers to a susceptible, and α and β are appropriate rate constants, then it is evident that at time t = ∞, the expected number of infected individuals must be zero provided that β > 0. If x(t) denotes the number of infected at time t, then the deterministic model is summarized by
where I refers to an infected, S refers to a susceptible, and α and β are appropriate rate constants, then it is evident that at time t = ∞, the expected number of infected individuals must be zero provided that β > 0. If x(t) denotes the number of infected at time t, then the deterministic model is summarized by

- Type
- III. Results on the General Stochastic Epidemic
- Information
- Copyright
- Copyright © Applied Probability Trust 1971
References
- 2
- Cited by


