Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-29T06:27:12.816Z Has data issue: false hasContentIssue false
  • English
  • Français

Multi-Symplectic Method for the Zakharov-Kuznetsov Equation

Published online by Cambridge University Press:  09 January 2015

Haochen Li ,
Jianqiang Sun  and
Mengzhao Qin
Haochen Li
Affiliation:
Department of Mathematics, College of Information Science and Technology, Hainan University, Hainan 570228, China School of Mathematical Science, Nanjing Normal University, Jiangsu 210023, China
Jianqiang Sun*
Affiliation:
Department of Mathematics, College of Information Science and Technology, Hainan University, Hainan 570228, China
Mengzhao Qin
Affiliation:
LSEC, Academy of Mathematics and System Sciences, Chinese Academy of Science, Beijing 100190, China
*
*Email:[email protected](J. Q. Sun)
Get access

Abstract

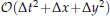A new scheme for the Zakharov-Kuznetsov (ZK) equation with the accuracy order of is proposed. The multi-symplectic conservation property of the new scheme is proved. The backward error analysis of the new multi-symplectic scheme is also implemented. The solitary wave evolution behaviors of the Zakharov-Kunetsov equation is investigated by the new multi-symplectic scheme. The accuracy of the scheme is analyzed.

Keywords

65D17The Zakharov-Kuznetsov equationmulti-symplectic methodbackward error analysis
Type
Research Article
Information
Advances in Applied Mathematics and Mechanics , Volume 7 , Issue 1 , February 2015 , pp. 58 - 73
Copyright
Copyright © Global Science Press Limited 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1]Ablowitz, M. J. and Schober, C. M., Hamiltonian integrators for the nonlinear Schrödinger equation, Int. J. Modern Phys. C, 5 (1994), pp. 397–401.CrossRefGoogle Scholar
[2]Bridges, T. J., Transverse instability of solitary-wave states of the water-wave problem, J. Fluid Mech., 439 (2001), pp. 255–278.CrossRefGoogle Scholar
[3]Bridges, T. J., Universal geometric condition for the transverse instability of solitary waves, Phys. Rev. Lett., 84 (2000), pp. 2614–2617.CrossRefGoogle ScholarPubMed
[4]Bridges, T. J., A geometric formulation of the conservation of wave action and its implications for signature and the classification of instabilities, Proc. Royal. Soc. London A, 453 (1997), pp. 1365–1395.CrossRefGoogle Scholar
[5]Bridges, T. J. and Reich, S., Multi-symplectic spectral discretizations for the Zakharov-Kuznetsov and shallow-water equations, Phys. D, 152 (2001), pp. 491–504.CrossRefGoogle Scholar
[6]Bridges, T. J. and Reich, S., Numerical methods for Hamiltonian PDEs, J. Phys. A Math. Gen., 39 (2006), pp. 5287–5320.CrossRefGoogle Scholar
[7]Bridges, T. J. and Reich, S., Multi-symplectic integrators: numerical schemes for Hamiltonian PDEs that conserve symplecticity, Phys. Lett. A, 284 (2001), pp. 184–193.CrossRefGoogle Scholar
[8]Blanes, S. and Moan, P. C., Splitting methods for the time-dependent Schrödinger equation, Phys. Lett. A, 265 (2000), pp. 35–42.CrossRefGoogle Scholar
[9]Chen, J. B., Multisymplectic geometry, local conservation laws and a multisymplectic integrator for the Zakharov-Kuznetsov equation, Lett. Math. Phys., 63 (2003), pp. 115–124.CrossRefGoogle Scholar
[10]Chen, J. B., A multisymplectic integrator for the periodic nonlinear Schrodinger equation, Appl. Math. Comput., 170 (2005), pp. 1394–1417.Google Scholar
[11]Feng, K., Collected Works of Feng Kang II, National Defence Industry Press, Beijing, 1995.Google Scholar
[12]Frank, J., Moore, B. and Reich, S., Linear PDEs and numerical mehods that preserve a multi-symplectic conservation law, SIAM J. Sci. Comput., 28 (2006), pp. 260–277.CrossRefGoogle Scholar
[13]Infeld, E., Self-focusing of nonlinear ion acoustic waves and solitons in magnetized plasmas, J. Plasma Phys., 33 (1985), pp. 171–182.CrossRefGoogle Scholar
[14]Infeld, E., Skorupski, A. A. and Senatorski, A., Dynamics of waves and multidimensional solitons of the Zakharov-Kuznetsov equation, J. Plasma Phys., 64 (2000), pp. 397–409.CrossRefGoogle Scholar
[15]Iwasaki, S., Toh, and Kawahara, T., Cylindrical qusi-solitons to the Zakharov-Kuznetsov equation, Phys. D, 43 (1990), pp. 293–303.CrossRefGoogle Scholar
[16]Kuznetsov, E. A., Rubenchik, A. M. and Zakharov, V. E., Soliton stability in plasmas and hydrodynamics, Phys. Rep., 142 (1986), pp. 103–165.CrossRefGoogle Scholar
[17]Kong, L. H., Hong, J. L. and Zhang, J. J., Splitting multisymplectic integrators for Maxwell’s equations, J. Comput. Phys., 229 (2010), pp. 4259–4278.CrossRefGoogle Scholar
[18]Aydin, A. and Karasozen, B., Multisymplectic box scheme for the complex modified Kortewegde Vries equation, J. Math. Phys., 51 (2010), 083511.CrossRefGoogle Scholar
[19]Moore, B. and Reich, S., Backward error analysis for multi-symplectic integration methods, Nu-merische Mathematik, 95 (2003), pp. 625–652.CrossRefGoogle Scholar
[20]Marsden, J. E., Patrick, G. P. and Shkoller, S., Multi-symplectic geometry, variational integrators, and nonlinear PDEs, Commun. Math. Phys., 199 (1999), pp. 351–395.CrossRefGoogle Scholar
[21]Nozaki, K., Vortex solitons of drift waves and anomalous diffusion, Phys. Rev. Lett., 46 (1981), pp. 184–187.CrossRefGoogle Scholar
[22]Petviashvili, V. I., Red spot of Jupiter and the drift soliton in a plasma, JETP Lett., 32 (1980), pp. 619–622.Google Scholar
[23]Reich, S., Backward error analysis for numerical integrators, SIAM J. Numer. Anal., 36 (1999), 1549.CrossRefGoogle Scholar
[24]Sun, J. Q. and Qin, M. Z., Multi-symplectic methods for the coupled 1D nonlinear Schrödinger system, Comput. Phys. Commun., 155 (2003), pp. 221–235.CrossRefGoogle Scholar
[25]Wang, Y. S., Wang, B. and Chen, X., Multi-symplectic Euler-box scheme for the KdV equation, China Phys. Lett., 24 (2007), pp. 312–314.Google Scholar
[26]Zakharov, V. E. and Kuznetsov, E. A., Three-dimensional solitons, Soviet. Phys., 39 (1974), pp. 285–286.Google Scholar