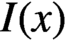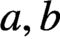Let R be a semiprime ring with extended centroid C and let $I(x)$ denote the set of all inner inverses of a regular element x in R. Given two regular elements $a, b$ in R, we characterise the existence of some 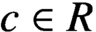$c\in R$ such that $I(a)+I(b)=I(c)$ . Precisely, if $a, b, a+b$ are regular elements of R and a and b are parallel summable with the parallel sum ${\cal P}(a, b)$ , then $I(a)+I(b)=I({\cal P}(a, b))$ . Conversely, if 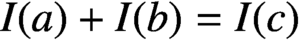$I(a)+I(b)=I(c)$ for some 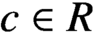$c\in R$ , then $\mathrm {E}[c]a(a+b)^{-}b$ is invariant for all 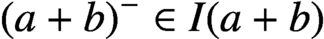$(a+b)^{-}\in I(a+b)$ , where 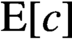$\mathrm {E}[c]$ is the smallest idempotent in C satisfying 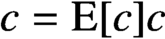$c=\mathrm {E}[c]c$ . This extends earlier work of Mitra and Odell for matrix rings over a field and Hartwig for prime regular rings with unity and some recent results proved by Alahmadi et al. [‘Invariance and parallel sums’, Bull. Math. Sci.10(1) (2020), 2050001, 8 pages] concerning the parallel summability of unital prime rings and abelian regular rings.

We study properties of the Drazin index of regular elements in a ring with a unity 1. We give expressions for generalized inverses of 1−ba in terms of generalized inverses of 1−ab. In our development we prove that the Drazin index of 1−ba is equal to the Drazin index of 1−ab.