Let f be a non-CM Hecke eigencusp form of level 1 and fixed weight, and let 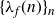$\{\lambda_f(n)\}_n$ be its sequence of normalised Fourier coefficients. We show that if 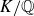$K/ \mathbb{Q}$ is any number field, and 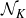$\mathcal{N}_K$ denotes the collection of integers representable as norms of integral ideals of K, then a positive proportion of the positive integers 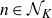$n \in \mathcal{N}_K$ yield a sign change for the sequence $\{\lambda_f(n)\}_{n \in \mathcal{N}_K}$. 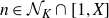More precisely, for a positive proportion of $n \in \mathcal{N}_K \cap [1,X]$ we have 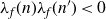$\lambda_f(n)\lambda_f(n') < 0$, where n′ is the first element of 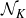$\mathcal{N}_K$ greater than n for which 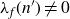$\lambda_f(n') \neq 0$.

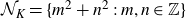For example, for $K = \mathbb{Q}(i)$ and $\mathcal{N}_K = \{m^2+n^2 \;:\; m,n \in \mathbb{Z}\}$ the set of sums of two squares, we obtain 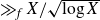$\gg_f X/\sqrt{\log X}$ such sign changes, which is best possible (up to the implicit constant) and improves upon work of Banerjee and Pandey. Our proof relies on recent work of Matomäki and Radziwiłł on sparsely-supported multiplicative functions, together with some technical refinements of their results due to the author.

In a related vein, we also consider the question of sign changes along shifted sums of two squares, for which multiplicative techniques do not directly apply. Using estimates for shifted convolution sums among other techniques, we establish that for any fixed 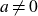$a \neq 0$ there are 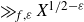$\gg_{f,\varepsilon} X^{1/2-\varepsilon}$ sign changes for 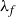$\lambda_f$ along the sequence of integers of the form 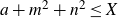$a + m^2 + n^2 \leq X$.

In this paper, we obtain a precise formula for the one-level density of L-functions attached to non-Galois cubic Dedekind zeta functions. We find a secondary term which is unique to this context, in the sense that no lower-order term of this shape has appeared in previously studied families. The presence of this new term allows us to deduce an omega result for cubic field counting functions, under the assumption of the Generalised Riemann Hypothesis. We also investigate the associated L-functions Ratios Conjecture and find that it does not predict this new lower-order term. Taking into account the secondary term in Roberts’s conjecture, we refine the Ratios Conjecture to one which captures this new term. Finally, we show that any improvement in the exponent of the error term of the recent Bhargava–Taniguchi–Thorne cubic field counting estimate would imply that the best possible error term in the refined Ratios Conjecture is $O_\varepsilon (X^{-\frac 13+\varepsilon })$ . This is in opposition with all previously studied families in which the expected error in the Ratios Conjecture prediction for the one-level density is $O_\varepsilon (X^{-\frac 12+\varepsilon })$ .

Stark conjectured that for any $h\in \Bbb {N}$ , there are only finitely many CM-fields with class number h. Let $\mathcal {C}$ be the class of number fields L for which L has an almost normal subfield K such that $L/K$ has solvable Galois closure. We prove Stark’s conjecture for $L\in \mathcal {C}$ of degree greater than or equal to 6. Moreover, we show that the generalised Brauer–Siegel conjecture is true for asymptotically good towers of number fields $L\in \mathcal {C}$ and asymptotically bad families of $L\in \mathcal {C}$ .

We break the convexity bound in the t-aspect for L-functions attached to cusp forms f for GL2(k) over arbitrary number fields k. The argument uses asymptotics with error term with a power saving, for second integral moments over spectral families of twists L(s,f⊗χ) by Grossencharacters χ, from our previous paper on integral moments.

We obtain second integral moments of automorphic L-functions on adele groups GL2 over arbitrary number fields, by a spectral decomposition using the structure and representation theory of adele groups GL1 and GL2. This requires reformulation of the notion of Poincaré series, replacing the collection of classical Poincaré series over GL2(ℚ) or GL2(ℚ(i)) with a single, coherent, global object that makes sense over a number field. This is the first expression of integral moments in adele-group terms, distinguishing global and local issues, and allowing uniform application to number fields. When specialized to the field of rational numbers ℚ, we recover the classical results on moments.

Let $K$ be a totally real number field of degree $n$. We show that the number of totally positive integers (or more generally the number of totally positive elements of a given fractional ideal) of given trace is evenly distributed around its expected value, which is obtained from geometric considerations. This result depends on unfolding an integral over a compact torus.

We construct a finite-dimensional vector space of functions of two complex variables attached to a smooth algebraic curve C over a finite field $\mathbb{F}_q$, q odd, and a level. These functions collect the analytic information about the cohomology of the curve and its quadratic twists that is encoded in the corresponding L-functions; they are double Dirichlet series in two independent complex variables s and w. We prove that these series satisfy a finite, non-abelian group of functional equations in the two complex variables (s, w) and are rational functions in q-s and q-w with a specified denominator. The group is D6, the dihedral group of order 12.