The usual proof of the adiabatic invariance of the quantum integrals of a multiply periodic dynamical system rests on the assumption that the Hamiltonian equations remain valid for the adiabatically changing system, with the same Hamiltonian function H, or at any rate with a new Hamiltonian function differing from the old one only by terms that vanish with ȧ, (a) being the varying parameter.

The dynamical problem of the “diatomic molecule” is solved on the new mechanics. The terms of the rotational energy are , where ; the weights of the corresponding states are 2m; the frequencies differ a little from the classical ones. Finally the intensities are slightly different from those computed by Kemble; the main term agrees with that of Fowler, but the positive branch is only slightly stronger than the negative. The central line vanishes. The intensities are valid only for the fundamental band.

It is well known that the calm which commonly comes on a cold clear night is due to the cooling effect of the ground on the lowest layers of the atmosphere. It frequently happens that the wind at a height of a few hundred feet does not die down at all at night; in fact observations taken at the top of the Eiffel tower show that there is frequently a slight increase in wind at that height. The cooling of the air near the ground makes the lower layers heavier than the higher layers and thus tends to prevent the formation of turbulence at the earth's surface. Since it is only through the medium of turbulence that the higher layers are able to drag the lowest layers over the ground the surface air stops moving.

A method has been devised by Dr Alex Müller for determining the orientation of a single-crystal of metal by photographic measurement of the reflection of characteristic X-rays from surface layers. The incident beam passes perpendicularly through an axis of rotation around which the crystal is turned until a reflection is obtained with one of the component wave-lengths of the X-rays.

New factors arise in a species by the process of mutation. The frequency of mutation is generally small, but it seems probable that it can sometimes be increased by changes in the environment (1,2). On the whole mutants recessive to the normal type occur more commonly than dominants. The frequency of a given type of mutation varies, but for some factors in Drosophila it must be less than 10−6, and is much less in some human cases. We shall first consider initial conditions, when only a few of the new type exist as the result of a single mutation; and then the course of events in a population where the new factor is present in such numbers as to be in no danger of extinction by mere bad luck. In the first section the treatment of Fisher (3) is followed.

The method described gives the differential energy distribution curve directly from readings of a galvanometer and a voltmeter. When adjusted, the arrangement is convenient in operation and very good “resolution” can be obtained. The existence of a maximum frequency of secondary electrons at a finite small energy has been established, and its position is shown to be independent of the energy of the primary beam over a fairly wide range. The effect of deposition of tungsten on a clean copper surface is studied with respect to the secondary emission properties of the surface. From these experiments some interesting conclusions concerning the mechanism of the process may be drawn.

I am very grateful to Prof. Sir Ernest Rutherford for the encouragement he has given and the interest he has taken in this work.

In the course of a long series of experiments on the scattering of positive rays I have had occasion to take some hundreds of photographs in which positive rays diverging from an approximately linear source pass through a metal slit about ·5 mm. wide parallel to the source and then strike the sensitive surface of the plate. The change caused by the actual impact of the rays can be developed like the ordinary latent image produced by light. The pattern thus formed on the negative is a rectangular strip about 1·8 mm. × 20 mm. answering in shape to the shape of the slit, and bordered by a much less intense edge (of the order 10%) due to the scattered rays whose investigation was the object of the experiment. It was found that, in a majority of the photographs, the rectangular strip, which should have been approximately uniform in blackness, at least across, had its longer edges apparently much blacker than its centre. So marked is this effect that it was supposed to be due to some cause affecting the distribution of the rays. However, careful measurements with a microphotometer showed that the effect was unreal, the edges always being less black than the centre. The effect of the positive rays is thus merely to produce a certain distribution of blackening on the plate, and the increased blackness seen at the edges is purely subjective.

The existence of complex compounds of metallic salts with ammonia was recognised in the early years of last century. Platinum in particular readily unites with ammonia, and the early chemists, when studying the general chemistry of ammonium platino-and platini-chlorides, soon discovered highly crystalline ammonia derivatives of platinum which contained an unusually high proportion of the metal. In the formulation of such compounds difficulties at once arose. Thus Peyrone in 1844 knew three distinct compounds of the composition PtCl2N2H6, a pale lemoncoloured compound known as the second chloride of Reiset, an orange-coloured compound discovered by Peyrone himself, and finally a dark green insoluble substance known as Magnus' green salt, discovered by the latter in 1828. The existence of these three compounds, now known as trans and cis dichloro-diaminoplatinum [Pt(NH3)2Cl2], and as tetramino-platinous platinochloride [Pt(NH3)4]PtCl4 respectively, could not be satisfactorily explained on current theories of salt formation. Similar compounds in which aliphatic and cyclic mono-amines replaced the ammonia groups were discovered later, but it was not until 1889 that Jörgensen introduced the use of the simplest stable aliphatic diamine, viz. ethylene diamine.

There are two effects involved in the sucking action of the diffusion pump. One is the penetration of gas molecules into the mercury vapour jet, and the second is the existence of a gradient of the partial pressure of the gas in the direction of the jet, so that the pressure at the origin of the jet is much lower than at the end of it.

An α-particle passing through a gas loses velocity because it gives up energy to the gaseous atoms. If we can calculate the average energy transferred to an atom, the stopping power of the gas follows immediately. The earlier theories, by Thomson and Darwin, took account of only the close collisions, in which the α-particle actually passes through the atom. Bohr, however, in 1913, took into account the transfers of energy to atoms at distances from the track considerably larger than atomic dimensions. His calculation was purely classical, and dealt with an atomic, model in which the electrons were capable of simple harmonic motions. The result was in very satisfactory agreement with experiment. For atoms containing each a single electron, with mass μ, charge ε, and natural period ω, Bohr's formula is

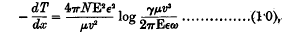
where − dT/dx is the rate of loss of energy by an α-particle whose charge is E, and velocity v; N is the number of atoms per c.c.; and γ = 1·123.

The experiments described in this paper were carried out in the University of Sydney during November 1924 and, owing to the short time which was available, are of a purely preliminary character. The following account is given as they are not likely to be resumed in the immediate future.

It is well known that charcoal possesses catalytic properties. It accelerates the decomposition of hydrogen peroxide, the removal of bromine from ββ-dibromo propionic acid and enables certain oxidations to take place in air at ordinary temperatures.

The problem of the two bodies has been treated on the new mechanics by Dirac, Pauli, and Schrödinger, who have independently derived the Balmer terms. The present paper is an attempt at a more complete solution. In particular, formulae are derived for the line intensities of the hydrogen spectrum, for the photoelectric effect and its inverse, and for the continuous absorption spectrum in the ultraviolet and in the X-ray regions. Also the probabilities of transition, deflection and capture are computed for the collision of an electron and an ion. Numerical values are only obtained, however, for the simplest line intensities. It is hoped to treat the problem in greater detail.

The usual method of calculating the probability of a switch between stationary states by the Quantum Mechanics is really equivalent to finding the intensity of the dipole radiation. Other switches of the system not involved in this radiation may be possible but are not taken account of by the calculation. A method of calculating the probability of switches due to radiation by the quadripole moment seems to be supplied by Dirac's recent theory of the interaction between matter and radiation in his paper “The Quantum Theory of Dispersion.” Equations from this paper will be denoted by a D. The results are probably of a purely theoretical interest and the intensities found probably too faint to be observed; but the present considerations do appear to emphasise the need for including the radiation in the exact theoretical treatment of a Quantum Mechanical system.

The β-ray type of disintegration is accompanied in general by two forms of radiation, the β-rays and the γ-rays. Radium E is noteworthy in that it gives a large amount of γ-radiation that it can only be detected by careful measurements. Yet, since it differs in this way from β-ray elements such as radium B and radium C, it is important for theoretical discussion that we should obtain as much knowledge as possible about this small amount of γ-radiation.

In a paper by Slater a new theory of radiation is discussed and the following explanation of the breadths of spectral lines is given: “An atom in the ith. state has a probability Pi of suffering in unit time a transition. Thus there is a probability Pi that the vibrations of each of the oscillators will simultaneously cease. But we shall assume that, in addition to this probability Pi of ceasing its oscillation altogether, each oscillator has also an independent probability Pj of suffering an interruption in which it ceases its oscillation as if it were leaving the state, but immediately begins again as if it were entering the same state” (i.e. with an arbitrary phase difference). “This term Pj is the same as the probability that an atom in the jth state will leave that state.” The total probability of interruption of vibration is thus (Pi + Pj) which is symmetrical with regard to the two end states. This makes the breadths of absorption and emission lines equal and so satisfies Kirchhoff's law.