The operator Im is defined as m-fold indefinite integration with zero constants of integration. The zero distribution of Im(p) for polynomials p is studied in general, and for two special classes of polynomials in detail. The main results are: (i) The zeros of In(Pn), where Pn(𝑧) is the n-th Legendre polynomial, converge to a certain algebraic curve; (ii) the zeros of 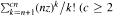 an integer) converge to pieces of a circle and of two "Szegö curves".
an integer) converge to pieces of a circle and of two "Szegö curves".