In this paper, we consider the family of nth degree polynomials whose coefficients form a log-convex sequence (up to binomial weights), and investigate their roots. We study, among others, the structure of the set of roots of such polynomials, showing that it is a closed convex cone in the upper half-plane, which covers its interior when n tends to infinity, and giving its precise description for every 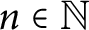$n\in \mathbb {N}$ , $n\geq 2$ . Dual Steiner polynomials of star bodies are a particular case of them, and so we derive, as a consequence, further properties for their roots.