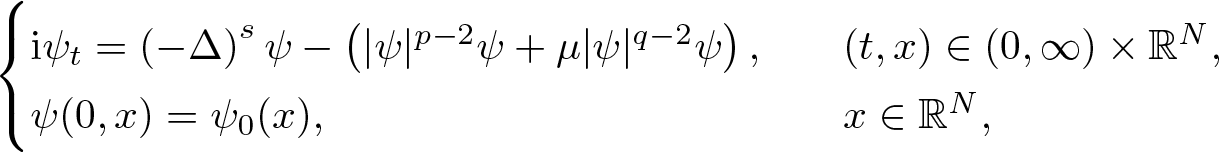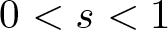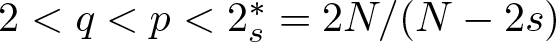1 results
Instability of standing waves for fractional NLS with combined nonlinearities
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics , First View
- Published online by Cambridge University Press:
- 27 November 2024, pp. 1-29
-
- Article
-
- You have access
- HTML
- Export citation
-
We study the existence and strong instability of standing wave solutions for the fractional nonlinear Schrödinger equation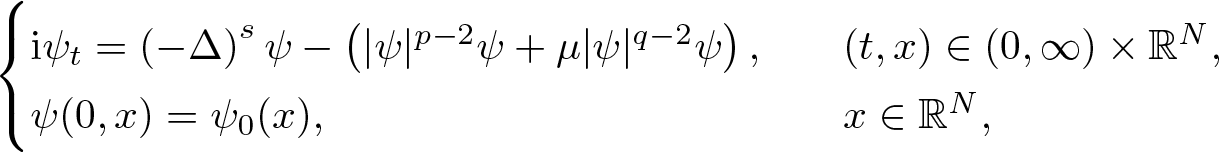
\begin{equation*}\begin{cases}\mathrm{i} \psi_{t}=\left(-\Delta\right)^s \psi-\left(|\psi|^{p-2}\psi+\mu|\psi|^{q-2}\psi\right), & \quad(t,x)\in(0,\infty)\times\mathbb{R}^N,\\\psi(0,x)=\psi_0(x),&\quad x\in\mathbb{R}^N,\end{cases}\end{equation*}where
$N\geq2$,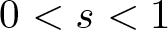 
$0 \lt s \lt 1$, 
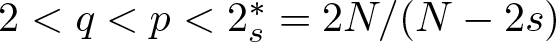$2 \lt q \lt p \lt 2_s^*=2N/(N-2s)$, and 
$\mu\in\mathbb{R}$. The primary challenge lies in the inhomogeneity of the nonlinearity.We deal with the following three cases: (i) for 
$2 \lt q \lt p \lt 2+4s/N$ and µ < 0, there exists a threshold mass a0 for the existence of the least energy normalized solution; (ii) for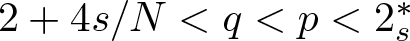 
$2+4s/N \lt q \lt p \lt 2_s^*$ and µ > 0, we reveal the existence of the ground state solution, explore the strong instability of standing waves, and provide a blow-up criterion; (iii) for 
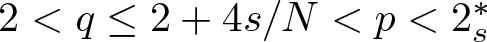$2 \lt q\leq2+4s/N \lt p \lt 2_s^*$ and µ < 0, the strong instability of standing wave solutions is demonstrated. These findings are illuminated through variational characterizations, the profile decomposition, and the virial estimate.
