In this paper, we compare queueing systems that differ only in their arrival processes, which are special forms of doubly stochastic Poisson (DSP) processes. We define a special form of stochastic dominance for DSP processes which is based on the well-known variability or convex ordering for random variables. For two DSP processes that satisfy our comparability condition in such a way that the first process is more ‘regular' than the second process, we show the following three results: (i) If the two systems are DSP/GI/1 queues, then 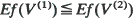 for all f increasing convex, with V(i), i = 1 and 2, representing the workload (virtual waiting time) in system. (ii) If the two systems are DSP/M(k)/1→ /M(k)/l ∞ ·· ·∞ /M(k)/1 tandem systems, with M(k) representing an exponential service time distribution with a rate that is increasing concave in the number of customers, k, present at the station, then
for all f increasing convex, with V(i), i = 1 and 2, representing the workload (virtual waiting time) in system. (ii) If the two systems are DSP/M(k)/1→ /M(k)/l ∞ ·· ·∞ /M(k)/1 tandem systems, with M(k) representing an exponential service time distribution with a rate that is increasing concave in the number of customers, k, present at the station, then 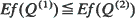 for all f increasing convex, with Q(i), i = 1 and 2, being the total number of customers in the two systems. (iii) If the two systems are DSP/M(k)/1/N systems, with N being the size of the buffer, then
for all f increasing convex, with Q(i), i = 1 and 2, being the total number of customers in the two systems. (iii) If the two systems are DSP/M(k)/1/N systems, with N being the size of the buffer, then  where
where 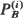 denotes the blocking (loss) probability of the two systems. A model considered before by Ross (1978) satisfies our comparability condition; a conjecture stated by him is shown to be true.
denotes the blocking (loss) probability of the two systems. A model considered before by Ross (1978) satisfies our comparability condition; a conjecture stated by him is shown to be true.

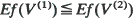 for all
for all 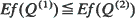 for all
for all  where
where 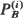 denotes the blocking (loss) probability of the two systems. A model considered before by Ross (1978) satisfies our comparability condition; a conjecture stated by him is shown to be true.
denotes the blocking (loss) probability of the two systems. A model considered before by Ross (1978) satisfies our comparability condition; a conjecture stated by him is shown to be true.