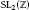Skip to main content Accessibility help

We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.

Close cookie message
×
  1. Home
  2. Search

Refine search

Refine search

Access:
Content type:
Publication date:
Apply   From and To filters
Subject: Show more
Journals Show more
Publishers: Show more
Societies Show more
Series: Show more
Collections: Show more

Actions for selected content:

Select all | Deselect all
  • View selected items
  • Export citations
  • Download PDF (zip)
  • Save to Kindle
  • Save to Dropbox
  • Save to Google Drive

Save Search

You can save your searches here and later view and run them again in "My saved searches".

Please provide a title, maximum of 40 characters.
Cancel
×

2 results

  • Equivariant Forms: Structure and Geometry

  • Abdelkrim Elbasraoui, Abdellah Sebbar
  • Journal:
    Canadian Mathematical Bulletin / Volume 56 / Issue 3 / 01 September 2013
    Published online by Cambridge University Press:
    20 November 2018, pp. 520-533
    Print publication:
    01 September 2013
    • Article
      • You have access
    • PDF
    • Export citation

  • In this paper we study the notion of equivariant forms introduced in the authors' previous works. In particular, we completely classify all the equivariant forms for a subgroup of $\text{S}{{\text{L}}_{2\left( \mathbb{Z} \right)}}$ by means of the cross-ratio, weight 2 modular forms, quasimodular forms, as well as differential forms of a Riemann surface and sections of a canonical line bundle.

  • CONFORMALLY INVARIANT ENERGIES OF KNOTS

  • R. Langevin, J. O’Hara
  • Journal:
    Journal of the Institute of Mathematics of Jussieu / Volume 4 / Issue 2 / April 2005
    Published online by Cambridge University Press:
    08 March 2005, pp. 219-280
    Print publication:
    April 2005

  • Conformally invariant functionals on the space of knots are introduced via extrinsic conformal geometry of knots and integral geometry on the space of spheres. Our functionals are expressed in terms of a complex-valued 2-form, which can be considered as the cross-ratio of a pair of infinitesimal segments of the knot. We show that our functionals detect the unknot as the total curvature does, and that their values explode if a knot degenerates to a singular knot with double points.

    AMS 2000 Mathematics subject classification: Primary 57M25; 53A30. Secondary 57R17