1 results
A note on the selection of random variables under a sum constraint
-
- Journal:
- Journal of Applied Probability / Volume 28 / Issue 4 / December 1991
- Published online by Cambridge University Press:
- 14 July 2016, pp. 919-923
- Print publication:
- December 1991
-
- Article
- Export citation
-
Consider an i.i.d. sequence of non-negative random variables (X1, · ··, Xn) with known distribution F. Consider decision rules for selecting a maximum number of the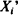
subject to the following constraints: (1) the sum of the elements selected must not exceed a given constant c > 0, and (2) the 
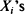must be inspected in strict sequence with the decision to accept or reject an element being final at the time it is inspected. Coffman et al. (1987) proved that there exists such a rule that maximizes the expected number En(c) of variables selected, and determined the asymptotics of En(c) for special distributions. Here we determine the asymptotics of En(cn) for very general choices of sequences (cn) and of F, by showing that En(c) is very close to an easily computable number. Our proofs are (somewhat deceptively) very simple, and rely on an appropriate stopping-time argument.
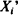subject to the following constraints: (1) the sum of the elements selected must not exceed a given constant 
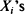must be inspected in strict sequence with the decision to accept or reject an element being final at the time it is inspected. Coffman et al. (1987) proved that there exists such a rule that maximizes the expected number 