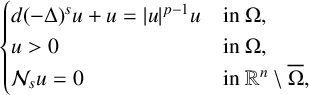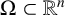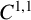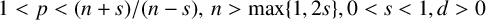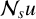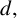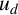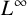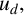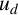ASYMPTOTIC BEHAVIOUR OF THE LEAST ENERGY SOLUTIONS TO FRACTIONAL NEUMANN PROBLEMS

Abstract

We study the asymptotic behaviour of the least energy solutions to the following class of nonlocal Neumann problems:

$$ \begin{align*} \begin{cases} { d(-\Delta)^{s}u+ u= \vert u\vert^{p-1}u } & \text{in } \Omega, \\ {u>0} & \text{in } \Omega, \\ { \mathcal{N}_{s}u=0 } & \text{in } \mathbb{R}^{n}\setminus \overline{\Omega}, \end{cases} \end{align*} $$

where $\Omega \subset \mathbb {R}^{n}$ is a bounded domain of class $C^{1,1}$, $1<p<({n+s})/({n-s}),\,n>\max \{1, 2s \}, 0<s<1, d>0$ and $\mathcal {N}_{s}u$ is the nonlocal Neumann derivative. We show that for small $d,$ the least energy solutions $u_d$ of the above problem achieve an $L^{\infty }$-bound independent of $d.$ Using this together with suitable $L^{r}$-estimates on $u_d,$ we show that the least energy solution $u_d$ achieves a maximum on the boundary of $\Omega $ for d sufficiently small.

1. Introduction

We discuss the asymptotic behaviour of nonconstant least energy solutions of the following problem:

(1-1) $$ \begin{align}\begin{cases} { d(-\Delta)^{s}u+ u= \vert u\vert^{p-1}u } &\text{in } \Omega, \\ {u>0} &\text{in } \Omega, \\ { \mathcal{N}_{s}u=0 } & \text{in } \mathcal{C} \overline{\Omega}, \end{cases}\end{align} $$

where $\Omega \subset \mathbb {R}^{n}$ is a bounded domain of class $C^{1,1},\,\,1<p<({n+s})/({n-s}), n>\max \{1, 2s \}, 0<s<1, d>0,\, \mathcal {C} \Omega := \mathbb {R}^n\setminus \Omega $ and $\mathcal {N}_{s}u$ is the nonlocal Neumann derivative, which is defined next. The nonlocal operator $(-\Delta )^{s}$ is called the fractional Laplacian, which is defined for smooth functions as follows:

(1-2) $$ \begin{align} (-\Delta)^{s}u(x)= c_{n,s}\,\mathrm{PV}\int_{\mathbb{R}^{n}}\frac{u(x)-u(y)}{\vert x-y\vert^{n+2s}}\,dy. \end{align} $$

Here, by PV, we mean the Cauchy principal value and $c_{n,s}$ is a normalising constant, given by

$$ \begin{align*} c_{n,s}=\bigg(\int_{\mathbb{R}^{n}} \frac{1-\text{cosx}_{1}}{\vert x\vert^{n+2s}}\,dx\bigg)^{-1}; \end{align*} $$

see for instance [12] for the details. Recently, Dipierro et al. [14] have introduced a new nonlocal Neumann condition $\mathcal {N}_{s}, $ which is defined as follows:

$$ \begin{align*} \mathcal{N}_{s}u(x):=c_{n,s}\int_{\Omega}\frac{u(x)-u(y)}{\vert x-y\vert^{n+2s}}\,dy, \quad x \in \mathcal{C} \overline{\Omega}. \end{align*} $$

The advantage of this nonlocal Neumann condition is that it has simple probabilistic interpretation and (1-1) has a variational structure. Further, it naturally arises from the superposition of Brownian and Lévy processes; see [16] for the details. We recall that $ \mathcal {N}_{s}u$ approaches the classical Neumann derivative $\partial _{\nu }u$ as s goes to $1.$

In the last few decades, mathematical analysis of biological phenomena has gained much attention. For example, chemotaxis models, which are also known as Keller–Segel models [28], have been widely studied in different directions in many papers; see [3, 24, 25] for a survey on this subject. Chemotaxis refers to the movement of cells or organisms in response to chemical gradients in their environment. The analysis on the steady-state for a chemotactic aggregation model with linear or logarithmic sensitivity function was thoroughly done in many papers; see for instance [27, 31, 35].

Let us point out that the following semilinear Neumann problem is an example of the Keller–Segel model with a logarithmic chemotactic sensitivity:

(1-3) $$ \begin{align}\begin{cases} {-d \Delta u+ u= \vert u\vert^{p-1}u } &\text{in } \Omega, \\ {u>0} &\text{in } \Omega, \\[3pt] {\dfrac{\partial u}{\partial \nu}=0 } &\text{on } \partial \Omega, \end{cases} \end{align} $$

where $d>0$ , $\Omega \subset \mathbb {R}^n$ is a bounded domain with smooth boundary and $1<p \leq ({n+2})/({n-2})$ if $n \geq 3$ and $1<p<\infty $ if $p=2$ ; see [31] for the details. Problem (1-3) admits a nonconstant solution for d sufficiently small; see [1, 30, 31]. Lin et al. [31] and Lin and Ni [30] established the existence of solutions to (1-3) in the subcritical case $1<p < ({n+2})/({n-2}) .$ In the critical case, when $ p= ({n+2})/({n-2}),$ Adimurthi and Mancini [1] obtained a solution of (1-3). There have been developments on the asymptotic behaviour of solutions to such equations. In the subcritical case, $1<p < ({n+2})/({n-2}),$ Ni and Takagi [34, 35] have studied the shape of the least energy solutions of (1-3). They have shown that the least energy solutions tend to zero as the diffusion constant d goes to zero except at a finite number of points. Moreover, the maximum of a solution $u_{d}$ of (1-3) is attained at a unique point on the boundary of $\Omega .$ The critical case was examined by Adimurthi et al. [2] using blow-up analysis. We refer to [23] for the existence, nonexistence and the asymptotic behaviour of solutions to fractional Choquard equations with local perturbations.

We mention that Problem (1-1), which we explore in this paper is a nonlocal analogue of the classical problem (1-3).

The substitution of standard diffusion with fractional diffusion is a perceived approach in modelling feeding procedures across a wide range of organisms. In many situations observed in nature, Lévy flights are often used as an accomplished search strategy by living organisms [5, 29]. Since the fractional Laplacian $(-\Delta )^{s}$ is an infinitesimal generator of a Lévy process, dispersal is better modelled by the nonlocal operator $(-\Delta )^{s}$ . The generalised Keller–Segel model with nonlocal diffusion term $d(-\Delta )^{s}$ , where d is a positive constant is used to investigate chemotaxis with anomalous diffusion. For the fractional Keller–Segel model, we refer to [18, 26]. In [26], Huang and Liu studied the existence, stability, uniqueness and regularity of solutions for the following model in dimension $n \geq 2$ :

$$ \begin{align*} \begin{cases} u_{t}= d(-\Delta)^{s} u- \nabla \cdot (u \nabla \phi ), & x \in \mathbb{R}^n, \,\,\,t \geq 0, \\ -\Delta \phi=u, & \\ u(x,0)=u_{0}(x), \end{cases} \end{align*} $$

where d is a positive constant, $u(t, x)$ is the density of some biological cells and $\phi (t, x)$ is the chemical substance concentration. We mention the work [9], where the authors have investigated the asymptotic behaviour of solutions for nonlinear elliptic problems for fractional Laplacians with Dirichlet boundary conditions. We refer to [15] for the regularity, monotonicity and other results on fractional equations in Lipschitz sets, [22] for the existence of solutions to critical Neumann problems and [32] for an in-depth treatment of variational methods to nonlocal fractional problems.

Motivated by the above literature, the works on the fractional Laplacian [33, 36, 38, 39] and the very recent works on the nonlocal Neumann problem for fractional Laplacians and its connections with fractional Keller–Segel models, we have the following natural question to ask.

Question. Can we establish the asymptotic behaviour of the least energy solutions of (1-1)?

The aim of this paper is to answer the above question. More precisely, we discuss the asymptotic behaviour of the least energy solutions of (1-1).

A weak solution of (1-1) can be obtained as a critical point of the following energy functional $J_{d}$ :

$$ \begin{align*} J_{d}(u):=\frac{1}{2} \bigg[ \frac{dc_{n,s}}{2} \int_{T(\Omega)} \frac{\vert u(x)-u(y)\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}u^{2}\,dx \bigg] -\frac{1}{p+1}\int_{\Omega}{\lvert u \rvert}^{p+1}\,dx, \quad u \in H_{\Omega}^{s}. \end{align*} $$

In the above equation, $T(\Omega )= \mathbb {R}^{2n}\setminus (\mathcal {C} \Omega )^{2}$ and the space $H_{\Omega }^{s}$ is defined in (2-1). The functional $J_{d}$ is well defined and of class $C^2$ by Theorem 2.1, stated next. An application of the Mountain-Pass lemma applied to the functional $J_{d}$ yields that

(1-4) $$ \begin{align} c_{d}:=\inf_{\gamma \in \Gamma}\max_{[0,1]}J_{d}(\gamma(t)) \end{align} $$

is a critical value of $J_{d}.$ In the above equation, by $\Gamma ,$ we mean the following set:

$$ \begin{align*} \Gamma = \{\gamma \in C([0,1]; H^{s}_{\Omega}) \mid \gamma(0)=1, \, \gamma(1)=u \}, \end{align*} $$

where $u \in H^{s}_{\Omega }$ , and $u>0$ satisfies $J_{d}(u)=0.$ It turns out that $c_d$ is the least positive critical value; see Lemma 3.3. For the details, one may refer to [4, Theorem 6.1] and [7, Theorem 1.1], where the authors have obtained a nonnegative weak solution $u_{d}$ of (1-1) with critical value $c_{d}$ , provided d is sufficiently small. Moreover, $u_{d}$ satisfies

$$ \begin{align*}0< J_{d}(u_{d}) \leq C d^{{n}/{2s}},\end{align*} $$

where the constant C is independent of $d.$ Consequently, $u_{d}$ is nonconstant. From the proof of [7, Theorem 1.1], it is immediate to see that the critical points of $J_{d}$ are not sign-changing in $\Omega $ . In fact, when $u_{d} \leq 0,$ we can choose $-u_{d}$ to have a nonnegative solution of (1-1). By the strong maximum principle (see [10, Theorem 2.6]), one can see that $u_{d}>0$ almost everywhere (a.e.) in $\Omega .$ Further, since $u_{d}$ satisfies the Neumann condition, $\mathcal {N}_{s}u_{d}(x)=0$ in $\mathcal {C} \Omega $ , which implies that $u_{d}>0$ a.e. in $\mathbb {R}^{n}.$

Definition 1.1. We call a critical point $u_{d}$ of $J_{d}$ with $J_{d}(u_{d})=c_{d}$ the least energy solution or Mountain-Pass solution of (1-1).

We show the asymptotic behaviour of the least energy solutions of (1-1) following a similar approach to that of Ni and Takagi [35] for (1-3). They used a positive solution w of the nonlinear Schrödinger equation

$$ \begin{align*}- \Delta u + u= \vert u\vert^{p-1}u \text{ in } \mathbb{R}^{n}, \quad 1<p< \frac{n+2}{n-2}\end{align*} $$

to study the asymptotic behaviour of the least energy solutions of (1-3). The fractional nonlinear Schrödinger equation

(1-5) $$ \begin{align} (-\Delta)^{s}u + u = \vert u\vert^{p-1}u \quad\text{in } \mathbb{R}^{n}, \end{align} $$

where $1<p<({n+2s})/({n-2s}), n>\max \{1, 2s\},\,0<s<1$ , is thoroughly studied; see for instance [8, 13, 20, 21] and the references therein.

Let us discuss the main idea of this work, which goes as follows.

Let $c_{d}$ be the critical value of $J_{d},$ which is defined in (1-4). Following the arguments of [35], we use a positive solution w of (1-5) to observe the asymptotic behaviour of $c_{d}$ as $d\downarrow 0.$ More specifically, w is used to build a suitable function $\phi _d$ to compare $c_d$ with $\max _{t\geq 0} J_{d}(t \phi _{d}).$ In particular, we obtain an inequality

$$ \begin{align*} c_{d}< \frac{d^{{n}/{2s}}}{2}F(w) \end{align*} $$

for d sufficiently small, where F is the functional associated with (1-5), defined in (2-2). This is closely related to the location of the maximum point of a solution $u_{d}$ of (1-1) on the boundary of $\Omega .$

Now, we summarise the above discussion in terms of the following three main theorems. A priori, it is known that for $1 \leq p < ({n+s})/({n-s}),$ any weak solution u of (1-1) satisfies

$$ \begin{align*} \Vert u\Vert_{L^{\infty}(\Omega)} \leq K, \end{align*} $$

where $K>0$ is some constant depending on $\Omega , p$ and d; see [33, Theorem 3.1]. In the next result, we obtain a bound for the least energy solution $u_{d}$ of (1-1), which is independent of $d.$

Theorem 1.2. Let $u_{d}$ be the least energy solution of (1-1). Then

(1-6) $$ \begin{align} d \frac{c_{n,s}}{2} \int_{T(\Omega)} \frac{\vert u_{d}(x)-u_{d}(y)\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}u_{d}^{2}\,dx=& \int_{\Omega} u_{d}^{p+1}\,dx \leq C_{0}d^{{n}/{2s}}, \end{align} $$

where $C_{0}>0$ is some constant depending on $p.$ Moreover, there is a constant $C_{1}>0$ depending only on p and $\Omega $ such that

$$ \begin{align*} \sup_{\Omega}u_{d}(x)\leq C_{1}. \end{align*} $$

In the next theorem, we show that the $L^r$ -norm of the least energy solution $u_d$ is bounded by $d^{{n}/{2s}}$ times some constant independent of $d.$

Theorem 1.3. Let $u_{d}$ be the least energy solution of (1-1). Then

(1-7) $$ \begin{align} b(r)d^{{n}/{2s}}\leq \int_{\Omega} u_{d}^{r}\,dx \leq B(r)d^{{n}/{2s}} \quad \text{if } 1\leq r \leq \infty, \end{align} $$

(1-8) $$ \begin{align} b(r)d^{{n}/{2s}}\leq \int_{\Omega} u_{d}^{r}\,dx \leq B(r)d^{{nr}/{2s}} \quad \text{if } 0<r<1, \end{align} $$

where $b(r)$ and $B(r)$ are positive constants such that $b(r)<B(r)$ and are independent of $d.$

We show the asymptotic behaviour in the next theorem.

Theorem 1.4. Let $\Omega \subset \mathbb {R}^n$ be a bounded domain of class $C^{1,1}.$ Let $u_{d}$ be the least energy solution of (1-1). If $u_{d}$ achieves a maximum at a point $z_{d} \in \overline {\Omega },$ then for all d sufficiently small, we have $z_{d} \in \partial \Omega .$

The plan of the paper is as follows. In Section 2, we recollect known results that are useful for our analysis. In Section 3, we study the regularity of the least energy solution of (1-1) and complete the proof of Theorem 1.2. In Section 4, we derive $L^{r}$ -estimates for the least energy solutions of (1-1). Section 5 contains the proof of Theorem 1.4. The proof of inequality (3-8) is a part of Appendix A.

2. Auxiliary results

Let us recall some important results that are used in this paper.

Theorem 2.1 (Fractional Sobolev embedding [12])

Let $n>2s$ and $2_{s}^{*}={2n}/({n-2s})$ be the fractional critical exponent. Then, we have the following inclusions.

1. (1) For any function $u\in C_{0}(\mathbb {R}^{n})$ and for $q\in [0, 2_{s}^{*}-1],$

   $$ \begin{align*} \Vert u\Vert_{L^{q+1}(\mathbb{R}^{n})}^{2} \leq B(n,s)\int_{\mathbb{R}^{n}}\int_{\mathbb{R}^{n}} \frac{\vert u(x)-u(y)\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy \end{align*} $$
   for some positive constant $B.$ That means $H^{s}(\mathbb {R}^{n})$ is continuously embedded in $L^{q+1}(\mathbb {R}^{n}).$

2. (2) Let $\Omega \subset \mathbb {R}^{n}$ be a bounded extension domain for $H^{s}(\Omega ).$ Then, the space $H^{s}(\Omega )$ is continuously embedded in $L^{q+1}(\Omega )$ for any $q\in [0, 2_{s}^{*}-1],$ that is,

   $$ \begin{align*} \Vert u\Vert_{L^{q+1}(\Omega)}^{2} \leq B(n,s,\Omega)\Vert u\Vert_{H^{s}(\Omega)}^{2}\end{align*} $$
   for some positive constant $B.$ Further, the above embedding is compact for any $q\in [0, 2_{s}^{*}-1).$

Let $T(\Omega ):=\mathbb {R}^{2n}\setminus (\mathbb {R}^{n}\setminus \Omega )^{2}$ be a cross-shaped set on a bounded domain $\Omega \subset \mathbb {R}^n$ . Define

(2-1) $$ \begin{align} H_{\Omega}^{s}:=\{ u: \mathbb{R}^{n}\longrightarrow \mathbb{R} \text{ measurable}: {\Vert u\Vert}_{H_{\Omega}^{s}}< \infty \}, \end{align} $$

which is equipped with the norm

$$ \begin{align*} \Vert u\Vert_{H_{\Omega}^{s}}:=\bigg(\Vert u\Vert_{L^{2} (\Omega)}^{2}+\int_{T(\Omega)}\frac{{\lvert u(x)-u(y)\rvert}^{2}}{{\lvert x-y \rvert}^{n+2s}}\,dx\,dy \bigg)^{{1}/{2}}. \end{align*} $$

Remark 2.2. Here, $H_{\Omega }^{s}$ is a Hilbert space (see [14, Proposition 3.1]).

Let us define the following set:

$$ \begin{align*}\mathcal{L}_{s}:= \bigg\{u: \mathbb{R}^{n}\longrightarrow \mathbb{R} \text{ measurable}: \int_{\mathbb{R}^n} \frac{\vert u(x)\vert}{1+\vert x\vert^{n+2s}}\,dx < \infty \bigg\}.\end{align*} $$

The condition $u \in \mathcal {L}_{s}$ is useful to give a sense to the pointwise definition of fractional Laplacians (1-2).

Lemma 2.3 [10, Lemma 2.3]

Let $\Omega \subset \mathbb {R}^n$ be a bounded set. Then, $H^{s}_{\Omega } \subset \mathcal {L}_{s}.$

Next, we recall a few known results about the fractional Schrödinger equation (1-5).

Definition 2.4. A measurable function $u: \mathbb {R}^{n} \longrightarrow \mathbb {R}$ is called a weak solution of (1-5) if it satisfies the following equation:

$$ \begin{align*} & \frac{c_{n,s}}{2} \int_{\mathbb{R}^{n}}\int_{\mathbb{R}^{n}} \frac{(u(x)-u(y))(\psi(x)-\psi(y))}{\vert x-y\vert^{n+2s}}\,dx\,dy\\ &\quad + \int_{\mathbb{R}^{n}}u(x)\psi(x)\,dx = \int_{\mathbb{R}^{n}} \vert u(x)\vert^{p-1}u(x)\psi(x)\,dx \end{align*} $$

for all $\psi \in C_{0}^{1}(\mathbb {R}^{n}).$

We define the corresponding energy functional $F: H^{s}(\mathbb {R}^n) \longrightarrow \mathbb {R} $ as follows:

(2-2) $$ \begin{align} F(u):=\frac{1}{2} \bigg[ \frac{c_{n,s}}{2} \int_{\mathbb{R}^{n}}\int_{\mathbb{R}^{n}} \frac{\vert u(x)-u(y)\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\mathbb{R}^{n}}u^{2}\,dx \bigg] -\frac{1}{p+1}\int_{\mathbb{R}^{n}}|u|^{p+1}\,dx. \end{align} $$

The weak solutions of (1-5) correspond to the critical points of $F.$

Definition 2.5. A function $u \in \mathcal {L}_{s}(\mathbb {R}^{n}) \cap C^{2s+\epsilon }(\mathbb {R}^n),$ when $0<s<\tfrac 12$ , $2s+\epsilon <1$ , or $u \in C^{1, 2s+\epsilon -1}(\mathbb {R}^n) \cap \mathcal {L}_{s}(\mathbb {R}^{n}),$ when $\tfrac 12\leq s<1$ , $2s+\epsilon -1<1 $ , is said to be a classical solution of (1-5) if it satisfies (1-5) pointwise in $\mathbb {R}^{n}.$

The next result gives us a positive, radially symmetric solution of (1-5), which decays at infinity.

Theorem 2.6 [20, Theorem 3.4]

Let u be the weak solution of (1-5). Then, $u \in L^{q}(\mathbb {R}^{n}) \cap C^{\alpha }(\mathbb {R}^{n})$ for some $q \in [2, \infty ) $ and $\alpha \in (0,1).$ Moreover,

$$ \begin{align*} \lim_{\vert x\vert \rightarrow \infty}u(x)=0. \end{align*} $$

Theorem 2.7 [20, Theorem 1.3]

Equation (1-5) has a weak solution in $H^{s}(\mathbb {R}^{n}),$ which satisfies $u \geq 0$ a.e. in $\mathbb {R}^{n}.$ Moreover, u is a classical solution, which satisfies $u>0$ in $\mathbb {R}^{n}.$

The following theorem shows that the solutions of (1-5) have a power type of decay at infinity.

Theorem 2.8 [20, Theorem 1.5]

Let u be a positive classical solution of (1-5) such that

$$ \begin{align*}\lim_{\vert x\vert \rightarrow \infty}u(x)=0.\end{align*} $$

Then, there exist constants $0< C_{1} \leq C_{2}$ such that

$$ \begin{align*} \frac{C_{1}}{\vert x\vert^{n+2s}}\leq u(x) \leq \frac{C_{2}}{\vert x\vert^{n+2s}} \quad \text{for all } \vert x\vert\geq 1. \end{align*} $$

One can see that there exist some $m>0$ and $s_{0}>0$ such that for $f(u)=u^{p}-u,$

$$ \begin{align*} \frac{f(v)-f(u)}{v-u}\leq \frac{v^{p}-u^{p}}{v-u}\leq C (v+u)^{m} \quad\text{for all } 0 < u< v < s_{0}, \end{align*} $$

where $C>0$ is some constant. Also, it is simple to see that $f: [0,\infty ) \to \mathbb {R}$ is locally Lipschitz. Consequently, we have the following result on the radial symmetry and monotonicity property of positive solutions of (1-5).

Theorem 2.9 [21, Theorem 1.2]

Let u be a positive classical solution of (1-5) such that

$$ \begin{align*}\lim_{\vert x\vert \rightarrow \infty}u(x)=0.\end{align*} $$

Further, assume that there exists

$$ \begin{align*}t> \max \bigg\{\frac{2s}{m}, \frac{n}{m+2} \bigg\}\end{align*} $$

such that u satisfies $u(x)=O({1}/{\vert x\vert ^{t}})$ as $ \vert x\vert \rightarrow \infty .$ Then, u is radially symmetric and strictly decreasing about some point in $\mathbb {R}^{n}.$

Remark 2.10. Since

$$ \begin{align*} \frac{C_{1}}{\vert x\vert^{n+2s}}\leq u(x) \leq \frac{C_{2}}{\vert x\vert^{n+2s}} \quad \text{for all } \vert x\vert\geq 1, \end{align*} $$

we can take $t=n+2s$ in the above theorem.

Now, [37, Proposition 4.1] ascertains that if $u \in \mathbb {R}^n$ is a weak solution of (1-5), then u satisfies the following Pohozaev identity:

$$ \begin{align*} \mathcal{P}(u):= \frac{(n-2s)c_{n,s}}{4}\int_{\mathbb{R}^n} \int_{\mathbb{R}^n} \frac{\vert u(x)-u(y)\vert^2}{\vert x-y\vert^{n+2s}}\,dx\,dy + \frac{n}{2}\int_{\mathbb{R}^n} u^2 \,dx-\frac{n}{p+1} \int_{\mathbb{R}^n} u^{p+1}=0. \end{align*} $$

Let us define

$$ \begin{align*} \mathcal{G}:=\{ u \in H^s(\mathbb{R}^n) \setminus \{0\} \mid \mathcal{P}(u)=0 \}. \end{align*} $$

In [8], the authors have obtained a weak solution $w \in H^{s}(\mathbb {R}^{n})$ of (1-5) with the least energy among all other solutions. In particular, they have proved the following result.

Theorem 2.11 [8, Theorem 1.2]

Equation (1-5) has a weak solution $w \in H^s(\mathbb {R}^n)$ such that

$$ \begin{align*}0<F(w)=\inf_{u \in \mathcal{G}}F(u) .\end{align*} $$

Combining Theorems 2.7, 2.8, 2.9 and 2.11, we have the following result.

Theorem 2.12. Equation (1-5) has a positive classical solution $w \in H^{s}(\mathbb {R}^n)$ satisfying:

1. (a) w has a power type of decay at infinity, that is, there exist constants $0< C_{1} \leq C_{2}$ such that

   $$ \begin{align*} \frac{C_{1}}{\vert x\vert^{n+2s}}\leq w(x) \leq \frac{C_{2}}{\vert x\vert^{n+2s}} \quad \text{for all } \vert x\vert\geq 1; \end{align*} $$

2. (b) w is radially symmetric, that is, $w(x)=w(r)$ with $r=\vert x\vert ;$

3. (c) for any nonnegative classical solution $u \in H^{s}(\mathbb {R}^{n})$ of (1-5), $0<F(w) \leq F(u)$ holds unless $u=0.$

Definition 2.13. We call $w,$ given by Theorem 2.12, a ground state solution of (1-5).

3. Regularity and bounds for the least energy solution $u_{d}$

Let $s\in (0,1)$ and $\Omega \subset \mathbb {R}^{n}$ be a bounded domain of class $C^{1,1}.$

Definition 3.1. A measurable function $u: \mathbb {R}^{n} \longrightarrow \mathbb {R}$ is said to be a weak solution of (1-1) if it satisfies the following equation:

(3-1) $$ \begin{align} &\frac{dc_{n,s}}{2} \int_{T(\Omega)} \frac{(u(x)-u(y))(\psi (x)-\psi(y))}{\vert x-y\vert^{n+2s}}\,dx\,dy\nonumber\\ &\quad+ \int_{\Omega}u(x)\psi(x)\,dx=\int_{\Omega} \vert u(x)\vert^{p-1}u(x)\psi(x)\,dx \end{align} $$

for all $\psi \in H_{\Omega }^{s}.$

We have the following result on the existence of a weak solution of (1-1).

Theorem 3.2 ([4, Theorem 6.1], [7, Theorem 1.1])

There exists a nonnegative weak solution $u_{d}$ of (1-1) with critical value $c_{d}$ , provided d is sufficiently small. Moreover, $u_{d}$ satisfies

$$ \begin{align*}0< J_{d}(u_{d}) \leq C d^{{n}/{2s}},\end{align*} $$

where the constant C is independent of $d.$ Consequently, $u_{d}$ is nonconstant.

Define

$$ \begin{align*}M[v]:=\sup_{t \geq 0}J_{d}(tv), \quad v\in H_{\Omega}^{s}.\end{align*} $$

In the next lemma, we indicate a useful characterisation of the critical value $c_{d}.$ We follow similar lines of proof to [35, Lemma 3.1].

Lemma 3.3. The critical value $c_{d}$ is independent of the choice of $u \in H_{\Omega }^{s}$ such that $u \geq 0,~u \not \equiv 0$ and $J_{d}(u)=0.$ In fact, $c_{d}$ is the least positive critical value of $J_{d},$ which is given by

(3-2) $$ \begin{align} c_{d}=\inf\{M[v] \mid v\in H_{\Omega}^{s},~v\not \equiv 0, v\geq 0 \text{ in } \Omega \}. \end{align} $$

Proof. For $v\in H_{\Omega }^{s},$ let

$$ \begin{align*}\Omega^{+}=\{x \in \Omega \mid v(x)>0\}.\end{align*} $$

Now, for all those v satisfying $\vert \Omega ^{+}\vert>0,$ define

$$ \begin{align*}g_{d}(t):=J_{d}(tv) \quad \text{for } t \geq 0. \end{align*} $$

First, we show that $g_{d}(t)$ has a unique maximum. For this,

$$ \begin{align*} g^{\prime}_{d}(t)= t\bigg[ \frac{dc_{n,s}}{2} \int_{T(\Omega)} \frac{\vert v(x)-v(y)\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}v^{2}\,dx \bigg] -t^{p}\int_{\Omega}{v }^{p+1}\,dx. \end{align*} $$

Therefore, $g^{\prime }_{d}(t_{0})=0$ for some $t_{0}>0$ if and only if

$$ \begin{align*}\frac{dc_{n,s}}{2} \int_{T(\Omega)} \frac{\vert v(x)-v(y)\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}v^{2}\,dx = t_{0}^{p-1}\int_{\Omega} v^{p+1}\,dx.\end{align*} $$

Note that the right-hand side is strictly increasing in $t_{0}.$ And hence there exists a unique $t_{0}>0$ such that $g^{\prime }_{d}(t_{0})=0.$ Since $g_{d}(t)>0$ for $t>0$ small and $g_{d}(t) \rightarrow -\infty $ as $t \rightarrow +\infty ,$ one easily find that $g_{d}(t)$ has a unique maximum.

Let us fix a function $u \not \equiv 0, u\geq 0$ in $H_{\Omega }^{s} $ with $J_{d}(u)=0.$ Let $u_{d}$ be a positive solution of (1-1) obtained by applying the Mountain-Pass lemma and $c_{d}$ the corresponding critical value. We have $J_{d}(u_{d})=c_{d}$ and $J_{d}^{'}(u_{d})=0.$ Since $u_{d}>0$ and $J_{d}^{'}(u_{d})=0,$

(3-3) $$ \begin{align} M[u_{d}]=c_{d}, \end{align} $$

and hence

(3-4) $$ \begin{align} c_{d}\geq \inf\{M[v] \mid v\in H_{\Omega}^{s},~v\not \equiv 0, v\geq 0 \text{ in } \Omega \}. \end{align} $$

In contrast, assume that strict inequality occurs in (3-4). Then,

$$ \begin{align*}M[v_{0}]<c_{d},\end{align*} $$

for some $v_{0} \geq 0,\, v_{0} \not \equiv 0 $ in $H_{\Omega }^{s}. $ Therefore, there exists some $t_{1}>0$ such that $t_{1}v_{0}=u_{0}$ satisfies $J_{d}(u_{0})=0.$ Denote by U the subspace of $H_{\Omega }^{s}$ spanned by u and $u_{0}.$ Consider the subset of U defined as follows:

$$ \begin{align*}U^{+}:=\{ \alpha u + \beta u_{0}\mid \alpha,~\beta \geq 0 \}.\end{align*} $$

Suppose S is a circle on U of radius R so large that $R> \max \{ \Vert u\Vert , \Vert u_{0}\Vert \}$ and $J_{d}\leq 0$ on $S \cap U^{+}.$ Assume that $\gamma $ is the path made up of the line segment with endpoints $0$ and ${Ru_{0}}/{\Vert u_{0}\Vert },$ the circular arc $ S\cap U^{+}$ and the line segment with endpoints ${Ru}/{\Vert u\Vert }$ and $u.$ One can easily see that, along $\gamma $ , $J_{d}$ is positive only on the line segment joining $0$ and $u_{0}.$ Hence,

$$ \begin{align*} \max_{v \in \gamma}J_{d}(v)=M[v_{0}] < c_{d},\end{align*} $$

which is a contradiction to (1-4). Thus, we have equality in (3-4), that is,

$$ \begin{align*} c_{d}= \inf\{M[v] \mid v\in H_{\Omega}^{s},~v\not \equiv 0, v\geq 0 \text{ in } \Omega \}. \end{align*} $$

Note that $J_{d}(v)=J_{d}(-v)$ for any $v \in H^{s}_{\Omega }$ . Since any nontrivial critical point of $J_{d}$ is either positive or negative a.e. in $\Omega ,$ from the above discussion, one can see that $c_{d}$ is the least positive critical value of $J_{d}$ . This completes the proof.

The following lemma gives us the regularity estimate. A similar result is already proved in [10, Lemma 3.6] and [11, Remark 4.9].

Lemma 3.4. Let $u \in H_{\Omega }^{s}$ be a weak solution of (1-1). Let $u \in L^{\infty }(\Omega ),$ then $u \in L^{\infty }(\mathbb {R}^{n}).$ Moreover:

1. (1) for $0<s<\tfrac 12$ , $u \in C^{2}(\Omega )$ if $p>3-2s$ and $u \in C^{1, p-2+2s}(\Omega )$ if $2<p\leq 3-2s;$

2. (2) for $\tfrac 12 \leq s< 1$ , $u \in C^{2}(\Omega ).$

Now, we prove that the least energy solution $u_{d}$ is bounded by some constant independent of $d.$

Proof of Theorem 1.2

The proof of the first inequality of Theorem 1.2 is fairly standard and simple, and can be seen in the literature; for instance, see [8, Theorem 1.1]. Since it is short, for the sake of completeness, we include it here. For this,

$$ \begin{align*} J_{d}(u_{d}):=\frac{1}{2} \bigg[ \frac{c_{n,s}d}{2} \int_{T(\Omega)} \frac{\vert u_{d}(x)-u_{d}(y)\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}u^{2}\,dx \bigg] -\frac{1}{p+1}\int_{\Omega}u_{d}^{p+1}\,dx. \end{align*} $$

Since $u_{d}$ is a critical point of $J_{d}$ ,

$$ \begin{align*} J_{d}^{\prime}(u_{d})=0 \quad\text{on } H_{\Omega}^{s}. \end{align*} $$

This implies that

(3-5) $$ \begin{align} d\frac{c_{n,s}}{2} \int_{T(\Omega)} \frac{\vert u_{d}(x)-u_{d}(y)\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}u_{d}^{2}\,dx =\int_{\Omega}u_{d}^{p+1}\,dx. \end{align} $$

Hence, from the above equations,

(3-6) $$ \begin{align} J_{d}(u_{d})&= \bigg(\frac{1}{2}-\frac{1}{p+1}\bigg)\int_{\Omega}u_{d}^{p+1}\,dx \\&= \frac{(p-1)}{2(p+1)} \int_{\Omega}u_{d}^{p+1}\,dx.\nonumber \end{align} $$

Now, by Theorem 3.2, we have $J_{d}(u_{d})\leq C d^{{n}/{2s}}$ , where the constant C depends only on $p.$ Using this inequality in the above equation,

$$ \begin{align*} \int_{\Omega}u_{d}^{p+1}\,dx \leq \frac{2(p+1)}{p-1}Cd^{{n}/{2s}}. \end{align*} $$

Taking $C_{0}={2(p+1)}/({p-1})C$ proves the first inequality of Theorem 1.2. The proof of the second inequality of Theorem 1.2 is a little constructive. We claim that

$$ \begin{align*}\displaystyle \sup_{\Omega}u_{d}(x) \leq C_{1}\end{align*} $$

for some constant $C_{1}>0$ depending on p and $\Omega $ only. Multiplying (1-1) by $u_{d}^{2t-1}$ and integrating over $\Omega ,$

(3-7) $$ \begin{align} \frac{c_{n,s}d}{2}\int_{T(\Omega)} \frac{(u_{d}(x)-u_{d}(y))(u_{d}^{2t-1}(x)-u_{d}^{2t-1}(y))}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}u_{d}^{2t}\,dx = \int_{\Omega}u_{d}^{p+2t-1}\,dx. \end{align} $$

Now, we use the following inequality. We give the proof of this inequality in the Appendix. Let $x, y \geq 0$ be real numbers and $k\geq 1$ , then

(3-8) $$ \begin{align} \frac{1}{k}(x^{k}-y^{k})^{2}\leq (x-y)(x^{2k-1}-y^{2k-1}). \end{align} $$

Consequently,

(3-9) $$ \begin{align} \frac{1}{t}\int_{T(\Omega)} \frac{(u_{d}^{t}(x)-u_{d}^{t}(y))^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy \leq \int_{T(\Omega)} \frac{(u_{d}(x)-u_{d}(y))(u_{d}^{2t-1}(x)-u_{d}^{2t-1}(y))}{\vert x-y\vert^{n+2s}}\,dx\,dy. \end{align} $$

From (3-7) and (3-9),

(3-10) $$ \begin{align} \frac{dc_{n,s}}{2t}\int_{T(\Omega)} \frac{(u_{d}^{t}(x)-u_{d}^{t}(y))^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}u_{d}^{2t}\,dx \leq \int_{\Omega}u_{d}^{p+2t-1}\,dx. \end{align} $$

Further, by the fractional Sobolev embedding (Theorem 2.1),

(3-11) $$ \begin{align} \bigg(\int_{\Omega}{\vert v\vert^{2_{s}^{*}}} \bigg)^{2/2_{s}^{*}} \leq \frac{A}{d}\bigg(d\frac{c_{n,s}}{2}\int_{\Omega}\int_{\Omega} \frac{\vert v(x)-v(y)\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}\vert v\vert^{2}\,dx \bigg), \end{align} $$

where $d\in (0,d_{0})$ for some $d_{0}>0$ , $A>0$ some constant, $v\in H^{s}(\Omega )$ and $2_{s}^{*}={2n}/({n-2s}).$ The embedding constant A depends only on n, s, $d_{0}$ and $\Omega .$ To see this, let us define

$$ \begin{align*} \Omega_{d}:=\bigg \{ y : \frac{y}{d^{1/2s}} \in \Omega \bigg \} \quad\text{and}\quad w(y):=v \bigg (\frac{y}{d^{1/2s}} \bigg ),\quad\text{where } y \in \Omega_{d}. \end{align*} $$

Now,

$$ \begin{align*} &d\int_{\Omega}\int_{\Omega} \frac{\vert v(x)-v(y)\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}{v}^{2}\,dx\\&\quad= \frac{1}{d^{{n}/{2s}}} \bigg [ \int_{\Omega_{d}}\int_{\Omega_{d}} \frac{\vert v (\tfrac{x'}{d^{{1}/{2s}}} )-v(\tfrac{y'}{d^{{1}/{2s}}} )\vert^{2}}{\vert x'-y'\vert^{n+2s}}\,dx'\,dy' + \int_{\Omega_{d}}{v\bigg (\frac{x'}{d^{{1}/{2s}}} \bigg )}^{2}\,dx' \bigg ] \\&\quad= \frac{1}{d^{{n}/{2s}}} \bigg [ \int_{\Omega_{d}}\int_{\Omega_{d}} \frac{\vert w(x')-w(y')\vert^{2}}{\vert x'-y'\vert^{n+2s}}\,dx'\,dy' + \int_{\Omega_{d}}{w(x')}^{2}\,dx' \bigg ] \\&\quad\geq \frac{A}{d^{{n}/{2s}}} \bigg(\int_{\Omega_{d}}{\vert w\vert^{2_{s}^{*}}} \,dx' \bigg)^{{2}/{2_{s}^{*}}} \\&\quad= Ad^{({2}/{2_{s}^{*}}-1 ){n}/{2s}} \bigg(\int_{\Omega}{\vert v\vert^{2_{s}^{*}}} \,dx \bigg)^{{2}/{2_{s}^{*}}}. \end{align*} $$

Therefore, we observe that A is uniform for $d \in (0, d_{0}).$

It is easy to see that $\Omega \times \Omega \subset T(\Omega ).$ Then, by virtue of (3-10) and (3-11),

(3-12) $$ \begin{align} \bigg(\int_{\Omega}{\vert u_{d}\vert^{t2_{s}^{*}}} \bigg)^{{2}/{2_{s}^{*}}} \leq \frac{tA}{d}\int_{\Omega}u_{d}^{p+2t-1}\,dx. \end{align} $$

Now, we define two sequences $\{L_{j} \}$ and $\{M_{j} \}$ by the following recurrence relations:

(3-13) $$ \begin{align} p-1+2L_{0}= & \, 2_{s}^{*}, \nonumber \\ p-1+2L_{j+1}= & \, 2_{s}^{*}L_{j}, \quad j=0,1,2,\ldots \end{align} $$

(3-14) $$ \begin{align} M_{0}=& \, (AC_{0})^{{2_{s}^{*}}/{2}}, \nonumber \\ M_{j+1}=& \, (AL_{j}M_{j})^{{2_{s}^{*}}/{2}}, \quad j=0,1,2,\ldots \end{align} $$

We note that $L_{j}$ is explicitly given by

(3-15) $$ \begin{align} L_{j}=\frac{1}{(2_{s}^{*}-2)}\bigg( \bigg(\frac{2_{s}^{*}}{2}\bigg)^{j+1}(2_{s}^{*}-p-1)+p-1 \bigg). \end{align} $$

Since $1<p<2_{s}^{*}-1$ , it follows that $L_{j}\geq 1$ for all $j \geq 0$ and $L_{j} \rightarrow \infty $ as $j \rightarrow \infty .$ We show that

(3-16) $$ \begin{align} \int_{\Omega}u_{d}^{p-1+2L_{j}}\,dx \leq & \, M_{j}d^{{n}/{2s}} \quad\text{for all } j\geq 0, \end{align} $$

and

(3-17) $$ \begin{align} M_{j}\leq e^{mL_{j-1}} \end{align} $$

for some constant $m>0.$ Then,

$$ \begin{align*} \sup_{\Omega}u_{d}(x)\leq C_{1},\end{align*} $$

where $C_{1}>0$ depends only on $C_{0}$ and $\Omega .$ In fact, (3-15) and (3-16) give

$$ \begin{align*} \Vert u\Vert_{L^{2_{s}^{*}L_{j-1}}(\Omega)} &\leq ( e^{mL_{j-1}}d^{{n}/{2s}} )^{{1}/{(2_{s}^{*}L_{j-1})}} \nonumber \\ &= e^{{m}/{2_{s}^{*}} d^{{(n-2s)}/{4L_{j-1}}}} \end{align*} $$

and hence letting $j \rightarrow \infty ,$

$$ \begin{align*}\Vert u\Vert_{L^{\infty}(\Omega)} \leq e^{{m}/{2_{s}^{*}}}.\end{align*} $$

First, we verify (3-16). By virtue of (1-6) and (3-11),

$$ \begin{align*} \bigg(\int_{\Omega}{\vert u_{d}\vert^{2_{s}^{*}}} \bigg)^{{2}/{2_{s}^{*}}} &\leq \frac{A}{d}\bigg( \frac{c_{n,s}d}{2}\int_{T(\Omega)} \frac{\vert u_{d}(x)-u_{d}(y)\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}\vert u_{d}\vert^{2}\,dx \bigg) \nonumber \\ &\leq \frac{A}{d}C_{0}d^{{n}/{2s}} \nonumber \\ &= AC_{0}d^{{n}/{s2_{s}^{*}}}. \end{align*} $$

Hence, (3-16) holds for $j=0.$ Suppose that we have proved (3-16) for $j \geq 0.$ Then, by (3-12),

$$ \begin{align*} \int_{\Omega}{\vert u_{d}\vert^{p-1+2L_{j+1}}}\,dx &\leq \bigg (\frac{L_{j}A}{d}\int_{\Omega}u_{d}^{p+2L_{j}-1}\,dx \bigg )^{{2_{s}^{*}}/{2}} \nonumber \\ &\leq ( AL_{j}d^{-1}M_{j}d^{{n}/{2s}} )^{{2_{s}^{*}}/{2}} \nonumber \\ &= (AL_{j}M_{j} )^{{2_{s}^{*}}/{2}}d^{{n}/{2s}}. \end{align*} $$

This implies that (3-16) is also true for $j+1.$ Therefore, it remains to show (3-17). Put

(3-18) $$ \begin{align} \lambda_{j}= & \, \frac{2_{s}^{*}}{2} \cdot \log(AL_{j}) \quad\text{and}\quad \eta_{j}= \log(M_{j}). \end{align} $$

Hence,

$$ \begin{align*} \eta_{j+1}=& \, \frac{2_{s}^{*}}{2}\cdot \eta_{j} +\lambda_{j}. \end{align*} $$

The explicit value of $L_{j}$ is given by

(3-19) $$ \begin{align} L_{j}= (2_{s}^{*}-2)^{-1} ( (2^{-1}2_{s}^{*})^{j+1}(2_{s}^{*}-p-1)+p-1 ). \end{align} $$

Now,

$$ \begin{align*} \lambda_{j}=& \, \frac{2_{s}^{*}}{2}\log\bigg[ \frac{A}{(2_{s}^{*}-2)}((2^{-1}2_{s}^{*})^{j+1}(2_{s}^{*}-p-1)+p-1 ) \bigg ]\\ =& \, \frac{2_{s}^{*}}{2} \bigg [ \log(A(2_{s}^{*}-2))+ \log ((2^{-1}2_{s}^{*})^{j+1}(2_{s}^{*}-p-1)+p-1 ) \bigg ].\nonumber \end{align*} $$

Therefore, we can find some $C^{*}$ such that

$$ \begin{align*} \lambda_{j} \leq C^{*}(j+1). \end{align*} $$

We now define a sequence $ \{\gamma _{j} \}$ by

(3-20) $$ \begin{align} \gamma_{0}= \eta_{0} \quad\text{and}\quad \gamma_{j+1}= \frac{2_{s}^{*}}{2}\gamma_{j} + C^{*}(j+1) \end{align} $$

for $j \geq 1.$ Clearly, $\eta _{j} \leq \gamma _{j}$ for all $j \geq 0.$ Moreover, since

$$ \begin{align*} \gamma_{j}= \bigg (\frac{2_{s}^{*}}{2} \bigg )^{j}( \eta_{0}+2C^{*}2_{s}^{*}(2_{s}^{*}-2)^{-2} )-2C^{*}(2_{s}^{*}-2)^{-1} (j+ 2_{s}^{*}(2_{s}^{*}-2)), \end{align*} $$

in view of (3-19), there exists $m>0$ such that $\gamma _{j} \leq mL_{j-1}.$ Hence, $\log (M_{j}) \leq m L_{j-1}$ and we obtain (3-17). Observe that m depends only on $\eta _{0}$ , $2_{s}^{*}$ and $C^{*},$ whereas $C^{*}$ depends only on $2_{s}^{*}$ , p and $A.$ This completes the proof.

Remark 3.5. It is known that if $u \in \mathcal {L}_{s}(\mathbb {R}^{n})\cap C^{2s+\epsilon }(\Omega ), $ when $0<s<\tfrac 12, 2s+\epsilon <1$ or $u \in \mathcal {L}_{s}(\mathbb {R}^{n})\cap C^{1,2s+\epsilon -1}(\Omega ), $ when $\tfrac 12\leq s<1, 2s+\epsilon -1<1$ , one can compute $(-\Delta )^{s}u(x)$ pointwise for all x in $\Omega .$ In fact, one can write

$$ \begin{align*} (-\Delta)^{s}u(x)=c_{n,s}\ \mathrm{PV} \int_{\mathbb{R}^{n}} \frac{u(x)-u(y)}{\vert x-y\vert^{n+2s}}\,dy. \end{align*} $$

Definition 3.6. We call $u: \mathbb {R}^{n} \longrightarrow \mathbb {R}$ a classical solution of (1-1) if it satisfies the following:

1. (1) $u \in \mathcal {L}_{s}(\mathbb {R}^{n})\cap C^{2s+\epsilon }(\Omega ), $ when $0<s<\tfrac 12, 2s+\epsilon <1$ or $u \in \mathcal {L}_{s}(\mathbb {R}^{n})\cap C^{1,2s+\epsilon -1}(\Omega ), $ when $\tfrac 12\leq s<1, 2s+\epsilon -1<1;$

2. (2) $\mathcal {N}_{s}u(x)=0, \,\,\, x \in \mathbb {R}^{n}\setminus \Omega ;$

3. (3) $d(-\Delta )^{s}u(x)+u(x)= \vert u(x)\vert ^{p-1}u(x)$ pointwise for all $x \in \Omega .$

We make similar remarks as in [6], which offers a relation between the weak and classical solutions of (1-1).

Remark 3.7. Let $u_{d}$ be the least energy solution of (1-1) in $H^{s}_{\Omega }.$ Then, by Lemma 2.3, Theorem 1.2 and Lemma 3.4:

1. (1) for $0<s<\tfrac 12$ , $u_{d} \in \mathcal {L}_{s}(\mathbb {R}^n)\cap C^{2}(\Omega )$ if $p>3-2s$ and $u_{d} \in \mathcal {L}_{s}(\mathbb {R}^n) \cap C^{1, p-2+2s}(\Omega )$ if $2<p\leq 3-2s$ ;

2. (2) for $\tfrac 12 \leq s < 1$ , $u_d \in \mathcal {L}_{s}(\mathbb {R}^n) \cap C^{2}(\Omega ).$

Now, using the nonlocal integration by parts formulae given in [14], one can easily check that

$$ \begin{align*} d(-\Delta)^{s}u_{d}(x) + u_{d}(x)=\vert u_{d}(x)\vert^{p-1}u_{d}(x) \end{align*} $$

holds pointwise in $\Omega .$ This implies that $u_{d}$ is a classical solution of (1-1). Conversely, if $u_{d}$ is a classical solution of (1-1) satisfying $u_{d} \in H^{s}_{\Omega },$ then $u_{d}$ is a weak solution of (1-1).

The following lemma shows that the maximum of the least energy solution is always greater than unity.

Lemma 3.8. Let $u_d$ be the least energy solution of (1-1). Let

$$ \begin{align*} M_{d}=\sup_{x\in \overline{\Omega}} u_{d}(x). \end{align*} $$

Then, $M_{d}>1.$

Proof. Since $u_{d}$ is a weak solution of (1-1),

$$ \begin{align*} &d\frac{c_{n,s}}{2}\int_{T(\Omega)}\frac{(u_{d}(x)-u_{d}(y))(w(x)-w(y))}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}u_{d}w \,dx =\int_{\Omega} u_{d}^{p}w \,dx \end{align*} $$

holds for all $w \in H_{\Omega}^{s}$ . Taking $w=1$ in the above equation,

$$ \begin{align*} \int_{\Omega}u_{d}(x)\,dx=& \,\int_{\Omega}u_{d}^{p}(x)\,dx. \end{align*} $$

This implies that

$$ \begin{align*} \int_{\Omega}u_{d}(x)(1-u_{d}^{p-1}(x))\,dx=& \,0. \end{align*} $$

Now, if $u_{d}(x)\leq 1$ for all $x \in \overline {\Omega },$ then

$$ \begin{align*}1-u_{d}(x)\geq 0 \quad\text{for all } x \in {\overline{\Omega}} .\end{align*} $$

Thus, from the above equation, we get that $u_{d}(x)= 1~a.e.$ in $\overline {\Omega }.$ Now, by Lemma 3.4, we can assume that $u_{d}$ is continuous and hence $u_{d}\equiv 1$ in $\overline {\Omega },$ which is a contradiction to our assumption that $u_{d}$ is a nonconstant solution. Therefore, there exists $x_{0}$ in $\overline {\Omega }$ such that $u_{d}(x_{0})>1.$ Thus, $M_{d}>1.$

4. $L^{r}$ -estimates on $u_{d}$

Here, we derive an $L^{r}$ -estimate for $u_{d}.$ The following results are generalisations of [31, Proposition 2.2 and Lemma 2.3] to the nonlocal case.

Proposition 4.1. For $d_{0}>0$ fixed, there is a constant $K_{0}$ such that

(4-1) $$ \begin{align} d\frac{c_{n,s}}{2}\int_{T(\Omega)}\frac{(u_{d}(x)-u_{d}(y))^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}u_{d}^{2} \,dx \geq K_{0}d^{{n}/{2s}}, \end{align} $$

where $u_{d}$ is the least energy solution of (1-1) with $0<d<d_{0}.$

Proof. In contrast, suppose that there is a sequence $\{d_{k}\}$ contained in the interval $(0,d_{0})$ and a sequence of positive solutions $\{u_{k}\}$ to (1-1) with $d=d_{k}$ such that

$$ \begin{align*} \zeta_{k}:= \frac{1}{d^{{n}/{2s}}}\bigg(d \frac{c_{n,s}}{2} \int_{T(\Omega)}\frac{(u_{k}(x)-u_{k}(y))^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}u_{k}^{2} \,dx \bigg) \rightarrow 0 \quad\text{as } k\rightarrow \infty. \end{align*} $$

We are going to follow the same arguments as used in the proof of Lemma 1.2 to prove this proposition. Once again, define the sequences $\{L_{k}\}$ and $\{M_{j}\}$ as defined earlier in (3-13) and (3-14), respectively. Instead of $C_{0},$ we write $\zeta _{k}$ in the definition of $\{M_{j}\}$ :

$$ \begin{align*} p-1+2L_{0}= & \, 2_{s}^{*}, \nonumber \\ p-1+2L_{j+1}= & \, 2_{s}^{*}L_{j}, \quad j=0,1,2,\ldots \end{align*} $$

and

$$ \begin{align*} M_{0}=& \, (A\zeta_{k})^{{2_{s}^{*}}/{2}}, \nonumber \\ M_{j+1}=& \, (AL_{j}M_{j})^{{2_{s}^{*}}/{2}}, \quad j=0,1,2,\ldots. \end{align*} $$

Further, define the sequences $\{\lambda _{j}\}$ , $\{\eta _{j}\}$ and $\{\gamma _{j}\}$ as defined earlier in (3-18) and (3-20). From (3-16),

(4-2) $$ \begin{align} \bigg(\int_{\Omega}u_{k}^{2_{s}^{*}L_{j-1}}\,dx\bigg)^{(2_{s}^{*}L_{j-1})} \leq & \, (M_{j}d_{k}^{n/2s} )^{1/(2_{s}^{*}L_{j-1})}. \end{align} $$

Since

$$ \begin{align*}\log(M_{j})=\eta_{j} \leq \gamma_{j},\end{align*} $$

we have

$$ \begin{align*} \frac{\log(M_{j})}{2_{s}^{*}L_{j-1}} \leq \frac{\eta_{j}}{2_{s}^{*}L_{j-1}}. \end{align*} $$

Now,

$$ \begin{align*} \lim_{j \rightarrow \infty} \frac{\eta_{j}}{2_{s}^{*}L_{j-1}}&= \lim_{j \rightarrow \infty}\frac{\big(\tfrac{2_{s}^{*}}{2}\big)^{j}[ \eta_{0}+2C^{*}2_{s}^{*}(2_{s}^{*}-2)^{-2} ]-2C^{*}(2_{s}^{*}-2)^{-1} [j+ 2_{s}^{*}(2_{s}^{*}-2)]}{\tfrac{2_{s}^{*}}{(2_{s}^{*}-2)}\Big [ \big(\tfrac{2_{s}^{*}}{2}\big)^{j}(2_{s}^{*}-p-1)+p-1 \Big ]}\\[3pt] &= \frac{(2_{s}^{*}-2)(\eta_{0}+2C^{*}2_{s}^{*}(2_{s}^{*}-2)^{-2})}{2_{s}^{*}(2_{s}^{*}-p-1)}. \end{align*} $$

Letting $j \rightarrow \infty $ in (4-2),

(4-3) $$ \begin{align} \Vert u_{k}\Vert_{L^{\infty}(\Omega)} \leq e^{a_{1}(\eta_{0}+a_{2})}, \end{align} $$

with $a_{1}$ and $a_{2}$ depending only on $2_{s}^{*},~p$ and $C^{*}.$ Since

$$ \begin{align*} \eta_{0}= \log (M_{0})= \frac{2_{s}^{*}}{2}\log(A\zeta_{k}), \end{align*} $$

as $k \rightarrow \infty ,~\eta _{0}\rightarrow -\infty .$ Thus, in view of (4-3),

$$ \begin{align*} \Vert u_{k}\Vert_{L^{\infty}(\Omega)} \rightarrow 0, \end{align*} $$

which leads to a contradiction to Lemma 3.8.

Proof of Theorem 1.3

First, we show the second part of (1-7).

Case I. $r \geq 2_{s}^{*}={2n}/({n-2s}).$ Let $\{L_{j} \}$ be the sequence defined in (3-13). If $r \in \{2_{s}^{*}L_{j} \}$ , then the second inequality of (1-7) follows from (3-16). So assume that $2_{s}^{*}L_{j}<r< 2_{s}^{*}L_{j+1}$ for some $j \geq 0.$ We have

$$ \begin{align*}r=t2_{s}^{*}L_{j}+(1-t)2_{s}^{*}L_{j+1} \text{ for some } t \in (0,1).\end{align*} $$

Using the Hölder inequality and (3-16),

$$ \begin{align*} \int_{\Omega}u_{d}^{r}\,dx = & \, \int_{\Omega}u_{d}^{t2_{s}^{*}L_{j}+(1-t)2_{s}^{*}L_{j+1}}\,dx, \\ \leq & \, \bigg( \int_{\Omega}u_{d}^{2_{s}^{*}L_{j}}\,dx \bigg)^{t}\bigg( \int_{\Omega}u_{d}^{2_{s}^{*}L_{j+1}}\,dx \bigg)^{1-t} \\ \leq & \, (M_{j-1}d^{n/2s} )^{t}(M_{j}d^{n/2s})^{1-t}\\ = & \, M_{j-1}^{t}M_{j}^{1-t}d^{{n}/{2s}}. \end{align*} $$

Case II. $2 \leq r \leq 2_{s}^{*}$ . We write

$$ \begin{align*}r=2t+(1-t)2_{s}^{*}, \end{align*} $$

for some $t \in [0,1].$ Then, using the Hölder inequality, from (1-6) and (3-16) with $j=0$ ,

$$ \begin{align*} \int_{\Omega}u_{d}^{r}\,dx \leq & \, \bigg( \int_{\Omega}u_{d}^{2}\,dx \bigg)^{t} \bigg( \int_{\Omega}u_{d}^{2_{s}^{*}}\,dx \bigg)^{1-t}\\ \leq & \, C_{0}^{t}M_{0}^{(1-t)}d^{{n}/{2s}}, \end{align*} $$

where the constant $C_{0}$ is independent of $d.$

Case III. $1 \leq r < p+1.$ Integrating both sides of (1-1) and using the condition $\mathcal {N}_{s}u(x)=0$ for $x\in \mathcal {C}\Omega ,$

(4-4) $$ \begin{align} \int_{\Omega}u_{d}\,dx= \int_{\Omega}u_{d}^{p}\,dx. \end{align} $$

It is easy to see that

$$ \begin{align*} p=t+(1-t)(p+1) \quad\text{with } t=\frac{1}{p} \in (0,1). \end{align*} $$

Notice that $p+1 \in (2, 2_{s}^{*}).$ Therefore, using the Hölder inequality and (4-4),

$$ \begin{align*} \int_{\Omega}u_{d}^{p}\,dx \leq & \, \bigg( \int_{\Omega}u_{d}\,dx \bigg)^{t}\bigg( \int_{\Omega}u_{d}^{p+1}\,dx \bigg)^{(1-t)}, \\ \int_{\Omega}u_{d}^{p}\,dx \leq & \, \int_{\Omega}u_{d}^{p+1}\,dx \leq C_{0}d^{{n}/{2s}} \quad (\text{by}\ ({1\text{-}6})), \end{align*} $$

where the constant $C_{0}$ depends only upon $p+1.$

Also, in view of (4-4) and (1-6), we observe that the second inequality of (1-7) holds for $r=1.$ Now, repeating the interpolation between $1$ and $p+1$ , we see that the second inequality of (1-7) holds for all r $\geq 1.$

Case IV. Let $0<r \leq 1.$ Taking $F=u_{d}^{r},\, G=1, \, p=\frac {1}{r}, \, q=\frac {1}{1-r}$ and using the Hölder inequality,

$$ \begin{align*} \int_{\Omega}u_{d}^{r}\,dx \leq \Vert F\Vert_{p}\Vert G\Vert_{q}=\vert\Omega\vert^{1-r} \bigg(\int_{\Omega}u_{d}\,dx \bigg)^{r} \leq \vert\Omega\vert^{1-r} B(1)^{r}d^{{nr}/{2s}}. \end{align*} $$

This proves the second inequality of (1-8).

Now, let us prove the first inequality of (1-7) and (1-8). In view of (3-5) and (4-1),

$$ \begin{align*} \int_{\Omega}u_{d}^{p+1} \geq K_{0}d^{{n}/{2s}}. \end{align*} $$

Since

$$ \begin{align*}\displaystyle \sup_{\Omega}u_{d}(x) \leq C_{1}\quad \text{for some constant } C_{1}>0,\end{align*} $$

we have

$$ \begin{align*} K_{0}d^{{n}/{2s}}\leq \int_{\Omega}u_{d}^{p+1} &= \, \int_{\Omega}(u_{d}^{p+1-r})(u_{d}^{r})\,dx \\ &\leq C_{1}^{p+1-r}\int_{\Omega}u_{d}^{r}\,dx. \end{align*} $$

This implies that

$$ \begin{align*}\int_{\Omega}u_{d}^{r}\,dx \geq K_{0}C_{1}^{r-p-1}d^{{n}/{2s}}, ~r<p+1.\end{align*} $$

For $r>p+1$ , we write $p+1=1+(1-t)r.$ Therefore,

$$ \begin{align*} K_{0}d^{{n}/{2s}}\leq & \, \int_{\Omega}u_{d}^{p+1}\,dx \\ =& \, \int_{\Omega}u_{d}^{1+(1-t)r}\,dx \\ \leq & \, (u_{d}\,dx )^{t}(u_{d}^{r}\,dx )^{1-t} \\ \leq & \, ( B(1)d^{{n}/{2s}} )^{t} ( u_{d}^{r}\,dx )^{1-t}. \end{align*} $$

This yields that

$$ \begin{align*}\int_{\Omega}u_{d}^{r}\,dx \geq (K_{0}B(1)^{-t})^{{1}/({1-t})}d^{{n}/{2s}}.\\[-41pt] \end{align*} $$

5. Proof of Theorem 1.4

In this section, we prove Theorem 1.4. Its proof is intricate and requires some scaling and compactness arguments. We prove the statements of the theorem one by one. Let $z_{d} \in \overline {\Omega } $ be a point of maximum of $u_{d}.$ We approximate $u_{d}$ around $z_{d}$ by a scaled positive radial solution of (1-5). It gives us an upper bound on $c_{d},$ which is closely related to the location of point $z_{d}.$

Step I. We prove that there exists a positive constant $K_{*}$ such that

(5-1) $$ \begin{align} \rho (z_{d}, \partial \Omega) \leq K_{*}d^{{1}/{2s}} .\end{align} $$

If the inequality in (5-1) is not true, then there is a decreasing sequence $d_{j}\downarrow 0$ such that

(5-2) $$ \begin{align} \rho_{j}:=\frac{\rho(z_{j}, \partial \Omega)}{d_{j}^{{1}/{2s}}} \rightarrow +\infty \quad\text{as } j \rightarrow \infty, \end{align} $$

where $z_{j}:=z_{d_{j}}$ is a point of maximum of $u_{d_{j}}$ on $\overline {\Omega }.$ Define

$$ \begin{align*}\phi_{j}(y):=u_{d_{j}}(yd_{j}^{{1}/{2s}}+z_{j}) \quad \text{for } y \in \mathbb{R}^{n}.\end{align*} $$

Since $u_{d}$ is a classical solution of (1-1),

(5-3) $$ \begin{align} (-\Delta)^{s}\phi_{j}+\phi_{j}=\phi_{j}^{p} \quad \text{in } B_{\rho_{j}}, \end{align} $$

and:

1. (1) $ \phi _{j} \in C^{0,2s+\epsilon }(B_{\rho _{j}}),$ when $0<s<\tfrac 12$ , $ 2s+\epsilon <1; $

2. (2) $ \phi _{j} \in C^{1,2s+\epsilon -1}(B_{\rho _{j}}),$ when $\tfrac 12 \leq s <1$ , $2s+\epsilon -1<1.$

First, we claim that the sequence $\{\phi _{j}\}$ contains a convergent subsequence. Let $\{R_{k}\}$ be a monotone increasing sequence of positive numbers with $R_{k} \rightarrow +\infty $ as $k \rightarrow \infty .$ Therefore, we have for each $k,$ there is a number $j_{k}$ such that $4R_{k}< \rho _{j}$ whenever $j \geq j_{k}.$ Since $u_{d} \in L^{\infty }(\mathbb {R}^n) \cap \mathcal {L}_{s}(\mathbb {R}^n),$ we have $ \phi _{{j}} \in L^{\infty }(\mathbb {R}^n) \cap \mathcal {L}_{s}(\mathbb {R}^n)$ for each $j \geq 1.$ Now, we can use [19, Theorem 1.4] to get the following estimates.

For $0<s<\tfrac 12$ , $ 2s+\epsilon <1$ :

1. (i) let $4s+\epsilon <1,$ then

   $$ \begin{align*} \Vert\phi_{{j}}\Vert_{C^{0,4s+\epsilon} (B_{2R_{k}})} \leq C (\Vert\phi_j\Vert_{L^{\infty} (\mathbb{R}^{n})} + \Vert\phi_{j}^{p}-\phi_j\Vert_{C^{0,2s+\epsilon}(B_{4R_{k}})} ); \end{align*} $$

2. (ii) let $1<4s+\epsilon <2,$ then

   $$ \begin{align*} \Vert\phi_{{j}}\Vert_{C^{1,4s+\epsilon-1} (B_{2R_{k}})} \leq C (\Vert\phi_j\Vert_{L^{\infty} (\mathbb{R}^{n})} + \Vert\phi_{j}^{p}-\phi_j\Vert_{C^{0,2s+\epsilon}(B_{4R_{k}})} ); \end{align*} $$

and for $\tfrac 12 \leq s <1$ , $2s+\epsilon -1<1$ :

1. (iii) let $4s+\epsilon -1<1,$ then

   $$ \begin{align*} \Vert\phi_{{j}}\Vert_{C^{1,4s+\epsilon-1} (B_{2R_{k}})} \leq C (\Vert\phi_j\Vert_{L^{\infty} (\mathbb{R}^{n})} + \Vert\phi_{j}^{p}-\phi_j\Vert_{C^{1,2s+\epsilon-1}(B_{4R_{k}})} ); \end{align*} $$

2. (iv) let $1<4s+\epsilon -1<2,$ then

   $$ \begin{align*} \Vert\phi_{{j}}\Vert_{C^{2,4s+\epsilon-1} (B_{2R_{k}})} \leq C (\Vert\phi_j\Vert_{L^{\infty} (\mathbb{R}^{n})} + \Vert\phi_{j}^{p}-\phi_j\Vert_{C^{1,2s+\epsilon-1}(B_{4R_{k}})} ), \end{align*} $$

where the constant $C>0$ is independent of $j.$

Let us recall the inequality (1-6) here:

$$ \begin{align*} d \frac{c_{n,s}}{2} \int_{T(\Omega)} \frac{\vert u_{d}(x)-u_{d}(y)\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}u^{2}\,dx=\int_{\Omega} u_{d}^{p+1} \leq C_{0}d^{{n}/{2s}}, \end{align*} $$

where $C_{0}$ is independent of $d.$ This yields

$$ \begin{align*} \int_{B_{\rho_{j}}} \phi_{j}^{p+1} \leq C_{0}, \end{align*} $$

and

(5-4) $$ \begin{align} \Vert\phi_{j}\Vert_{H^{s}(B_{\rho_{j}})} \leq C_{0} \quad\text{for all } j\geq 1. \end{align} $$

Also, by Theorem 1.3,

$$ \begin{align*} \int_{\Omega} u_{d}^{r} \leq B(r)d^{{n}/{2s}} \quad \text{for all } r \geq 1, \end{align*} $$

which implies that

(5-5) $$ \begin{align} \int_{B_{\rho_{j}}} \phi_{j}^r \leq B(r)\quad\text{for all } j\geq 1 \text{ and } r \geq 1. \end{align} $$

By Lemma 3.4 and Theorem 1.2,

(5-6) $$ \begin{align} \Vert u_{d}\Vert_{L^{\infty}(\mathbb{R}^{n})} \leq C_{1}, \end{align} $$

where the constant $C_{1}$ is independent of the diffusion constant $d.$ So, (5-5), (5-6) and [19, Theorem 1.3] imply that

$$ \begin{align*} \Vert\phi_{j}\Vert_{X_{s}(\overline{B}_{R_{k}})} < C_2 \quad \text{for all } j \geq j_{k}, \end{align*} $$

where the constant $C_2>0$ is independent of j and the space $X_{s}(\overline {B}_{R_{k}})$ is identified with one of the spaces $C^{0,4s+\epsilon }(\overline {B}_{R_{k}})$ , $C^{1,4s+\epsilon -1}(\overline {B}_{R_{k}})$ or $C^{2,4s+\epsilon -1}(\overline {B}_{R_{k}})$ with the same assumptions on s and $\epsilon $ as above. Therefore, $\{\phi _{j}\}$ is a relatively compact set in $X_{s}(\overline {B}_{R_{k}}),$ and hence by the standard diagonal process, we can extract a convergent subsequence of $\{\phi _{j}\},$ which we continue to denote by $\{\phi _{j}\}$ itself such that

$$ \begin{align*} \phi_{j} \rightarrow v \quad \text{in } C^{0, 2s+\epsilon}_{loc}(\mathbb{R}^{n}) \quad\text{when } 0<s<\tfrac{1}{2}, 2s+\epsilon<1 \end{align*} $$

or

$$ \begin{align*} \phi_{j} \rightarrow v \quad \text{in } C^{1, 2s+\epsilon-1}_{loc}(\mathbb{R}^{n}) \quad\text{when } \tfrac{1}{2}<s<1, 2s+\epsilon-1<1 \end{align*} $$

for some $v.$ The limit $v \in C^{0,2s+\epsilon }(\mathbb {R}^{n}) \cap H^{s}(\mathbb {R}^{n})$ when $0<s<\tfrac 12, 2s+\epsilon <1$ or $v \in C^{1,2s+\epsilon -1}(\mathbb {R}^{n}) \cap H^{s}(\mathbb {R}^{n})$ when $\tfrac 12<s<1, 2s+\epsilon -1<1$ follows from (5-4). Consequently,

$$ \begin{align*} \lim_{\vert x\vert\rightarrow \infty}v(x)=0. \end{align*} $$

Using [17, Theorem 1.1], we have $(-\Delta )^{s}\phi _{j}(x) $ converges to $ (-\Delta )^{s}v(x)$ point-wise in $\mathbb {R}^{n}.$ Consequently, we see that the limit v satisfies the equation

$$ \begin{align*} (-\Delta)^{s}v+v=v^{p} \quad \text{in } \mathbb{R}^{n}. \end{align*} $$

Clearly, $v \geq 0$ because each $\phi _{j} \geq 0.$ Since by Lemma 3.8 we have $\phi _{j}(0)=u_{d_{j}}(z_{j})>1$ for each $j \geq 1,$ one can see that $v \not \equiv 0.$

Using Theorem 2.9, one can see that v is radially symmetric and decreasing about some point in $\mathbb {R}^{n}.$ Since

$$ \begin{align*} \nabla v(0)= \lim_{j \rightarrow \infty} \nabla \phi_{j}(0)=0, \end{align*} $$

v is radially symmetric about the origin. Additionally, by Theorem 2.8, v has a power type of decay at infinity, that is,

$$ \begin{align*} v(r)\leq \frac{C_{2}}{r^{n+2s}}, \quad r \geq 1. \end{align*} $$

Now we derive a lower bound on the critical value $c_{d_{j}}.$ Let us define

(5-7) $$ \begin{align} \delta_{R}:= \frac{C_{2}}{R^{n+2s}}, \end{align} $$

where $R>0$ is an arbitrarily large real number. Then, there exists a positive integer $j_{R}$ such that if $j \geq j_{R}$ , then $\rho _{j}\geq 2R$ and

(5-8) $$ \begin{align} \Vert\phi_{j}-v\Vert_{C^{2}(\overline{B}_{2R})} \leq \delta_{R}. \end{align} $$

By Lemma 3.3,

$$ \begin{align*}c_{d_{j}}= M[u_{d_{j}}]=J_{d_{j}}(u_{d_{j}}).\end{align*} $$

Using this fact and (3-6),

$$ \begin{align*} c_{d_{j}}&= \bigg(\frac{1}{2}-\frac{1}{p+1}\bigg)\int_{\Omega}u_{d_{j}}^{p+1}\,dx \\ &\geq \bigg(\frac{1}{2}-\frac{1}{p+1}\bigg) \int_{\vert x-z_{j}\vert<d_{j}^{{1}/{2s}}R}u_{d_{j}}^{p+1}\,dx \nonumber \\ &= d_{j}^{{n}/{2s}} \bigg(\frac{1}{2}-\frac{1}{p+1}\bigg) \int_{\vert y\vert < R} \phi_{j}^{p+1}\,dy. \nonumber \end{align*} $$

Now,

(5-9) $$ \begin{align} c_{d_{j}}=& \, d_{j}^{{n}/{2s}} \bigg(\bigg(\frac{1}{2}-\frac{1}{p+1}\bigg)\int_{B_{R}} v^{p+1}\,dy+ F_{j} \bigg), \end{align} $$

where

$$ \begin{align*}F_{j}:= \bigg(\frac{1}{2}-\frac{1}{p+1}\bigg) \int_{B_{R}}\bigg( \phi_{{j}}^{p+1}- v^{p+1} \bigg)\,dy. \end{align*} $$

By Equation (5-8), we have for all $y \in B_{R},~ j \geq j_{R}$ ,

$$ \begin{align*} \vert \phi_{{j}}^{p+1}- v^{p+1}\vert \leq C \vert\phi_{{j}}-v\vert \leq \delta_{R}, \end{align*} $$

where $C>0$ is some constant. This implies that

$$ \begin{align*} \vert F_{j}\vert \leq \bigg(\frac{1}{2}-\frac{1}{p+1}\bigg)C \vert B_{R}\vert \delta_{R}=C_{3}R^{n}\delta_{R}, \end{align*} $$

where

$$ \begin{align*}C_{3}= \bigg(\frac{1}{2}-\frac{1}{p+1}\bigg)\frac{w_{n}}{n} C\end{align*} $$

and $w_{n}$ denotes the surface area of the unit sphere in $\mathbb {R}^{n}.$ Consequently, (5-9) becomes

(5-10) $$ \begin{align} c_{d_{j}}\geq d_{j}^{{n}/{2s}} \bigg[\bigg(\frac{1}{2}-\frac{1}{p+1}\bigg)\int_{B_{R}} v^{p+1}\,dy-C_{3}R^{n}\delta_{R} \bigg]. \end{align} $$

Now, it is easy to see that

$$ \begin{align*} \bigg(\frac{1}{2}-\frac{1}{p+1}\bigg)\int_{B_{R}} v^{p+1}\,dy= F(v)-\bigg(\frac{1}{2}-\frac{1}{p+1}\bigg)\int_{\vert y\vert>R} v^{p+1}\,dy, \end{align*} $$

where $F(v)$ is defined earlier in (2-2). Simplifying the second term on right-hand side,

$$ \begin{align*} \bigg(\frac{1}{2}-\frac{1}{p+1}\bigg)\int_{\vert y\vert>R} v^{p+1}\,dy&= \, \bigg(\frac{1}{2}-\frac{1}{p+1}\bigg) \int_{R}^{\infty} \frac{r^{n-1}w_{n}}{r^{(n+2s)(p+1)}}\,dr \\ &= \, \bigg(\frac{1}{2}-\frac{1}{p+1}\bigg) \frac{w_{n}}{(n+2s)p+2s}\frac{1}{R^{(n+2s)p+2s}}=\frac{C_{4}}{R^{(n+2s)p+2s}}. \end{align*} $$

Therefore, one can write

(5-11) $$ \begin{align} \bigg(\frac{1}{2}-\frac{1}{p+1}\bigg)\int_{B_{R}} v^{p+1}\,dy = F(v)-\frac{C_{4}}{R^{(n+2s)p+2s}}. \end{align} $$

On combining (5-7), (5-10) and (5-11), we get for $j \geq j_{R},$

(5-12) $$ \begin{align} c_{d_{j}} &\geq d_{j}^{{n}/{2s}}\bigg( F(v)- \frac{C_{4}}{R^{(n+2s)p+2s}}-\frac{C_{2}C_{3}}{R^{2s}} \bigg) \nonumber \\ &\geq d_{j}^{{n}/{2s}}\bigg( F(v)- \frac{C_{5}}{R^{2s}} \bigg) , \end{align} $$

where $C_{5}$ is independent of j and $R.$

Now, we derive an upper bound on the critical value $c_{d_{j}}.$ Without loss of generality, we may assume that the domain $\Omega $ is a subset of $\mathbb {R}^{n}_{+}$ and $0 \in \partial \Omega .$ Given Definition 2.13, let w be the ground state solution of (1-5). Define

$$ \begin{align*} \Omega_{d}&:=\bigg\{\frac{x}{d^{{1}/{2s}}} \mid x\in \Omega \bigg\},\\ w_{d}(x)&:=w\bigg( \frac{x}{d^{{1}/{2s}}}\bigg), \quad\text{for } x\in \mathbb{R}^{n}. \end{align*} $$

Since $w \geq 0$ , this implies that $w_{d} \geq 0$ . Define

$$ \begin{align*}g_{d}(t):=J_{d}(tw_{d}),\quad t \geq 0.\end{align*} $$

Then, by Lemma 3.3, there exists a unique $t_{0}=t_{0}(d)>0$ at which $g_{d}$ attains a maximum. It is easy to see that $t_{0}(d) \rightarrow 1$ as $d \downarrow 0.$ Hence,

$$ \begin{align*} M[w_{d}]&= J_{d}\bigg(t_{0}w_{d}\bigg) \\&= \frac{t_{0}^{2}}{2} \bigg[\frac{dc_{n,s}}{2}\int_{T(\Omega)}\frac{\vert w_{d}(x)-w_{d}(y)\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy+ \int_{\Omega}w_{d}^{2}\,dx\bigg]-\frac{t_{0}^{p+1}}{p+1}\int_{\Omega}w_{d}^{p+1}\,dx \\&= \frac{t_{0}^{2}}{2} \bigg[\frac{dc_{n,s}}{2}\int_{T(\Omega)}\frac{\bigg\vert w\bigg(\dfrac{x}{d^{{1}/{2s}}}\bigg)-w\bigg(\dfrac{y}{d^{{1}/{2s}}}\bigg)\bigg\vert^{2}}{\vert x-y\vert^{n+2s}}\,dx\,dy + \int_{\Omega}w^{2}\bigg(\frac{x}{d^{{1}/{2s}}}\bigg)\,dx\bigg]\\&\quad -\frac{t_{0}^{p+1}}{p+1}\int_{\Omega}w^{p+1}\bigg(\frac{x}{d^{{1}/{2s}}}\bigg)\,dx. \end{align*} $$

The change of variables

$$ \begin{align*} \frac{x}{d^{{1}/{2s}}}=a,\, \frac{y}{d^{{1}/{2s}}}=b, \end{align*} $$

gives us

$$ \begin{align*} M[w_{d}]&= d^{{n}/{2s}} \bigg(\frac{t_{0}^{2}}{2} \bigg[\frac{c_{n,s}}{2}\int_{T(\Omega_{d})}\frac{\vert w(a)-w(b)\vert^{2}}{\vert a-b\vert^{n+2s}}\,da\,db+ \int_{\Omega_{d}}w^{2}\,da\bigg]-\frac{t_{0}^{p+1}}{p+1}\int_{\Omega_{d}}w^{p+1}\,da \bigg) \\&= d^{{n}/{2s}} I_d \end{align*} $$

where $I_{d}$ is the expression

$$ \begin{align*} &\frac{t_{0}^{2}}{2} \bigg[\frac{c_{n,s}}{2}\!\int_{\Omega_{d}}\! \int_{\Omega_{d}}\! \frac{\vert w(a)-w(b)\vert^{2}}{\vert a-b\vert^{n+2s}}\,da\,db+ 2c_{n,s}\int_{\mathcal{C}\Omega_{d}}\! \int_{\Omega_{d}}\! \frac{\vert w(a)-w(b)\vert^{2}}{\vert a-b\vert^{n+2s}}\,da\,db +\! \int_{\Omega_{d}}\!w^{2}\,da\bigg]\\&\quad -\frac{t_{0}^{p+1}}{p+1}\int_{\Omega_{d}}w^{p+1}\,da. \end{align*} $$

Since

$$ \begin{align*} t_{0}(d) \rightarrow 1 \quad\text{as }d\downarrow 0, \end{align*} $$

we get for $I_d$

$$ \begin{align*} &\frac{1}{2} \bigg[\frac{c_{n,s}}{2}\!\int_{\mathbb{R}^{n}_{+}} \!\int_{\mathbb{R}^{n}_{+}}\! \frac{\vert w(a)-w(b)\vert^{2}}{\vert a-b\vert^{n+2s}}\,da\,db+ 2c_{n,s}\!\int_{\mathcal{C} \mathbb{R}^{n}_{+}}\! \int_{\mathbb{R}^{n}_{+}}\! \frac{\vert w(a)-w(b)\vert^{2}}{\vert a-b\vert^{n+2s}}\,da\,db + \!\int_{\mathbb{R}^{n}_{+}}\! w^{2}\,da\bigg]\\&\quad -\frac{1}{p+1}\int_{\mathbb{R}^{n}_{+}}w^{p+1}\,da + o(1)\end{align*} $$

as $d \downarrow 0.$ Further, w being nonnegative and radially symmetric implies that

$$ \begin{align*}\int_{\mathbb{R}^{n}_{+}}w^{2}\,da=\frac{1}{2}\int_{\mathbb{R}^{n}}w^{2}\,da,\,\, \int_{\mathbb{R}^{n}_{+}}w^{p+1}\,da=\frac{1}{2}\int_{\mathbb{R}^{n}}w^{p+1}\,da,\end{align*} $$

$$ \begin{align*}\int_{\mathbb{R}^{n}_{+}} \int_{\mathbb{R}^{n}_{+}} \frac{\vert w(a)-w(b)\vert^{2}}{\vert a-b\vert^{n+2s}}\,da\,db = \frac{1}{4} \int_{\mathbb{R}^{n}} \int_{\mathbb{R}^{n}} \frac{\vert w(a)-w(b)\vert^{2}}{\vert a-b\vert^{n+2s}}\,da\,db,\end{align*} $$

$$ \begin{align*} \int_{\mathcal{C}\mathbb{R}^{n}_{+}} \int_{\mathbb{R}^{n}_{+}} \frac{\vert w(a)-w(b)\vert^{2}}{\vert a-b\vert^{n+2s}}\,da\,db = \frac{1}{4} \int_{\mathbb{R}^{n}} \int_{\mathbb{R}^{n}} \frac{\vert w(a)-w(b)\vert^{2}}{\vert a-b\vert^{n+2s}}\,da\,db.\end{align*} $$

Using these estimates,

$$ \begin{align*} I_{d} &< \frac{1}{2}\bigg( \frac{1}{2} \bigg[ \frac{c_{n,s}}{2}\int_{\mathbb{R}^{n}} \int_{\mathbb{R}^{n}} \frac{\vert w(a)-w(b)\vert^{2}}{\vert a-b\vert^{n+2s}}\,da\,db + \int_{\mathbb{R}^{n}}w^{2}\,da \bigg]- \frac{1}{p+1} \int_{\mathbb{R}^{n}}w^{p+1}\,da\bigg)+ o(1)\\& = \frac{1}{2}F(w)+o(1),\end{align*} $$

as $d \downarrow 0.$ Thus,

$$ \begin{align*} M[w_{d}]=d^{{n}/{2s}}I_{d}< \frac{d^{{n}/{2s}}}{2}F(w)+o(1), \end{align*} $$

as $d\downarrow 0.$ Using part (c) of Theorem 2.12, we have $0<F(w) \leq F(v)$ for any nonnegative nonzero classical solution v of (1-5) and by Lemma 3.3,

$$ \begin{align*}c_{d_{j}} \leq M[w_{d_{j}}]< \frac{d_{j}^{{n}/{2s}}}{2}F(v)\end{align*} $$

for $d_{j}$ sufficiently small. By letting R be sufficiently large in (5-12), we reach a contradiction. This proves (5-1).

Remark 5.1. In the classical case [35], the authors have defined diffeomorphisms, which straighten a boundary portion near $Q \in \partial \Omega .$ Further, using scaling and translations of the least energy solutions $u_{d}$ of (1-3), the classical problem (1-3) gets transferred into a new elliptic equation. Due to the nonlocal nature of the fractional Laplacian and of the boundary condition in our problem, it seems almost impossible to introduce such scaling and translation arguments.

Step II. Now, we claim that $z_{d} \in \partial \Omega .$ Suppose that there is a decreasing sequence $d_{j} \downarrow 0$ such that $z_{d_{j}}:=z_{j} \in \Omega .$ We have from Lemma 1.4 that the sequence $\{z_{j}\}$ converges to some $z \in \partial \Omega .$ Without loss of generality, let us assume that $z=0.$ Define

$$ \begin{align*} \widehat{u}_{j}(x):= \begin{cases} u_{d_{j} }(x)& \, \text{in } \mathbb{R}^{n}_{+}, \\ u_{d_{j} }(x', -x_{n}) & \, \text{in } \mathbb{R}^{n}_{-}, \end{cases} \end{align*} $$

where

$$ \begin{align*}x'=(x_{1},x_{2},\ldots, x_{n-1}),\quad \mathbb{R}^{n}_{+}=\{(x',x_{n}) \mid x_{n} \geq 0 \},\,\,\mathbb{R}^{n}_{-}=\{(x',x_{n}) \mid x_{n} \leq 0 \}. \end{align*} $$

Also, define a scaled function

(5-13) $$ \begin{align} \psi_{j}(y):=\widehat{u}_{j}(yd_{j}^{{1}/{2s}}+z_{j})\quad\text{for } y \in \mathbb{R}^{n}. \end{align} $$

Now, for $z_{j}=(z_{j}^{\prime },z_{jn}),$ we can write $z_{jn}=\alpha _{j}d_{j}^{{1}/{2s}}$ for some $\alpha _{j}>0.$ The sequence $\{\alpha _{j} \} $ is bounded, which follows from Lemma 1.4. Let

$$ \begin{align*} \rho_{j}:=\frac{\rho(z_{j}, \partial \Omega)}{d_{j}^{{1}/{2s}}} , \end{align*} $$

where $\rho (z_{j}, \partial \Omega )$ denotes the distance between $z_{j}$ and $ \partial \Omega .$ One can see easily that the function $\psi _{j}$ satisfies the equation

$$ \begin{align*} (-\Delta)^{s}\psi_{j}(y)+ \psi_{j}(y)= \psi_{j}(y)^{p}+ d_{j}{h}(y) \quad \text{in } B_{\rho_{j}} \end{align*} $$

for some function ${h}$ of $y.$ To see this, let $y \in B_{\rho _{j}}$ , so

(5-14) $$ \begin{align} (-\Delta)^{s}\psi_{j}(y)&=c_{n,s}\ \mathrm{PV} \int_{\mathbb{R}^{n}}\frac{\psi_{j}(y)-\psi_{j}(x)}{\vert y-x\vert^{n+2s}}\,dx= c_{n,s} \lim_{\epsilon \rightarrow 0} \int_{\mathcal{C} B_{\epsilon}(y)}\frac{\psi_{j}(y)-\psi_{j}(x)}{\vert y-x\vert^{n+2s}}\,dx \nonumber \\ &= c_{n,s} \lim_{\epsilon \rightarrow 0} \bigg[ \int_{\mathcal{C} B_{\epsilon}(y)}\frac{\widehat{u}_{j}(yd_{j}^{{1}/{2s}}+z_{j})-\widehat{u}_{j}(xd_{j}^{{1}/{2s}}+z_{j})}{\vert y-x\vert^{n+2s}}\,dx \bigg] \nonumber \\ &= c_{n,s} \lim_{\epsilon \rightarrow 0} \int_{\{x_{n} \geq -\alpha_{j}\}\bigcap \mathcal{C} B_{\epsilon}(y)}\frac{\widehat{u}_{j}(yd_{j}^{{1}/{2s}}+z_{j})-\widehat{u}_{j}(xd_{j}^{{1}/{2s}}+z_{j})}{\vert y-x\vert^{n+2s}}\,dx \nonumber \\ & \quad+ c_{n,s} \lim_{\epsilon \rightarrow 0} \int_{\{x_{n} \leq-\alpha_{j}\}\bigcap \mathcal{C}B_{\epsilon}(y)}\frac{\widehat{u}_{j}(yd_{j}^{{1}/{2s}}+z_{j})-\widehat{u}_{j}(xd_{j}^{{1}/{2s}}+z_{j})}{\vert y-x\vert^{n+2s}}\,dx. \end{align} $$

For $y_{n} \geq -\alpha _{j},$

$$ \begin{align*} &(-\Delta)^{s}\psi_{j}(y)\nonumber\\&= c_{n,s}\lim_{\epsilon \rightarrow 0} \int_{\{x_{n} \geq -\alpha_{j}\}\bigcap \mathcal{C} B_{\epsilon}(y)}\frac{{u}_{{j}}(yd_{j}^{{1}/{2s}}+z_{j})-{u}_{{j}}(xd_{j}^{{1}/{2s}}+z_{j})}{\vert y-x\vert^{n+2s}}\,dx \nonumber \\& \quad+ c_{n,s}\lim_{\epsilon \rightarrow 0} \int_{\{x_{n} \leq-\alpha_{j}\}\bigcap \mathcal{C} B_{\epsilon}(y)}\frac{{u}_{{j}}(yd_{j}^{{1}/{2s}}+z_{j})-{u}_{{j}}(x' d_{j}^{{1}/{2s}}+z_{j}^{\prime}, -(x_{n}+\alpha_{j}d_{j}^{{1}/{2s}}))}{\vert y-x\vert^{n+2s}}\,dx \nonumber \\&= c_{n,s} \lim_{\epsilon \rightarrow 0} \int_{\{x_{n} \geq -\alpha_{j}\} \bigcap \mathcal{C} B_{\epsilon}(y)}\frac{{u}_{{j}}(yd_{j}^{{1}/{2s}}+z_{j})-{u}_{{j}}(xd_{j}^{{1}/{2s}}+z_{j})}{\vert y-x\vert^{n+2s}}\,dx \nonumber \\&\quad + c_{n,s}\lim_{\epsilon \rightarrow 0} \int_{\{x_{n} \leq-\alpha_{j}\}\bigcap \mathcal{C} B_{\epsilon}(y)}\frac{{u}_{{j}}(yd_{j}^{{1}/{2s}}+z_{j})-{u}_{{j}}(x'd_{j}^{{1}/{2s}}+z_{j}^{\prime}, -(x_{n}+\alpha_{j}d_{j}^{{1}/{2s}}))}{\vert y-x\vert^{n+2s}}\,dx \nonumber \\&= c_{n,s} \lim_{\epsilon \rightarrow 0} \int_{\mathcal{C} B_{\epsilon}(y)}\frac{{u}_{{j}}(yd_{j}^{{1}/{2s}}+z_{j})-{u}_{{j}}(xd_{j}^{{1}/{2s}}+z_{j})}{\vert y-x\vert^{n+2s}}\,dx \nonumber \\&\quad + c_{n,s}\lim_{\epsilon \rightarrow 0} \int_{\{x_{n} \leq-\alpha_{j}\} \bigcap \mathcal{C}B_{\epsilon}(y)}\frac{{u}_{{j}}(xd_{j}^{{1}/{2s}}+z_{j}) -{u}_{{j}}(x'd_{j}^{{1}/{2s}}+z_{j}^{\prime}, -(x_{n}+\alpha_{j}d_{j}^{{1}/{2s}}))}{\vert y-x\vert^{n+2s}}\,dx \nonumber \\&= c_{n,s} \lim_{\epsilon \rightarrow 0} \int_{\mathcal{C} B_{\epsilon}(y)}\frac{{u}_{{j}}(yd_{j}^{{1}/{2s}}+z_{j})-{u}_{{j}}(xd_{j}^{{1}/{2s}}+z_{j})}{\vert y-x\vert^{n+2s}}\,dx\nonumber\\&\quad + c_{n,s}\lim_{\epsilon \rightarrow 0} \int_{\{x_{n} \leq-\alpha_{j}\}\bigcap \mathcal{C} B_{\epsilon}(y)}\frac{{u}_{{j}}(xd_{j}^{{1}/{2s}}+z_{j}) -\widehat{u}_{{j}}(xd_{j}^{{1}/{2s}}+z_{j})}{\vert y-x\vert^{n+2s}}\,dx . \end{align*} $$

Making the change of variables

$$ \begin{align*}yd_{j}^{{1}/{2s}}+z_{j}=a \text{ and } xd_{j}^{{1}/{2s}}+z_{j}=b,\end{align*} $$

we get

(5-15) $$ \begin{align} (-\Delta)^{s}\psi_{j}(y)&= d_{j}(-\Delta)^{s}u_{j}(a)+ d_{j} c_{n,s} \lim_{\eta \rightarrow 0} \int_{\{b_{n} \leq 0\} \bigcap \mathcal{C} B_{\eta }(a)}\frac{{u}_{{j}}(b) -\widehat{u}_{{j}}(b)}{\vert a-b\vert^{n+2s}}db \nonumber\\ &= d_{j}(-\Delta)^{s}u_{j}(a)+ d_{j}h(a), \end{align} $$

where

$$ \begin{align*}\eta = \epsilon d_{j}^{{1}/{2s}} \end{align*} $$

and

$$ \begin{align*}h(a)= c_{n,s} \lim_{\eta \rightarrow 0} \int_{\{b_{n} \leq 0\} \bigcap \mathcal{C} B_{\eta }(a)}\frac{{u}_{{j}}(b) -\widehat{u}_{{j}}(b)}{\vert a-b\vert^{n+2s}}\,db.\end{align*} $$

Note that $a \in \Omega .$

Now, consider the case $y_{n} \leq -\alpha _{j}.$ Equation (5-14) becomes

$$ \begin{align*} &(-\Delta)^{s}\psi_{j}(y)= I_1 + I_2 \end{align*} $$

where

$$ \begin{align*}&I_1 = \int_{\{x_{n} \geq -\alpha_{j}\}\bigcap \mathcal{C} B_{\epsilon}(y)}\frac{{u}_{j}(y' d_{j}^{{1}/{2s}}+z_{j}^{\prime}, -(y_{n}d_{j}^{{1}/{2s}}+\alpha_{j}d_{j}^{{1}/{2s}}))-{u}_{j}(xd_{j}^{{1}/{2s}}+z_{j})}{\vert y-x\vert^{n+2s}}\,dx \end{align*} $$

and

(5-16) $$ \begin{align} &I_2 = \int_{\{x_{n} \leq-\alpha_{j}\}\bigcap \mathcal{C} B_{\epsilon}(y)}\frac{{u}_{j}(y' d_{j}^{{1}/{2s}}+z_{j}^{\prime}, -(y_{n}d_{j}^{{1}/{2s}}+\alpha_{j}d_{j}^{{1}/{2s}}))- {u}_{j}(x' d_{j}^{{1}/{2s}}+z_{j}^{\prime}, -(x_{n}d_{j}^{{1}/{2s}}+\alpha_{j}d_{j}^{{1}/{2s}}))}{\vert y-x\vert^{n+2s}}\,dx \end{align} $$

Let us introduce some notation. We write $\widehat {x}= (x',-x_{n})$ , $\widetilde {x}=(\widehat {x}',\widehat {x}_{n})$ and $\widehat {x}_{n}=-x_{n}$ for $x=(x',x_{n}) \in \mathbb {R}^{n}, n>1.$ Using these, let us compute

$$ \begin{align*} I_{2}&= c_{n,s} \lim_{\epsilon \rightarrow 0} \bigg[\int_{\{\widehat{x}_{n} \geq \alpha_{j}\} \bigcap \mathcal{C} B_{\epsilon}(\widehat{y})}\frac{{u}_{j}(\widehat{y}' d_{j}^{{1}/{2s}}+\hat{z}_{j}^{\prime}, \widehat{y}_{n}d_{j}^{{1}/{2s}}+\widehat{\alpha}_{j}d_{j}^{{1}/{2s}})- {u}_{j}(\widehat{x}' d_{j}^{{1}/{2s}}+\widehat{z}_{j}^{\prime}, \widehat{x}_{n}d_{j}^{{1}/{2s}}+\widehat{\alpha}_{j}d_{j}^{{1}/{2s}})}{\vert\widehat{y}-\widehat{x}\vert^{n+2s}}d\widetilde{x} \bigg] \nonumber \\ &= c_{n,s} \lim_{\epsilon \rightarrow 0} \bigg[\int_{\{\widehat{x}_{n} \geq \alpha_{j}\} \bigcap \mathcal{C} B_{\epsilon}(\widehat{y})}\frac{{u}_{j}(\widetilde{y}d_{j}^{{1}/{2s}}+\widetilde{z}_{j})- {u}_{j}(\widetilde{x}d_{j}^{{1}/{2s}}+\widetilde{z}_{j} )}{\vert\widehat{y}-\widehat{x}\vert^{n+2s}}d\widetilde{x} \bigg] \nonumber\\ &= c_{n,s} \lim_{\epsilon \rightarrow 0} \bigg[\int_{\{\widehat{x}_{n} \geq \alpha_{j}\} \bigcap \mathcal{C} B_{\epsilon}(\widetilde{y})}\frac{{u}_{j}(\widetilde{y}d_{j}^{{1}/{2s}}+\widetilde{z}_{j})- {u}_{j}(\widetilde{x}d_{j}^{{1}/{2s}}+\widetilde{z}_{j} )}{\vert\widetilde{y}-\widetilde{x}\vert^{n+2s}}d\widetilde{x} \bigg]. \end{align*} $$

Now, we simplify $I_{1}$ :

$$ \begin{align*} I_{1}&= c_{n,s} \lim_{\epsilon \rightarrow 0} \bigg[ \int_{\{x_{n} \geq -\alpha_{j}\} \bigcap \mathcal{C} B_{\epsilon}({y})}\!\!\frac{{u}_{j}(y' d_{j}^{{1}/{2s}}+z_{j}^{\prime}, -(y_{n}d_{j}^{{1}/{2s}}+\alpha_{j}d_{j}^{{1}/{2s}}))- {u}_{j}(x' d_{j}^{{1}/{2s}}+z_{j}^{\prime}, -(x_{n}d_{j}^{{1}/{2s}}+\alpha_{j}d_{j}^{{1}/{2s}}))}{\vert y-x\vert^{n+2s}} \nonumber \\ & \quad+ \int_{\{x_{n} \geq -\alpha_{j}\} \cap \mathcal{C} B_{\epsilon}({y})} \frac{ {u}_{j}(w'd_{j}^{{1}/{2s}}+z_{j}^{\prime}, -(x_{n}d_{j}^{{1}/{2s}}+\alpha_{j}d_{j}^{{1}/{2s}}))-{u}_{j}(xd_{j}^{{1}/{2s}}+z_{j})}{\vert y-x\vert^{n+2s}}\,dx \bigg] \nonumber \\ &= c_{n,s}\lim_{\epsilon \rightarrow 0} \bigg[ \int_{\{\widehat{x}_{n} \leq \alpha_{j}\} \bigcap \mathcal{C}B_{\epsilon}(\widetilde{y})}\frac{{u}_{j}(\widetilde{y}d_{j}^{{1}/{2s}}+\widetilde{z}_{j})- {u}_{j}(\widetilde{x}d_{j}^{{1}/{2s}}+\widetilde{z}_{j} )}{\vert\widetilde{y}-\widetilde{x}\vert^{n+2s}}d\widetilde{x}\nonumber\\ &\quad+ \int_{\{{x}_{n} \geq -\alpha_{j}\} \cap \mathcal{C}B_{\epsilon}(\widetilde{y})}\frac{{u}_{j}(\widetilde{x}d_{j}^{{1}/{2s}}+\widetilde{z}_{j})- \widehat{{u}}_{j}(\widetilde{x}d_{j}^{{1}/{2s}}+\widetilde{z}_{j} )}{\vert\widetilde{y}-\widetilde{x}\vert^{n+2s}}d\widetilde{x} \bigg]. \end{align*} $$

Using these estimates for $I_{1}$ and $I_{2}$ in (5-16),

$$ \begin{align*} (-\Delta)^{s}\psi_{j}(y)&= c_{n,s}\ \mathrm{PV} \int_{\mathbb{R}^{n}}\frac{{u}_{j}(\widetilde{y}d_{j}^{{1}/{2s}}+\widetilde{z}_{j})- {u}_{j}(\widetilde{x}d_{j}^{{1}/{2s}}+\widetilde{z}_{j} )}{\vert\widetilde{y}-\widetilde{x}\vert^{n+2s}}d\widetilde{x}\nonumber\\ &\quad+ c_{n,s} \lim_{\epsilon \rightarrow 0} \int_{\{{x}_{n} \geq -\alpha_{j}\} \bigcap \mathcal{C}B_{\epsilon}(\widetilde{y})}\frac{{u}_{j}(\widetilde{x}d_{j}^{{1}/{2s}}+\widetilde{z}_{j})- \widehat{{u}}_{j}(\widetilde{x}d_{j}^{{1}/{2s}}+\widetilde{z}_{j} )}{\vert\widetilde{y}-\widetilde{x}\vert^{n+2s}}d\widetilde{x}. \end{align*} $$

By the change of variables

$$ \begin{align*}\widetilde{y}d_{j}^{{1}/{2s}}+\widetilde{z}_{j}=e \quad\text{and}\quad \widetilde{w}d_{j}^{{1}/{2s}}+\widetilde{z}_{j}=f,\end{align*} $$

we get

(5-17) $$ \begin{align} &(-\Delta)^{s}\psi_{j}(y)= d_{j}(-\Delta)^{s}u_{j}(e)\nonumber\\ &\qquad+ d_{j} c_{n,s} \lim_{\eta \rightarrow 0} \int_{\{f_{n} \leq 0\}\bigcap \mathcal{C}B_{\eta}(e)}\frac{{u}_{{j}}(f) -\widehat{u}_{{j}}(f)}{\vert e-f\vert^{n+2s}}\,df, \,\mbox{where} f_n \mbox{ is the } n\,\mbox{th coordinate of } f \nonumber \\ &\quad= d_{j}(-\Delta)^{s}u_{j}(e) + d_{j}h(e). \end{align} $$

Note that $e \in \Omega .$ Further, for $y \in B_{\rho _{j}},$

$$ \begin{align*} \psi_{j}(y)= \widehat{u}_{j}(yd_{j}^{{1}/{2s}}+ z_{j})= \begin{cases} u_{d_{j} }(yd_{j}^{{1}/{2s}}+z_{j})& \text{if } y_{n} \geq - \alpha_{j}, \\ u_{d_{j} }(y' d_{j}^{{1}/{2s}}+z_{j}^{\prime}, -(y_{n}d_{j}^{{1}/{2s}}+ \alpha_{j}d_{j}^{{1}/{2s}})) & \text{if } y_{n} \leq -\alpha_{j}. \end{cases} \end{align*} $$

We can write

$$ \begin{align*}(y' d_{j}^{{1}/{2s}}+z_{j}^{\prime}, -(y_{n}d_{j}^{{1}/{2s}}+ \alpha_{j}d_{j}^{{1}/{2s}}))=\widetilde{y}d_{j}^{{1}/{2s}}+\widetilde{z}_{j}.\end{align*} $$

Again re-naming the variables $yd_{j}^{{1}/{2s}}+ z_{j}$ and $\widetilde {y}d_{j}^{{1}/{2s}}+\widetilde {z}_{j}$ by a and $e,$ respectively,

$$ \begin{align*} \psi_{j}(y)= \begin{cases} u_{d_{j} }(a)& \text{if } y_{n} \geq -\alpha_{j}, \\ u_{d_{j} }(e) & \text{if } y_{n} \leq -\alpha_{j}. \end{cases} \end{align*} $$

We know that $u_{j}$ satisfies (1-1) in the point-wise sense as well. Therefore, combining above equation with (5-15), (5-17), we have for $y \in B_{\rho _{j}}, $

$$ \begin{align*} (-\Delta)^{s}\psi_{j}(y)+\psi_{j}(y)=\psi_{j}(y)^{p}+ d_{j}{h}(y). \end{align*} $$

Now, arguing as in the proof of Step I with minor modifications, one can obtain a convergent subsequence of $\{\psi _{j} \},$ which we denote again by $\{\psi _{j} \}$ such that $\psi _{j} \rightarrow v$ in $C_{loc}^{2}(\mathbb {R}^{n}).$ Therefore, as $d_{j}\downarrow 0,$

$$ \begin{align*} (-\Delta)^{s}v+ v= v^{p}\quad \text{in } \mathbb{R}^{n}. \end{align*} $$

Since $v \in H^{s}(\mathbb {R}^{n})$ and v is radially decreasing, v is spherically symmetric to $y=0.$ Moreover, v has power-type decay at infinity, which follows from Theorem 2.8, that is,

$$ \begin{align*} v(r) \leq \frac{C_{2}}{r^{n+2s}}, \quad r \geq 1, \end{align*} $$

for some constant $C_{2}>0.$ Let us define $\delta _{R}$ as in (5-7), that is,

$$ \begin{align*}\delta_{R}:= \frac{C_{2}}{R^{n+2s}}\end{align*} $$

for R sufficiently large to be defined later. Then, there exists an integer $j_{R}$ such that for $j \geq j_{R},$

(5-18) $$ \begin{align} \Vert\psi_{j}-v\Vert_{C^{2}(\overline{B_{4R}})} \leq \delta_{R}. \end{align} $$

We choose R sufficiently large that $R> \alpha _{j}$ for all $j,$ where the $\alpha _{j}$ terms are the same as defined earlier right after (5-13). We can choose such an R because $\{\alpha _{j}\}$ is a bounded sequence. The following lemma is very useful to prove our claim that $z_{d} \in \partial \Omega .$

Lemma 5.2 (see [35, Lemma 4.2])

Let $f \in C^{2}(\overline {B_{t}})$ be a radial function. Assume that f satisfies $f'(0)=0$ and $f"(r)<0$ for $0 \leq r \leq t.$ Then, there exists a $\eta> 0$ such that if $g \in C^{2}(\overline {B_{t}}) $ satisfies:

1. (1) $\nabla g(0)=0$ ;

2. (2) $ \Vert f-g\Vert _{C^{2}(\overline {B_{t}})}< \eta ,$

then $\nabla g \neq 0$ for $x \neq 0.$

Now, we use this lemma to show that $\psi _{j}$ has only one local maximum point in $B_{R}.$ For this, we choose two numbers $k,l$ $(0<k<l)$ such that $v"(r)<0 $ for $0 \leq r \leq k.$ Further, we see that $v"(0)<0$ and $ v(k)<1.$ Let us define

$$ \begin{align*} \theta = \min \{ \vert v'(r)\vert \mid k \leq r \leq l \}. \end{align*} $$

It is easy to observe that $\theta>0$ because $v'<0$ for $r>0.$ Then for $\delta _{R}<\theta ,$ we have by (5-18) that

$$ \begin{align*} 0< \theta - \delta_{R} \leq \vert\nabla v(y)\vert -\vert\nabla \psi_{j}(y)-\nabla v(y)\vert \leq \vert\nabla \psi_{j}(y)\vert \text{ for } k \leq \vert y\vert \leq l. \end{align*} $$

Applying Lemma 5.2 in the ball $\overline {B}_{k},$ we conclude that $y=0$ is the only local maximum point of $\psi _{j}$ in $B_{l}.$ If $y_{j}$ is a maximum point of $\psi _{j}$ in $B_{R},$ then by Lemma 3.8, we have $\psi _{j} \geq 1.$ Choose $R>0$ sufficiently large so that $\delta _{R}< 1-v(l).$ Therefore,

$$ \begin{align*} \psi_{j}(y) \leq v(y) + \delta_{R} \leq v(l)+ \delta_{R}<1. \end{align*} $$

Hence, $y_{j} \in B_{l}$ and therefore $y_{j}=0.$

If $\alpha _{j}>0,$ then by the definition of $\widehat {u}_{j}$ , $z_{R}^{*}=(z_{j}^{\prime }, -\alpha _{j}d_{j}^{{1}/{2s}})$ is also a maximum point of $\widehat {u}_{j}.$ This implies that $(0,-\alpha _{j})$ is another maximum point of $\psi _{j}$ in $B_{R},$ which is a contradiction. This proves our claim and hence completes the proof of Theorem 1.4.