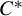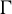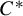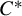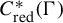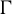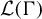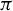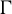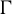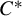1 Introduction
Given a discrete group
![]() $\Gamma $
and a subgroup
$\Gamma $
and a subgroup
![]() ${\Lambda }\leq \Gamma $
, we have a canonical inclusion of the reduced group
${\Lambda }\leq \Gamma $
, we have a canonical inclusion of the reduced group
![]() $C^*$
-algebras
$C^*$
-algebras
![]() $C^*_{\mathrm {red}}({\Lambda })\subseteq C^*_{\mathrm {red}}(\Gamma )$
, and the characteristic function
$C^*_{\mathrm {red}}({\Lambda })\subseteq C^*_{\mathrm {red}}(\Gamma )$
, and the characteristic function
![]() $\mathbf {1}_{\Lambda }$
of
$\mathbf {1}_{\Lambda }$
of
![]() ${\Lambda }$
extends to a conditional expectation
${\Lambda }$
extends to a conditional expectation
![]() $\mathcal {E}_\Lambda : C^*_{\mathrm {red}}(\Gamma )\to C^*_{\mathrm {red}}(\Lambda )$
. Considering the canonical action of
$\mathcal {E}_\Lambda : C^*_{\mathrm {red}}(\Gamma )\to C^*_{\mathrm {red}}(\Lambda )$
. Considering the canonical action of
![]() $\Gamma $
on
$\Gamma $
on
![]() $C^*_{\mathrm {red}}(\Gamma )$
by unitary conjugations, one can observe that
$C^*_{\mathrm {red}}(\Gamma )$
by unitary conjugations, one can observe that
![]() $\Lambda $
is normal in
$\Lambda $
is normal in
![]() $\Gamma $
if and only if
$\Gamma $
if and only if
![]() $C^*_{\mathrm {red}}(\Lambda )$
is
$C^*_{\mathrm {red}}(\Lambda )$
is
![]() $\Gamma $
-invariant inside
$\Gamma $
-invariant inside
![]() $C^*_{\mathrm {red}}(\Gamma )$
, or equivalently if the conditional expectation
$C^*_{\mathrm {red}}(\Gamma )$
, or equivalently if the conditional expectation
![]() $\mathcal {E}_\Lambda $
is
$\mathcal {E}_\Lambda $
is
![]() $\Gamma $
-equivariant. Similar observations hold for the inclusion
$\Gamma $
-equivariant. Similar observations hold for the inclusion
![]() $\mathcal {L}({\Lambda })\subset \mathcal {L}(\Gamma )$
of the corresponding group von Neumann algebras.
$\mathcal {L}({\Lambda })\subset \mathcal {L}(\Gamma )$
of the corresponding group von Neumann algebras.
In this sense,
![]() $\Gamma $
-invariant unital
$\Gamma $
-invariant unital
![]() $C^*$
-subalgebras of
$C^*$
-subalgebras of
![]() $C^*_{\mathrm {red}}(\Gamma )$
and
$C^*_{\mathrm {red}}(\Gamma )$
and
![]() $\Gamma $
-invariant von Neumann subalgebras of
$\Gamma $
-invariant von Neumann subalgebras of
![]() $\mathcal {L}(\Gamma )$
can be viewed as ‘non-commutative normal subgroups’ of
$\mathcal {L}(\Gamma )$
can be viewed as ‘non-commutative normal subgroups’ of
![]() $\Gamma $
. Similarly,
$\Gamma $
. Similarly,
![]() $\Gamma $
-equivariant conditional expectations on
$\Gamma $
-equivariant conditional expectations on
![]() $C^*_{\mathrm {red}}(\Gamma )$
or
$C^*_{\mathrm {red}}(\Gamma )$
or
![]() $\mathcal {L}(\Gamma )$
can be considered as alternative non-commutative generalizations of normal subgroups of
$\mathcal {L}(\Gamma )$
can be considered as alternative non-commutative generalizations of normal subgroups of
![]() $\Gamma $
.
$\Gamma $
.
This note is concerned with these objects. We are mostly interested in the case of lattices
![]() $\Gamma $
in higher rank semisimple groups, where some of the most prominent results (e.g., Margulis’ normal subgroup theorem [Reference MargulisMar91], Stuck–Zimmer’s rigidity theorem [Reference Stuck and ZimmerSZ94], character rigidity [Reference Bader, Boutonnet, Houdayer and PetersonBBHP20, Reference BekkaBek07, Reference Boutonnet and HoudayerBH21, Reference PetersonPet15]) are concerned with rigidity properties of their normal subgroups and various generalized objects. In this context, the above considerations naturally lead us to the questions of to what extent, and in what sense, those rigidity results can be extended to the non-commutative setting.
$\Gamma $
in higher rank semisimple groups, where some of the most prominent results (e.g., Margulis’ normal subgroup theorem [Reference MargulisMar91], Stuck–Zimmer’s rigidity theorem [Reference Stuck and ZimmerSZ94], character rigidity [Reference Bader, Boutonnet, Houdayer and PetersonBBHP20, Reference BekkaBek07, Reference Boutonnet and HoudayerBH21, Reference PetersonPet15]) are concerned with rigidity properties of their normal subgroups and various generalized objects. In this context, the above considerations naturally lead us to the questions of to what extent, and in what sense, those rigidity results can be extended to the non-commutative setting.
The main result of this paper is that irreducible lattices in higher rank semisimple Lie groups
![]() $\Gamma $
have no genuine ‘non-commutative normal subgroups’.
$\Gamma $
have no genuine ‘non-commutative normal subgroups’.
Theorem 1.1. Assume G is a connected semisimple Lie group with trivial center, no non-trivial compact factors, and such that all its simple factors have real rank at least two. Let
![]() $\Gamma < G$
be an irreducible lattice. Then, any
$\Gamma < G$
be an irreducible lattice. Then, any
![]() $\Gamma $
-invariant von Neumann subalgebra of
$\Gamma $
-invariant von Neumann subalgebra of
![]() $\mathcal {L}(\Gamma )$
is of the form
$\mathcal {L}(\Gamma )$
is of the form
![]() $\mathcal {L}({\Lambda })$
for some normal subgroup
$\mathcal {L}({\Lambda })$
for some normal subgroup
![]() ${\Lambda }\le \Gamma $
.
${\Lambda }\le \Gamma $
.
The work of Alekseev and Brugger [Reference Alekseev and BruggerAB21] and Brugger’s thesis [Reference BruggerBru18] seem to be the first systematic study of
![]() $\Gamma $
-invariant von Neumann subalgebras of
$\Gamma $
-invariant von Neumann subalgebras of
![]() $\mathcal {L}(\Gamma )$
, where the authors proved that for
$\mathcal {L}(\Gamma )$
, where the authors proved that for
![]() $\Gamma $
as in Theorem 1.1 (with the additional assumption of G being simple), the inclusion
$\Gamma $
as in Theorem 1.1 (with the additional assumption of G being simple), the inclusion
![]() $\textsf {M}\subset \mathcal {L}(\Gamma )$
of any non-trivial
$\textsf {M}\subset \mathcal {L}(\Gamma )$
of any non-trivial
![]() $\Gamma $
-invariant subfactor
$\Gamma $
-invariant subfactor
![]() $\textsf {M}$
of
$\textsf {M}$
of
![]() $\mathcal {L}(\Gamma )$
has finite (Jones) index (see also [Reference Chifan and DasCD20, §3] for some related results).
$\mathcal {L}(\Gamma )$
has finite (Jones) index (see also [Reference Chifan and DasCD20, §3] for some related results).
Recall that if
![]() ${\Lambda }$
is a subgroup of
${\Lambda }$
is a subgroup of
![]() $\Gamma $
, then
$\Gamma $
, then
![]() ${\Lambda }$
has finite index in
${\Lambda }$
has finite index in
![]() $\Gamma $
if and only if
$\Gamma $
if and only if
![]() $\mathcal {L}({\Lambda })$
has finite index in
$\mathcal {L}({\Lambda })$
has finite index in
![]() $\mathcal {L}(\Gamma )$
. Hence, their result can be considered as a non-commutative version of Margulis’ normal subgroup theorem (NST) [Reference MargulisMar91], which states that every group
$\mathcal {L}(\Gamma )$
. Hence, their result can be considered as a non-commutative version of Margulis’ normal subgroup theorem (NST) [Reference MargulisMar91], which states that every group
![]() $\Gamma $
as in Theorem 1.1 is just-infinite, meaning that every non-trivial normal subgroup of
$\Gamma $
as in Theorem 1.1 is just-infinite, meaning that every non-trivial normal subgroup of
![]() $\Gamma $
has finite index in
$\Gamma $
has finite index in
![]() $\Gamma $
.
$\Gamma $
.
In the
![]() $C^*$
-algebra setting, we prove a complete description of equivariant conditional expectations as follows. We denote by
$C^*$
-algebra setting, we prove a complete description of equivariant conditional expectations as follows. We denote by
![]() $\unicode{x3bb} _\Gamma $
the left regular representation of
$\unicode{x3bb} _\Gamma $
the left regular representation of
![]() $\Gamma $
.
$\Gamma $
.
Theorem 1.2. Let
![]() $\Gamma $
be as in the statement of Theorem 1.1, and let
$\Gamma $
be as in the statement of Theorem 1.1, and let
![]() $\pi $
be a weakly mixing unitary representation of
$\pi $
be a weakly mixing unitary representation of
![]() $\Gamma $
. If
$\Gamma $
. If
![]() $\mathcal {E}$
is a
$\mathcal {E}$
is a
![]() $\Gamma $
-equivariant conditional expectation on
$\Gamma $
-equivariant conditional expectation on
![]() $C^*_{\pi }(\Gamma )$
, then there is a normal subgroup
$C^*_{\pi }(\Gamma )$
, then there is a normal subgroup
![]() ${\Lambda }\le \Gamma $
such that
${\Lambda }\le \Gamma $
such that
![]() $\mathcal {E}|_{\pi (\Gamma )}=\mathbf {1}_{\pi ({\Lambda })}$
.
$\mathcal {E}|_{\pi (\Gamma )}=\mathbf {1}_{\pi ({\Lambda })}$
.
Here, by a conditional expectation on a unital
![]() $C^*$
-algebra
$C^*$
-algebra
![]() $\textsf {B}$
, we mean a unital completely positive (ucp) idempotent map
$\textsf {B}$
, we mean a unital completely positive (ucp) idempotent map
![]() $\mathcal {E}: \textsf {B}\to \textsf {B}$
whose range is a
$\mathcal {E}: \textsf {B}\to \textsf {B}$
whose range is a
![]() $C^*$
-subalgebra of
$C^*$
-subalgebra of
![]() $\textsf {B}$
, and the action of
$\textsf {B}$
, and the action of
![]() $\Gamma $
on
$\Gamma $
on
![]() $C^*_{\pi }(\Gamma )$
is by conjugations with unitaries
$C^*_{\pi }(\Gamma )$
is by conjugations with unitaries
![]() $\pi (g)$
,
$\pi (g)$
,
![]() $g\in \Gamma $
.
$g\in \Gamma $
.
Recall that a unitary representation is called weakly mixing if it does not have any finite-dimensional subrepresentations. Let us point out that the weakly mixing condition is rather non-restrictive. In particular, a unitary representation of a property
![]() $(\rm {T})$
group is weakly mixing if and only if
$(\rm {T})$
group is weakly mixing if and only if
![]() $\pi $
is non-amenable in the sense of Bekka (see [Reference BekkaBek90, Reference Bekka and ValetteBV93]). Examples of non-amenable representations
$\pi $
is non-amenable in the sense of Bekka (see [Reference BekkaBek90, Reference Bekka and ValetteBV93]). Examples of non-amenable representations
![]() $\pi $
of groups
$\pi $
of groups
![]() $\Gamma $
as in the statement of Theorem 1.2 include, for instance, the quasi-regular representation of
$\Gamma $
as in the statement of Theorem 1.2 include, for instance, the quasi-regular representation of
![]() $\Gamma $
on
$\Gamma $
on
![]() $\ell ^2(\Gamma /{\Lambda })$
for any subgroups
$\ell ^2(\Gamma /{\Lambda })$
for any subgroups
![]() ${\Lambda }\le \Gamma $
of infinite index, and the Koopman representation of
${\Lambda }\le \Gamma $
of infinite index, and the Koopman representation of
![]() $\Gamma $
on
$\Gamma $
on
![]() $L^2(X, \nu )$
for any
$L^2(X, \nu )$
for any
![]() $\Gamma $
-quasi-invariant measure on a compact minimal
$\Gamma $
-quasi-invariant measure on a compact minimal
![]() $\Gamma $
-space admitting no
$\Gamma $
-space admitting no
![]() $\Gamma $
-invariant probability measure (e.g., any topological
$\Gamma $
-invariant probability measure (e.g., any topological
![]() $\Gamma $
-boundary X).
$\Gamma $
-boundary X).
Since every von Neumann subalgebra of a finite von Neumann algebra is the image of a unique trace-preserving conditional expectation, Theorem 1.1 can be equivalently stated as a rigidity result for conditional expectations on
![]() $\mathcal {L}(\Gamma )$
.
$\mathcal {L}(\Gamma )$
.
Theorem 1.3. Let
![]() $\Gamma $
be as in the statement of Theorem 1.1. If
$\Gamma $
be as in the statement of Theorem 1.1. If
![]() $\mathcal {E}$
is an ultraweakly continuous
$\mathcal {E}$
is an ultraweakly continuous
![]() $\Gamma $
-equivariant conditional expectation on
$\Gamma $
-equivariant conditional expectation on
![]() $\mathcal {L}(\Gamma )$
, then there is a normal subgroup
$\mathcal {L}(\Gamma )$
, then there is a normal subgroup
![]() ${\Lambda }\le \Gamma $
such that
${\Lambda }\le \Gamma $
such that
![]() $\mathcal {E}|_{\unicode{x3bb} _{\Gamma }(\Gamma )}=\mathbf {1}_{\unicode{x3bb} _{\Gamma }({\Lambda })}$
.
$\mathcal {E}|_{\unicode{x3bb} _{\Gamma }(\Gamma )}=\mathbf {1}_{\unicode{x3bb} _{\Gamma }({\Lambda })}$
.
In contrast to the von Neumann algebra case, in general, even for groups
![]() $\Gamma $
with the infinite conjugacy class (icc) property, it is not the case that any
$\Gamma $
with the infinite conjugacy class (icc) property, it is not the case that any
![]() $\Gamma $
-invariant unital
$\Gamma $
-invariant unital
![]() $C^*$
-subalgebra
$C^*$
-subalgebra
![]() $\textsf {A}$
of
$\textsf {A}$
of
![]() $C^*_{\mathrm {red}}(\Gamma )$
is the image of some conditional expectation (see Example 3.2). In particular, Theorem 1.2 does not offer a complete description of all
$C^*_{\mathrm {red}}(\Gamma )$
is the image of some conditional expectation (see Example 3.2). In particular, Theorem 1.2 does not offer a complete description of all
![]() $\Gamma $
-invariant unital
$\Gamma $
-invariant unital
![]() $C^*$
-subalgebras
$C^*$
-subalgebras
![]() $\textsf {A}$
of
$\textsf {A}$
of
![]() $C^*_{\mathrm {red}}(\Gamma )$
, although in the case of these lattices, we do not know of any concrete example of such
$C^*_{\mathrm {red}}(\Gamma )$
, although in the case of these lattices, we do not know of any concrete example of such
![]() $\textsf {A}$
that is not the image of an equivariant conditional expectation of
$\textsf {A}$
that is not the image of an equivariant conditional expectation of
![]() $C^*_{\mathrm {red}}(\Gamma )$
.
$C^*_{\mathrm {red}}(\Gamma )$
.
We do however prove, in Theorem 1.5 below, a co-finiteness result for general
![]() $\Gamma $
-invariant unital
$\Gamma $
-invariant unital
![]() $C^*$
-subalgebras of
$C^*$
-subalgebras of
![]() $C^*_{\mathrm { red}}(\Gamma )$
, where
$C^*_{\mathrm { red}}(\Gamma )$
, where
![]() $\Gamma $
is a lattice as in Theorem 1.1.
$\Gamma $
is a lattice as in Theorem 1.1.
The co-finiteness of
![]() $C^*$
-subalgebras in our result is in the following sense.
$C^*$
-subalgebras in our result is in the following sense.
Definition 1.4. Let
![]() $\pi $
be a unitary representation of the group
$\pi $
be a unitary representation of the group
![]() $\Gamma $
.
$\Gamma $
.
A
![]() $\Gamma $
-invariant von Neumann subalgebra
$\Gamma $
-invariant von Neumann subalgebra
![]() $\textsf {N}$
of
$\textsf {N}$
of
![]() $\pi (\Gamma )^{\prime \prime }$
is said to be co-finite in
$\pi (\Gamma )^{\prime \prime }$
is said to be co-finite in
![]() $\pi (\Gamma )^{\prime \prime }$
if the commutant
$\pi (\Gamma )^{\prime \prime }$
if the commutant
![]() ${\textsf {N}}^\prime $
of
${\textsf {N}}^\prime $
of
![]() $\textsf {N}$
in
$\textsf {N}$
in
![]() $B(H_\pi )$
admits an ultraweakly continuous
$B(H_\pi )$
admits an ultraweakly continuous
![]() $\Gamma $
-invariant state (commutants are taken in
$\Gamma $
-invariant state (commutants are taken in
![]() $B(H_\pi )$
).
$B(H_\pi )$
).
A
![]() $\Gamma $
-invariant unital
$\Gamma $
-invariant unital
![]() $C^*$
-subalgebra
$C^*$
-subalgebra
![]() $\textsf {A}$
of
$\textsf {A}$
of
![]() $C^*_\pi (\Gamma )$
is said to be co-finite in
$C^*_\pi (\Gamma )$
is said to be co-finite in
![]() $C^*_\pi (\Gamma )$
if the double commutant
$C^*_\pi (\Gamma )$
if the double commutant
![]() ${\textsf {A}}^{\prime \prime }$
is co-finite in
${\textsf {A}}^{\prime \prime }$
is co-finite in
![]() $\pi (\Gamma )^{\prime \prime }$
.
$\pi (\Gamma )^{\prime \prime }$
.
Theorem 1.5. Let
![]() $\Gamma $
be as in the statement of Theorem 1.1. Then, for every non-trivial unital
$\Gamma $
be as in the statement of Theorem 1.1. Then, for every non-trivial unital
![]() $\Gamma $
-invariant
$\Gamma $
-invariant
![]() $C^*$
-subalgebra
$C^*$
-subalgebra
![]() $\textsf {A}$
of
$\textsf {A}$
of
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
and every unitary representation
$C^{*}_{\mathrm {red}}(\Gamma )$
and every unitary representation
![]() $\pi $
of
$\pi $
of
![]() $\Gamma $
which is weakly contained in
$\Gamma $
which is weakly contained in
![]() $\unicode{x3bb} _\Gamma $
,
$\unicode{x3bb} _\Gamma $
,
![]() $\pi (\textsf {A})$
is co-finite in
$\pi (\textsf {A})$
is co-finite in
![]() $C^*_\pi (\Gamma )$
.
$C^*_\pi (\Gamma )$
.
We also prove a von Neumann algebraic version of Theorem 1.5, recorded as Theorem 2.17, which will be a key ingredient in the proof of Theorem 1.1.
The conclusion of Theorem 1.5 is indeed a non-commutative just-infiniteness for
![]() $\Gamma $
(see Definition 2.13). Obviously, any group
$\Gamma $
(see Definition 2.13). Obviously, any group
![]() $\Gamma $
that satisfies the conclusion of Theorem 1.5 is just-infinite, but not conversely: we will give examples of simple non-amenable groups
$\Gamma $
that satisfies the conclusion of Theorem 1.5 is just-infinite, but not conversely: we will give examples of simple non-amenable groups
![]() $\Gamma $
for which the conclusion of Theorem 1.5 does not hold (see Example 2.15). In this sense, the above theorem is a sharper result than Margulis’ NST for the lattices
$\Gamma $
for which the conclusion of Theorem 1.5 does not hold (see Example 2.15). In this sense, the above theorem is a sharper result than Margulis’ NST for the lattices
![]() $\Gamma $
as in the statement.
$\Gamma $
as in the statement.
We close this introduction by pointing out that a different concept of just-infiniteness for
![]() $C^*$
-algebras has been studied by Grigorchuk, Musat and Rørdam [Reference Grigorchuk, Musat and RørdamGMR18].
$C^*$
-algebras has been studied by Grigorchuk, Musat and Rørdam [Reference Grigorchuk, Musat and RørdamGMR18].
1.1 New developments
Several new developments have occurred since the this version of the paper appeared in February 2022. In [Reference Amrutam and JiangAJ22], the authors prove a similar result as our Theorem 1.1 for a large class of ‘negatively curved’ groups, including all torsion free non-amenable hyperbolic groups. Their result was then generalized to all acylindrically hyperbolic groups in [Reference Chifan, Das and SunCDS22].
Most recently, a similar result as our Theorem 1.5 was proved in [Reference Amrutam and HartmanAH22]. Indeed, they prove a co-amenability result for invariant
![]() $C^*$
-algebras of reduced
$C^*$
-algebras of reduced
![]() $C^*$
-algebras of a class of groups satisfying a ‘singularity’ property (see [Reference Amrutam and HartmanAH22, Examples]).
$C^*$
-algebras of a class of groups satisfying a ‘singularity’ property (see [Reference Amrutam and HartmanAH22, Examples]).
2
 $\Gamma $
-invariant subalgebras
$\Gamma $
-invariant subalgebras
In this section, we prove some general properties of co-finite
![]() $\Gamma $
-invariant subalgebras, mostly in the case of
$\Gamma $
-invariant subalgebras, mostly in the case of
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
and
$C^{*}_{\mathrm {red}}(\Gamma )$
and
![]() $\mathcal {L}(\Gamma )$
. Throughout the section,
$\mathcal {L}(\Gamma )$
. Throughout the section,
![]() $\Gamma $
is always a discrete group.
$\Gamma $
is always a discrete group.
We observe that if
![]() ${\Lambda }$
is a finite-index normal subgroup of
${\Lambda }$
is a finite-index normal subgroup of
![]() $\Gamma $
, then for any unitary representation
$\Gamma $
, then for any unitary representation
![]() $\pi $
of
$\pi $
of
![]() $\Gamma $
,
$\Gamma $
,
![]() $C^*_\pi ({\Lambda })$
is co-finite in
$C^*_\pi ({\Lambda })$
is co-finite in
![]() $C^*_\pi (\Gamma )$
and
$C^*_\pi (\Gamma )$
and
![]() $\pi ({\Lambda })^{\prime \prime }$
is co-finite in
$\pi ({\Lambda })^{\prime \prime }$
is co-finite in
![]() $\pi (\Gamma )^{\prime \prime }$
. Indeed, in this case, the
$\pi (\Gamma )^{\prime \prime }$
. Indeed, in this case, the
![]() $\Gamma $
-action on
$\Gamma $
-action on
![]() $\textsf {M}:= {C^*_\pi ({\Lambda })}^{\prime } = \pi (\Lambda )^{\prime }$
factors through the finite group
$\textsf {M}:= {C^*_\pi ({\Lambda })}^{\prime } = \pi (\Lambda )^{\prime }$
factors through the finite group
![]() $\Gamma /\Lambda $
, and hence
$\Gamma /\Lambda $
, and hence
![]() $\textsf {M}$
admits a
$\textsf {M}$
admits a
![]() $\Gamma $
-invariant ultraweakly continuous state.
$\Gamma $
-invariant ultraweakly continuous state.
One expects that for any natural notion of co-finiteness, certain properties should lift from co-finite subalgebras to the ambient space. In the following theorem, we prove that amenability and Haagerup property are among such properties.
Theorem 2.1. Let
![]() $\pi $
be a factorial unitary representation of
$\pi $
be a factorial unitary representation of
![]() $\,\Gamma $
.
$\,\Gamma $
.
-
(1) If
 $\pi (\Gamma )^{\prime \prime }$
has a non-trivial co-finite
$\pi (\Gamma )^{\prime \prime }$
has a non-trivial co-finite
 $\Gamma $
-invariant von Neumann subalgebra with the Haagerup property, then
$\Gamma $
-invariant von Neumann subalgebra with the Haagerup property, then
 $\pi (\Gamma )^{\prime \prime }$
also has the Haagerup property.
$\pi (\Gamma )^{\prime \prime }$
also has the Haagerup property. -
(2) If
 $\pi (\Gamma )^{\prime \prime }$
has an amenable non-trivial co-finite
$\pi (\Gamma )^{\prime \prime }$
has an amenable non-trivial co-finite
 $\Gamma $
-invariant von Neumann subalgebra, then
$\Gamma $
-invariant von Neumann subalgebra, then
 $\pi (\Gamma )^{\prime \prime }$
is amenable.
$\pi (\Gamma )^{\prime \prime }$
is amenable.
Proof. (1) Let
![]() $\textsf {N}\subset \pi (\Gamma )^{\prime \prime }$
be a non-trivial co-finite
$\textsf {N}\subset \pi (\Gamma )^{\prime \prime }$
be a non-trivial co-finite
![]() $\Gamma $
-invariant von Neumann subalgebra that has the Haagerup property. Let
$\Gamma $
-invariant von Neumann subalgebra that has the Haagerup property. Let
![]() $\textsf {M}=\textsf {N}^\prime \subset B(H_\pi )$
. Then,
$\textsf {M}=\textsf {N}^\prime \subset B(H_\pi )$
. Then,
![]() $\textsf {M}$
has the Haagerup property [Reference Okayasu and TomatsuOT15, Theorem 3.12]. Let
$\textsf {M}$
has the Haagerup property [Reference Okayasu and TomatsuOT15, Theorem 3.12]. Let
![]() $\tau $
be the
$\tau $
be the
![]() $\Gamma $
-invariant ultraweakly continuous state on
$\Gamma $
-invariant ultraweakly continuous state on
![]() $\textsf {M}$
guaranteed by the co-finiteness assumption, and let
$\textsf {M}$
guaranteed by the co-finiteness assumption, and let
![]() $\mathfrak {p}\in \textsf {M}$
be the support projection of
$\mathfrak {p}\in \textsf {M}$
be the support projection of
![]() $\tau $
. Then,
$\tau $
. Then,
![]() $\mathfrak {p}$
is
$\mathfrak {p}$
is
![]() $\Gamma $
-invariant and therefore
$\Gamma $
-invariant and therefore
![]() $\mathfrak {p}\in \pi (\Gamma )^\prime $
. Furthermore,
$\mathfrak {p}\in \pi (\Gamma )^\prime $
. Furthermore,
![]() $\tau $
restricts to a faithful
$\tau $
restricts to a faithful
![]() $\Gamma $
-invariant ultraweakly continuous state on the
$\Gamma $
-invariant ultraweakly continuous state on the
![]() $\Gamma $
-von Neumann algebra
$\Gamma $
-von Neumann algebra
![]() $\mathfrak {p}\textsf {M}\mathfrak {p}$
, which also has the Haagerup property [Reference Okayasu and TomatsuOT15, Corollary 3.13]. Since there exists a conditional expectation
$\mathfrak {p}\textsf {M}\mathfrak {p}$
, which also has the Haagerup property [Reference Okayasu and TomatsuOT15, Corollary 3.13]. Since there exists a conditional expectation
![]() $\mathfrak {p}\textsf {M}\mathfrak {p}\to (\mathfrak {p}\textsf {M}\mathfrak {p})^\Gamma = \mathfrak {p}\textsf {M}^\Gamma \mathfrak {p} = \mathfrak {p}\pi (\Gamma )^\prime \mathfrak {p}$
(see, e.g., [Reference Bader, Boutonnet, Houdayer and PetersonBBHP20, Proposition 2.7(1)]), it follows that
$\mathfrak {p}\textsf {M}\mathfrak {p}\to (\mathfrak {p}\textsf {M}\mathfrak {p})^\Gamma = \mathfrak {p}\textsf {M}^\Gamma \mathfrak {p} = \mathfrak {p}\pi (\Gamma )^\prime \mathfrak {p}$
(see, e.g., [Reference Bader, Boutonnet, Houdayer and PetersonBBHP20, Proposition 2.7(1)]), it follows that
![]() $\mathfrak {p}\pi (\Gamma )^\prime \mathfrak {p}$
has the Haagerup property [Reference Okayasu and TomatsuOT15, Theorem 5.9]. Since
$\mathfrak {p}\pi (\Gamma )^\prime \mathfrak {p}$
has the Haagerup property [Reference Okayasu and TomatsuOT15, Theorem 5.9]. Since
![]() $\mathfrak {p}\pi (\Gamma )^\prime \mathfrak {p}$
is a factor, this implies
$\mathfrak {p}\pi (\Gamma )^\prime \mathfrak {p}$
is a factor, this implies
![]() $\pi (\Gamma )^\prime $
has the Haagerup property [Reference Okayasu and TomatsuOT15, Corollary 3.13]. Invoking [Reference Okayasu and TomatsuOT15, Theorem 3.12] once again, the result follows. (2) The proof is similar and the corresponding permanence results regarding amenability are well known (see, e.g., [Reference Anantharaman-Delaroche and PopaADP]).
$\pi (\Gamma )^\prime $
has the Haagerup property [Reference Okayasu and TomatsuOT15, Corollary 3.13]. Invoking [Reference Okayasu and TomatsuOT15, Theorem 3.12] once again, the result follows. (2) The proof is similar and the corresponding permanence results regarding amenability are well known (see, e.g., [Reference Anantharaman-Delaroche and PopaADP]).
Corollary 2.2. Let
![]() $\Gamma $
be icc. Then the following hold.
$\Gamma $
be icc. Then the following hold.
-
(1)
 $\Gamma $
is amenable if and only if
$\Gamma $
is amenable if and only if
 $\mathcal {L}(\Gamma )$
has a non-trivial co-finite
$\mathcal {L}(\Gamma )$
has a non-trivial co-finite
 $\Gamma $
-invariant amenable von Neumann subalgebra.
$\Gamma $
-invariant amenable von Neumann subalgebra. -
(2)
 $\Gamma $
has the Haagerup property if and only if
$\Gamma $
has the Haagerup property if and only if
 $\mathcal {L}(\Gamma )$
has a non-trivial co-finite
$\mathcal {L}(\Gamma )$
has a non-trivial co-finite
 $\Gamma $
-invariant von Neumann subalgebra that has the Haagerup property.
$\Gamma $
-invariant von Neumann subalgebra that has the Haagerup property.
Proof. The arguments for both parts are similar and therefore we only give the proof of item (2). If
![]() $\Gamma $
has the Haagerup property, then
$\Gamma $
has the Haagerup property, then
![]() $\mathcal {L}(\Gamma )$
has the Haagerup property and so the forward direction follows. Conversely, since
$\mathcal {L}(\Gamma )$
has the Haagerup property and so the forward direction follows. Conversely, since
![]() $\Gamma $
is icc, the regular representation of
$\Gamma $
is icc, the regular representation of
![]() $\Gamma $
is factorial. If
$\Gamma $
is factorial. If
![]() $\mathcal {L}(\Gamma )$
has a non-trivial co-finite
$\mathcal {L}(\Gamma )$
has a non-trivial co-finite
![]() $\Gamma $
-invariant von Neumann subalgebra that has the Haagerup property, Theorem 2.1 implies that
$\Gamma $
-invariant von Neumann subalgebra that has the Haagerup property, Theorem 2.1 implies that
![]() $\mathcal {L}(\Gamma )$
has the Haagerup property. Hence,
$\mathcal {L}(\Gamma )$
has the Haagerup property. Hence,
![]() $\Gamma $
has the Haagerup property [Reference ChodaCho83, Theorem 3].
$\Gamma $
has the Haagerup property [Reference ChodaCho83, Theorem 3].
2.1 Non-commutative just-infiniteness
Any notion of co-finiteness in the operator algebraic setting naturally leads to non-commutative notions of just-infiniteness.
Definition 2.3. We say
![]() $\Gamma $
is
$\Gamma $
is
![]() $\pi $
-just-infinite if
$\pi $
-just-infinite if
![]() $\pi $
is infinite-dimensional and every non-trivial
$\pi $
is infinite-dimensional and every non-trivial
![]() $\Gamma $
-invariant von Neumann subalgebra
$\Gamma $
-invariant von Neumann subalgebra
![]() $\textsf {N}\subset \pi (\Gamma )^{\prime \prime }$
is co-finite in
$\textsf {N}\subset \pi (\Gamma )^{\prime \prime }$
is co-finite in
![]() $\pi (\Gamma )^{\prime \prime }$
.
$\pi (\Gamma )^{\prime \prime }$
.
Recall that
![]() $\unicode{x3bb} _\Gamma $
denotes the left regular representation of
$\unicode{x3bb} _\Gamma $
denotes the left regular representation of
![]() $\Gamma $
.
$\Gamma $
.
Lemma 2.4. If
![]() $\Gamma $
is
$\Gamma $
is
![]() $\unicode{x3bb} _\Gamma $
-just-infinite, then
$\unicode{x3bb} _\Gamma $
-just-infinite, then
![]() $\Gamma $
is icc.
$\Gamma $
is icc.
Proof. Assume
![]() $\Gamma $
is not icc. Then
$\Gamma $
is not icc. Then
![]() $\mathcal {L}(\Gamma )$
contains a non-trivial central projection
$\mathcal {L}(\Gamma )$
contains a non-trivial central projection
![]() $\mathfrak {p}$
. Then,
$\mathfrak {p}$
. Then,
![]() $\textsf {N}= {\mathbb {C}} \mathfrak {p} \oplus {\mathbb {C}} \mathfrak {p}^\perp $
is a non-trivial
$\textsf {N}= {\mathbb {C}} \mathfrak {p} \oplus {\mathbb {C}} \mathfrak {p}^\perp $
is a non-trivial
![]() $\Gamma $
-invariant von Neumann subalgebra of
$\Gamma $
-invariant von Neumann subalgebra of
![]() $\mathcal {L}(\Gamma )$
, and
$\mathcal {L}(\Gamma )$
, and
![]() $\textsf {N}^\prime = \mathfrak {p} B(\ell ^2(\Gamma ))\mathfrak {p}\,\oplus \, \mathfrak {p}^\perp B(\ell ^2(\Gamma ))\mathfrak {p}^\perp $
. Since
$\textsf {N}^\prime = \mathfrak {p} B(\ell ^2(\Gamma ))\mathfrak {p}\,\oplus \, \mathfrak {p}^\perp B(\ell ^2(\Gamma ))\mathfrak {p}^\perp $
. Since
![]() $\Gamma $
is
$\Gamma $
is
![]() $\unicode{x3bb} _\Gamma $
-just-infinite,
$\unicode{x3bb} _\Gamma $
-just-infinite,
![]() $\textsf {N}^\prime $
admits a
$\textsf {N}^\prime $
admits a
![]() $\Gamma $
-invariant ultraweakly continuous state. The map
$\Gamma $
-invariant ultraweakly continuous state. The map
![]() $\mathfrak {a} \mapsto \mathfrak {p}\mathfrak {a} \mathfrak {p} + \mathfrak {p}^\perp \mathfrak {a} \mathfrak {p}^\perp $
, from
$\mathfrak {a} \mapsto \mathfrak {p}\mathfrak {a} \mathfrak {p} + \mathfrak {p}^\perp \mathfrak {a} \mathfrak {p}^\perp $
, from
![]() $B(\ell ^2(\Gamma ))$
to
$B(\ell ^2(\Gamma ))$
to
![]() $\textsf {N}^\prime $
, is ultraweakly continuous,
$\textsf {N}^\prime $
, is ultraweakly continuous,
![]() $\Gamma $
-equivariant, and ucp. Hence, we obtain a
$\Gamma $
-equivariant, and ucp. Hence, we obtain a
![]() $\Gamma $
-invariant ultraweakly continuous state on
$\Gamma $
-invariant ultraweakly continuous state on
![]() $B(\ell ^2(\Gamma ))$
, which implies that
$B(\ell ^2(\Gamma ))$
, which implies that
![]() $\Gamma $
is finite. This is a contradiction as
$\Gamma $
is finite. This is a contradiction as
![]() $\unicode{x3bb} _\Gamma $
-just-infinite groups are, by definition, assumed to be infinite.
$\unicode{x3bb} _\Gamma $
-just-infinite groups are, by definition, assumed to be infinite.
Example 2.5. It follows from Lemma 2.4 that there are just-infinite groups that are not
![]() $\unicode{x3bb} _\Gamma $
-just-infinite. Indeed, the integer group
$\unicode{x3bb} _\Gamma $
-just-infinite. Indeed, the integer group
![]() $\Gamma ={\mathbb {Z}}$
is just-infinite (in fact, it even satisfies the conclusion of Stuck–Zimmer’s rigidity theorem), but not icc, and hence it is not
$\Gamma ={\mathbb {Z}}$
is just-infinite (in fact, it even satisfies the conclusion of Stuck–Zimmer’s rigidity theorem), but not icc, and hence it is not
![]() $\unicode{x3bb} _\Gamma $
-just-infinite by Lemma 2.4.
$\unicode{x3bb} _\Gamma $
-just-infinite by Lemma 2.4.
Proposition 2.6. Assume
![]() $\Gamma $
is
$\Gamma $
is
![]() $\unicode{x3bb} _\Gamma $
-just-infinite and does not have the Haagerup property. Then
$\unicode{x3bb} _\Gamma $
-just-infinite and does not have the Haagerup property. Then
![]() $\mathcal {L}(\Gamma )$
does not admit any non-trivial
$\mathcal {L}(\Gamma )$
does not admit any non-trivial
![]() $\Gamma $
-invariant von Neumann subalgebras with the Haagerup property.
$\Gamma $
-invariant von Neumann subalgebras with the Haagerup property.
Proof. For the sake of contradiction, assume that
![]() $\textsf {N}$
is a non-trivial
$\textsf {N}$
is a non-trivial
![]() $\Gamma $
-invariant von Neumann subalgebra of
$\Gamma $
-invariant von Neumann subalgebra of
![]() $\mathcal {L}(\Gamma )$
with the Haagerup property. Since,
$\mathcal {L}(\Gamma )$
with the Haagerup property. Since,
![]() $\Gamma $
is
$\Gamma $
is
![]() $\unicode{x3bb} _\Gamma $
-just-infinite,
$\unicode{x3bb} _\Gamma $
-just-infinite,
![]() $\textsf {N}$
is co-finite in
$\textsf {N}$
is co-finite in
![]() $\mathcal {L}(\Gamma )$
, and hence Lemma 2.4 and Corollary 2.2 entail that
$\mathcal {L}(\Gamma )$
, and hence Lemma 2.4 and Corollary 2.2 entail that
![]() $\mathcal {L}(\Gamma )$
has the Haagerup property. Therefore, by [Reference ChodaCho83, Theorem 3], the group
$\mathcal {L}(\Gamma )$
has the Haagerup property. Therefore, by [Reference ChodaCho83, Theorem 3], the group
![]() $\Gamma $
has the Haagerup property, which contradicts our assumption.
$\Gamma $
has the Haagerup property, which contradicts our assumption.
Proposition 2.7. Assume that
![]() $\Gamma $
is
$\Gamma $
is
![]() $\unicode{x3bb} _\Gamma $
-just-infinite and non-amenable. Then
$\unicode{x3bb} _\Gamma $
-just-infinite and non-amenable. Then
![]() $\mathcal {L}(\Gamma )$
does not admit any non-trivial
$\mathcal {L}(\Gamma )$
does not admit any non-trivial
![]() $\Gamma $
-invariant amenable von Neumann subalgebras.
$\Gamma $
-invariant amenable von Neumann subalgebras.
Proof. The argument is similar to the proof of Proposition 2.6, using the fact that
![]() $\mathcal {L}(\Gamma )$
is amenable if and only if
$\mathcal {L}(\Gamma )$
is amenable if and only if
![]() $\Gamma $
is amenable.
$\Gamma $
is amenable.
In particular, we conclude the following corollary.
Corollary 2.8. Assume that
![]() $\Gamma $
is
$\Gamma $
is
![]() $\unicode{x3bb} _\Gamma $
-just-infinite and non-amenable. Then
$\unicode{x3bb} _\Gamma $
-just-infinite and non-amenable. Then
![]() $\mathcal {L}(\Gamma )$
does not admit any Cartan subalgebra with
$\mathcal {L}(\Gamma )$
does not admit any Cartan subalgebra with
![]() $\unicode{x3bb} _\Gamma (\Gamma )\subseteq \mathcal {N}_{\mathcal {L}(\Gamma )}(\textsf {N})$
.
$\unicode{x3bb} _\Gamma (\Gamma )\subseteq \mathcal {N}_{\mathcal {L}(\Gamma )}(\textsf {N})$
.
Let us point out that the current understanding of Cartan subalgebras of
![]() $\mathcal {L}(\Gamma )$
is far from complete in the higher-rank realm. Important partial results in this direction were obtained recently by Boutonnet, Ioana and Peterson in [Reference Boutonnet, Ioana and PetersonBIP21], where they proved the lack of certain classes of Cartan subalgebras.
$\mathcal {L}(\Gamma )$
is far from complete in the higher-rank realm. Important partial results in this direction were obtained recently by Boutonnet, Ioana and Peterson in [Reference Boutonnet, Ioana and PetersonBIP21], where they proved the lack of certain classes of Cartan subalgebras.
We conclude the section with another useful consequence of Proposition 2.7.
Corollary 2.9. Assume
![]() $\Gamma $
is
$\Gamma $
is
![]() $\unicode{x3bb} _\Gamma $
-just-infinite and non-amenable. Then every
$\unicode{x3bb} _\Gamma $
-just-infinite and non-amenable. Then every
![]() $\Gamma $
-invariant von Neumann subalgebra of
$\Gamma $
-invariant von Neumann subalgebra of
![]() $\mathcal {L}(\Gamma )$
is a factor.
$\mathcal {L}(\Gamma )$
is a factor.
Proof. Let
![]() $\textsf {M}$
be a
$\textsf {M}$
be a
![]() $\Gamma $
-invariant von Neumann subalgebra of
$\Gamma $
-invariant von Neumann subalgebra of
![]() $\mathcal {L}(\Gamma )$
. Then the center
$\mathcal {L}(\Gamma )$
. Then the center
![]() $\mathcal {Z}(\textsf {M})$
of
$\mathcal {Z}(\textsf {M})$
of
![]() $\textsf {M}$
is a
$\textsf {M}$
is a
![]() $\Gamma $
-invariant abelian von Neumann subalgebra of
$\Gamma $
-invariant abelian von Neumann subalgebra of
![]() $\mathcal {L}(\Gamma )$
. Hence,
$\mathcal {L}(\Gamma )$
. Hence,
![]() $\mathcal {Z}(\textsf {M})={\mathbb {C}}1$
by Proposition 2.7.
$\mathcal {Z}(\textsf {M})={\mathbb {C}}1$
by Proposition 2.7.
2.2 Weak just-infiniteness
We have parallel results in the
![]() $C^*$
-algebra setup and, as we see, a formally weaker just-infiniteness condition would suffice.
$C^*$
-algebra setup and, as we see, a formally weaker just-infiniteness condition would suffice.
Definition 2.10. Let
![]() $\pi $
be a unitary representation of
$\pi $
be a unitary representation of
![]() $\Gamma $
. We say
$\Gamma $
. We say
![]() $\Gamma $
is weakly
$\Gamma $
is weakly
![]() $\pi $
-just-infinite if
$\pi $
-just-infinite if
![]() $\pi $
is infinite-dimensional and every non-trivial
$\pi $
is infinite-dimensional and every non-trivial
![]() $\Gamma $
-invariant unital
$\Gamma $
-invariant unital
![]() $C^*$
-subalgebra of
$C^*$
-subalgebra of
![]() $C^*_\pi (\Gamma )$
is co-finite in
$C^*_\pi (\Gamma )$
is co-finite in
![]() $C^*_\pi (\Gamma )$
.
$C^*_\pi (\Gamma )$
.
The next two results are
![]() $C^*$
-algebraic analogs of Propositions 2.6 and 2.7. By the canonical trace, we mean the (unique) state on
$C^*$
-algebraic analogs of Propositions 2.6 and 2.7. By the canonical trace, we mean the (unique) state on
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
that extends the Dirac function supported on the neutral element
$C^{*}_{\mathrm {red}}(\Gamma )$
that extends the Dirac function supported on the neutral element
![]() $e\in \Gamma $
.
$e\in \Gamma $
.
Proposition 2.11. Assume
![]() $\Gamma $
is weakly
$\Gamma $
is weakly
![]() $\unicode{x3bb} _\Gamma $
-just-infinite and does not have the Haagerup property. Then,
$\unicode{x3bb} _\Gamma $
-just-infinite and does not have the Haagerup property. Then,
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
does not admit any non-trivial
$C^{*}_{\mathrm {red}}(\Gamma )$
does not admit any non-trivial
![]() $\Gamma $
-invariant exact
$\Gamma $
-invariant exact
![]() $C^*$
-subalgebra on which the restriction of the canonical trace is an amenable trace.
$C^*$
-subalgebra on which the restriction of the canonical trace is an amenable trace.
Proof. For the sake of contradiction, assume that
![]() $\textsf {A}$
is a non-trivial exact
$\textsf {A}$
is a non-trivial exact
![]() $\Gamma $
-invariant unital
$\Gamma $
-invariant unital
![]() $C^*$
-subalgebra of
$C^*$
-subalgebra of
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
such that the restriction of the canonical trace
$C^{*}_{\mathrm {red}}(\Gamma )$
such that the restriction of the canonical trace
![]() $\tau $
to
$\tau $
to
![]() $\textsf {A}$
is an amenable trace on
$\textsf {A}$
is an amenable trace on
![]() $\textsf {A}$
. Then by [Reference SuzukiSuz13, Corollary 3.7], the pair
$\textsf {A}$
. Then by [Reference SuzukiSuz13, Corollary 3.7], the pair
![]() $(\textsf {A}, \tau )$
has the Haagerup property in the sense of [Reference DongDon11] and so from [Reference SuzukiSuz13, Lemma 4.5], it follows that
$(\textsf {A}, \tau )$
has the Haagerup property in the sense of [Reference DongDon11] and so from [Reference SuzukiSuz13, Lemma 4.5], it follows that
![]() $\textsf {A}^{\prime \prime }$
has the Haagerup property. Since
$\textsf {A}^{\prime \prime }$
has the Haagerup property. Since
![]() $\textsf {A}$
is co-finite in
$\textsf {A}$
is co-finite in
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
by
$C^{*}_{\mathrm {red}}(\Gamma )$
by
![]() $\unicode{x3bb} _\Gamma $
-just-infiniteness,
$\unicode{x3bb} _\Gamma $
-just-infiniteness,
![]() $\textsf {A}^{\prime \prime }$
is co-finite in
$\textsf {A}^{\prime \prime }$
is co-finite in
![]() $\mathcal {L}(\Gamma )$
and so, as in the proof of Proposition 2.6, we get a contradiction.
$\mathcal {L}(\Gamma )$
and so, as in the proof of Proposition 2.6, we get a contradiction.
Proposition 2.12. Assume that
![]() $\Gamma $
is weakly
$\Gamma $
is weakly
![]() $\unicode{x3bb} _\Gamma $
-just-infinite and non-amenable. Then,
$\unicode{x3bb} _\Gamma $
-just-infinite and non-amenable. Then,
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
does not admit any non-trivial
$C^{*}_{\mathrm {red}}(\Gamma )$
does not admit any non-trivial
![]() $\Gamma $
-invariant nuclear
$\Gamma $
-invariant nuclear
![]() $C^*$
-subalgebras.
$C^*$
-subalgebras.
Proof. For the sake of contradiction, assume that
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
admits a non-trivial nuclear
$C^{*}_{\mathrm {red}}(\Gamma )$
admits a non-trivial nuclear
![]() $\Gamma $
-invariant unital
$\Gamma $
-invariant unital
![]() $C^*$
-subalgebra
$C^*$
-subalgebra
![]() $\textsf {A}$
. Then,
$\textsf {A}$
. Then,
![]() $\textsf {A}$
is co-finite in
$\textsf {A}$
is co-finite in
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
by
$C^{*}_{\mathrm {red}}(\Gamma )$
by
![]() $C^*$
-just-infiniteness and so
$C^*$
-just-infiniteness and so
![]() $\textsf {A}^{\prime \prime }$
is a non-trivial amenable co-finite von Neumann subalgebra of
$\textsf {A}^{\prime \prime }$
is a non-trivial amenable co-finite von Neumann subalgebra of
![]() $\mathcal {L}(\Gamma )$
. Corollary 2.2 implies that
$\mathcal {L}(\Gamma )$
. Corollary 2.2 implies that
![]() $\mathcal {L}(\Gamma )$
is amenable, and hence
$\mathcal {L}(\Gamma )$
is amenable, and hence
![]() $\Gamma $
is amenable. This is a contradiction and the proof is complete.
$\Gamma $
is amenable. This is a contradiction and the proof is complete.
2.3
 $C^*$
-just-infinite groups
$C^*$
-just-infinite groups
In this section, we prove Theorem 1.5.
Definition 2.13. A discrete group
![]() $\Gamma $
is said to be
$\Gamma $
is said to be
![]() $C^*$
-just-infinite, if it is infinite and for every non-trivial invariant unital
$C^*$
-just-infinite, if it is infinite and for every non-trivial invariant unital
![]() $C^*$
-subalgebra
$C^*$
-subalgebra
![]() $\textsf {A}$
of
$\textsf {A}$
of
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
and every unitary representation
$C^{*}_{\mathrm {red}}(\Gamma )$
and every unitary representation
![]() $\pi $
of
$\pi $
of
![]() $\Gamma $
which is weakly contained in
$\Gamma $
which is weakly contained in
![]() $\unicode{x3bb} _\Gamma $
,
$\unicode{x3bb} _\Gamma $
,
![]() $\pi (\textsf {A})$
is co-finite in
$\pi (\textsf {A})$
is co-finite in
![]() $C^*_\pi (\Gamma )$
.
$C^*_\pi (\Gamma )$
.
We remark that for a given unitary representation
![]() $\pi $
of
$\pi $
of
![]() $\Gamma $
and an invariant
$\Gamma $
and an invariant
![]() $C^{*}$
-subalgebra
$C^{*}$
-subalgebra
![]() $\textsf {A}\subset C^*_\pi (\Gamma )$
, co-finiteness of
$\textsf {A}\subset C^*_\pi (\Gamma )$
, co-finiteness of
![]() $\textsf {A}$
is effectively a von Neumann algebraic property: it is equivalent to the same property for the bi-commutant
$\textsf {A}$
is effectively a von Neumann algebraic property: it is equivalent to the same property for the bi-commutant
![]() ${\textsf {A}}^{\prime \prime }$
. Noting that the unitary representations
${\textsf {A}}^{\prime \prime }$
. Noting that the unitary representations
![]() $\pi $
of
$\pi $
of
![]() $\Gamma $
which are weakly contained in
$\Gamma $
which are weakly contained in
![]() $\unicode{x3bb} _\Gamma $
correspond to
$\unicode{x3bb} _\Gamma $
correspond to
![]() $\ast $
-representations of
$\ast $
-representations of
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
, we see that
$C^{*}_{\mathrm {red}}(\Gamma )$
, we see that
![]() $C^*$
-just-infiniteness is defined in terms of von Neumann algebras generated by all
$C^*$
-just-infiniteness is defined in terms of von Neumann algebras generated by all
![]() $\ast $
-representations of
$\ast $
-representations of
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
.
$C^{*}_{\mathrm {red}}(\Gamma )$
.
Before getting to the proof of Theorem 1.5, let us prove some general properties of
![]() $C^*$
-just-infinite groups.
$C^*$
-just-infinite groups.
Proposition 2.14. Let
![]() $\Gamma $
be a
$\Gamma $
be a
![]() $C^*$
-just-infinite group. Then,
$C^*$
-just-infinite group. Then,
![]() $\Gamma $
is either amenable or
$\Gamma $
is either amenable or
![]() $C^{*}$
-simple.
$C^{*}$
-simple.
Proof. Assume that
![]() $\Gamma $
is not
$\Gamma $
is not
![]() $C^*$
-simple. Let
$C^*$
-simple. Let
![]() $\pi \colon C^{*}_{\mathrm {red}}(\Gamma )\to B(H_\pi )$
be a non-faithful representation, and let
$\pi \colon C^{*}_{\mathrm {red}}(\Gamma )\to B(H_\pi )$
be a non-faithful representation, and let
![]() ${I}=\mathrm {ker}(\pi )$
. In particular,
${I}=\mathrm {ker}(\pi )$
. In particular,
![]() $\pi $
defines a unitary representation of
$\pi $
defines a unitary representation of
![]() $\Gamma $
which is weakly contained in
$\Gamma $
which is weakly contained in
![]() $\unicode{x3bb} $
. Define
$\unicode{x3bb} $
. Define
![]() $\textsf {A} = {I} + {\mathbb {C}} 1$
. Then,
$\textsf {A} = {I} + {\mathbb {C}} 1$
. Then,
![]() $\textsf {A}$
is a
$\textsf {A}$
is a
![]() $\Gamma $
-invariant unital
$\Gamma $
-invariant unital
![]() $C^*$
-subalgebra of
$C^*$
-subalgebra of
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
and
$C^{*}_{\mathrm {red}}(\Gamma )$
and
![]() $\pi (\textsf {A})= {\mathbb {C}} 1$
. Thus, by the assumption, there exists a
$\pi (\textsf {A})= {\mathbb {C}} 1$
. Thus, by the assumption, there exists a
![]() $\Gamma $
-invariant state on
$\Gamma $
-invariant state on
![]() $B(H_\pi )$
. Since
$B(H_\pi )$
. Since
![]() $\pi $
is weakly contained in
$\pi $
is weakly contained in
![]() $\unicode{x3bb} $
, there is also a
$\unicode{x3bb} $
, there is also a
![]() $\Gamma $
-invariant state on
$\Gamma $
-invariant state on
![]() $B(\ell ^2(\Gamma ))$
[Reference BekkaBek90, Corollary 5.3]. Restricting this state to
$B(\ell ^2(\Gamma ))$
[Reference BekkaBek90, Corollary 5.3]. Restricting this state to
![]() $\ell ^\infty (\Gamma )$
yields an invariant mean on
$\ell ^\infty (\Gamma )$
yields an invariant mean on
![]() $\ell ^\infty (\Gamma )$
, and so
$\ell ^\infty (\Gamma )$
, and so
![]() $\Gamma $
is amenable.
$\Gamma $
is amenable.
Example 2.15. It follows from Proposition 2.14 that there are simple non-amenable groups
![]() $\Gamma $
that are not
$\Gamma $
that are not
![]() $C^*$
-just-infinite. Indeed, the existence of non-
$C^*$
-just-infinite. Indeed, the existence of non-
![]() $C^*$
-simple groups with the unique trace property was proven by Le Boudec in [Reference Le BoudecLB17]. And as remarked after [Reference Le BoudecLB17, Theorem C], those examples include groups admitting simple subgroups of index two. Obviously, any such subgroup is also non-
$C^*$
-simple groups with the unique trace property was proven by Le Boudec in [Reference Le BoudecLB17]. And as remarked after [Reference Le BoudecLB17, Theorem C], those examples include groups admitting simple subgroups of index two. Obviously, any such subgroup is also non-
![]() $C^*$
-simple and non-amenable, and therefore also non-
$C^*$
-simple and non-amenable, and therefore also non-
![]() $C^*$
-just-infinite by Proposition 2.14.
$C^*$
-just-infinite by Proposition 2.14.
2.4 Proof of Theorem 1.5
The proof of Theorem 1.5 is structured similarly to Margulis’ proof of the normal subgroup theorem, consisting of two parts: the amenability half and the property (T) half.
Proof of Theorem 1.5
Let
![]() $\textsf {A}\subset C^{*}_{\mathrm {red}}(\Gamma )$
be a non-trivial
$\textsf {A}\subset C^{*}_{\mathrm {red}}(\Gamma )$
be a non-trivial
![]() $\Gamma $
-invariant unital
$\Gamma $
-invariant unital
![]() $C^*$
-subalgebra, and let
$C^*$
-subalgebra, and let
![]() $\pi $
be a unitary representation of
$\pi $
be a unitary representation of
![]() $\Gamma $
which is weakly contained in
$\Gamma $
which is weakly contained in
![]() $\unicode{x3bb} $
. Amenability half. We prove that
$\unicode{x3bb} $
. Amenability half. We prove that
![]() ${\pi (\textsf {A})}^\prime $
admits a
${\pi (\textsf {A})}^\prime $
admits a
![]() $\Gamma $
-invariant state.
$\Gamma $
-invariant state.
Let P be a minimal parabolic subgroup, and K a maximal compact subgroup of G such that
![]() $G=KP$
. Let
$G=KP$
. Let
![]() $\nu _P$
be the unique K-invariant probability on
$\nu _P$
be the unique K-invariant probability on
![]() $G/P$
, and
$G/P$
, and
![]() $\mu $
a fully supported probability on
$\mu $
a fully supported probability on
![]() $\Gamma $
such that
$\Gamma $
such that
![]() $(G/P, \nu _P)$
is the Poisson boundary of the
$(G/P, \nu _P)$
is the Poisson boundary of the
![]() $(\Gamma , \mu )$
-random walk [Reference FurstenbergFur67].
$(\Gamma , \mu )$
-random walk [Reference FurstenbergFur67].
We first show that it suffices to prove the claim for
![]() $\pi =\unicode{x3bb} $
. Note that the action
$\pi =\unicode{x3bb} $
. Note that the action
![]() $G\!\curvearrowright \! G/P$
is faithful; indeed, since
$G\!\curvearrowright \! G/P$
is faithful; indeed, since
![]() $\mathrm {ker}(G\!\curvearrowright \! G/P)$
is contained in P, it is a normal amenable subgroup of G, which is also a subproduct of G by semisimplicity. Since each factor of G is non-amenable, it follows that
$\mathrm {ker}(G\!\curvearrowright \! G/P)$
is contained in P, it is a normal amenable subgroup of G, which is also a subproduct of G by semisimplicity. Since each factor of G is non-amenable, it follows that
![]() $\mathrm {ker}(G\!\curvearrowright \! G/P)$
is trivial. In particular,
$\mathrm {ker}(G\!\curvearrowright \! G/P)$
is trivial. In particular,
![]() $\Gamma $
also acts faithfully on
$\Gamma $
also acts faithfully on
![]() $G/P$
. Thus, since the action
$G/P$
. Thus, since the action
![]() $\Gamma \!\curvearrowright \! G/P$
is strongly proximal [Reference FurstenbergFur67], it follows that
$\Gamma \!\curvearrowright \! G/P$
is strongly proximal [Reference FurstenbergFur67], it follows that
![]() $\Gamma $
has trivial amenable radical [Reference FurmanFur03, Proposition 7]. This implies
$\Gamma $
has trivial amenable radical [Reference FurmanFur03, Proposition 7]. This implies
![]() $\Gamma $
is
$\Gamma $
is
![]() $C^*$
-simple by [Reference Bekka, Cowling and de la HarpeBCdlH94, Theorem 1] (cf. [Reference Breuillard, Kalantar, Kennedy and OzawaBKKO17, Corollary 6.10]). Hence,
$C^*$
-simple by [Reference Bekka, Cowling and de la HarpeBCdlH94, Theorem 1] (cf. [Reference Breuillard, Kalantar, Kennedy and OzawaBKKO17, Corollary 6.10]). Hence,
![]() $\pi $
is weakly equivalent to
$\pi $
is weakly equivalent to
![]() $\unicode{x3bb} $
, which implies that the map
$\unicode{x3bb} $
, which implies that the map
![]() $\pi (g)\mapsto \unicode{x3bb} (g)$
extends to a
$\pi (g)\mapsto \unicode{x3bb} (g)$
extends to a
![]() $*$
-homomorphism
$*$
-homomorphism
![]() $\psi : C^*_\pi (\Gamma )\to C^{*}_{\mathrm {red}}(\Gamma )$
. By Arveson’s extension theorem [Reference ArvesonArv69, Theorem 1.2.3], we may extend
$\psi : C^*_\pi (\Gamma )\to C^{*}_{\mathrm {red}}(\Gamma )$
. By Arveson’s extension theorem [Reference ArvesonArv69, Theorem 1.2.3], we may extend
![]() $\psi $
to a ucp map from
$\psi $
to a ucp map from
![]() $B(H_\pi )$
to
$B(H_\pi )$
to
![]() $B(\ell ^2(\Gamma ))$
which we will still denote by
$B(\ell ^2(\Gamma ))$
which we will still denote by
![]() $\psi $
. Since
$\psi $
. Since
![]() $C^*_\pi (\Gamma )$
lies in the multiplicative domain of
$C^*_\pi (\Gamma )$
lies in the multiplicative domain of
![]() $\psi $
, it follows that
$\psi $
, it follows that
![]() $\psi $
is
$\psi $
is
![]() $\Gamma $
-equivariant and hence
$\Gamma $
-equivariant and hence
![]() $\psi ({\pi (\textsf {A})}^\prime )\subset {\textsf {A}}^\prime $
. Therefore, it suffices to show that
$\psi ({\pi (\textsf {A})}^\prime )\subset {\textsf {A}}^\prime $
. Therefore, it suffices to show that
![]() ${\textsf {A}}^\prime $
admits a
${\textsf {A}}^\prime $
admits a
![]() $\Gamma $
-invariant state.
$\Gamma $
-invariant state.
Define
![]() $\theta _\mu =\sum _{g\in \Gamma }\mu (g) \mathrm {Ad}_{\rho (g)}$
, where
$\theta _\mu =\sum _{g\in \Gamma }\mu (g) \mathrm {Ad}_{\rho (g)}$
, where
![]() $\rho \colon \Gamma \to B(\ell ^2(\Gamma ))$
is the right regular representation. Then,
$\rho \colon \Gamma \to B(\ell ^2(\Gamma ))$
is the right regular representation. Then,
![]() $\theta _\mu $
is a
$\theta _\mu $
is a
![]() $\Gamma $
-equivariant ucp map on
$\Gamma $
-equivariant ucp map on
![]() $B(\ell ^2(\Gamma ))$
, and we denote by
$B(\ell ^2(\Gamma ))$
, and we denote by
![]() ${\widetilde {\mathcal {H}}}_\mu $
the non-commutative Poisson boundary of
${\widetilde {\mathcal {H}}}_\mu $
the non-commutative Poisson boundary of
![]() $(\Gamma ,\mu )$
in the sense of Izumi [Reference IzumiIzu04], that is, the space
$(\Gamma ,\mu )$
in the sense of Izumi [Reference IzumiIzu04], that is, the space
![]() $\{\mathfrak {a}\in B(\ell ^2(\Gamma )) : \theta _\mu (\mathfrak {a}) = \mathfrak {a}\}$
of fixed points of
$\{\mathfrak {a}\in B(\ell ^2(\Gamma )) : \theta _\mu (\mathfrak {a}) = \mathfrak {a}\}$
of fixed points of
![]() $\theta _\mu $
. We also denote by
$\theta _\mu $
. We also denote by
![]() $\mathcal {H}_\mu = {\widetilde {\mathcal {H}}}_\mu \cap \ell ^\infty (\Gamma )$
the space of bounded
$\mathcal {H}_\mu = {\widetilde {\mathcal {H}}}_\mu \cap \ell ^\infty (\Gamma )$
the space of bounded
![]() $\mu $
-harmonic functions on
$\mu $
-harmonic functions on
![]() $\Gamma $
.
$\Gamma $
.
Any point-weak* limit
![]() $\mathcal {E}_\mu $
of the sequence of maps
$\mathcal {E}_\mu $
of the sequence of maps
![]() $(1/n) \sum _{k=1}^{n} \theta _{\mu ^{k}}$
defines a
$(1/n) \sum _{k=1}^{n} \theta _{\mu ^{k}}$
defines a
![]() $\Gamma $
-equivariant ucp idempotent from
$\Gamma $
-equivariant ucp idempotent from
![]() $B(\ell ^2(\Gamma ))$
onto
$B(\ell ^2(\Gamma ))$
onto
![]() ${\widetilde {\mathcal {H}}}_\mu $
, and endowed with the Choi–Effros product defined by
${\widetilde {\mathcal {H}}}_\mu $
, and endowed with the Choi–Effros product defined by
![]() $\mathcal {E}_\mu $
, the space
$\mathcal {E}_\mu $
, the space
![]() ${\widetilde {\mathcal {H}}}_\mu $
becomes a von Neumann algebra. Note that
${\widetilde {\mathcal {H}}}_\mu $
becomes a von Neumann algebra. Note that
![]() ${\widetilde {\mathcal {H}}}_\mu $
is also canonically equipped with a
${\widetilde {\mathcal {H}}}_\mu $
is also canonically equipped with a
![]() $\Gamma $
-action since it is a
$\Gamma $
-action since it is a
![]() $\Gamma $
-invariant subspace of
$\Gamma $
-invariant subspace of
![]() $B(\ell ^2(\Gamma ))$
.
$B(\ell ^2(\Gamma ))$
.
Since
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
lies in the multiplicative domain of
$C^{*}_{\mathrm {red}}(\Gamma )$
lies in the multiplicative domain of
![]() $\mathcal {E}_\mu $
, it follows that
$\mathcal {E}_\mu $
, it follows that
![]() $\mathcal {E}_\mu ({\textsf {A}}^\prime )\subset {\textsf {A}}^\prime \cap {\widetilde {\mathcal {H}}}_\mu $
. We will show that the latter intersection admits a
$\mathcal {E}_\mu ({\textsf {A}}^\prime )\subset {\textsf {A}}^\prime \cap {\widetilde {\mathcal {H}}}_\mu $
. We will show that the latter intersection admits a
![]() $\Gamma $
-invariant state, hence its composition with
$\Gamma $
-invariant state, hence its composition with
![]() $\mathcal {E}_\mu $
yields a
$\mathcal {E}_\mu $
yields a
![]() $\Gamma $
-invariant state on
$\Gamma $
-invariant state on
![]() ${\textsf {A}}^\prime $
, and this will conclude the proof of this part.
${\textsf {A}}^\prime $
, and this will conclude the proof of this part.
By [Reference IzumiIzu04, Theorem 4.1], there is a
![]() $\Gamma $
-equivariant von Neumann algebra isomorphism
$\Gamma $
-equivariant von Neumann algebra isomorphism
![]() $\Phi \colon \Gamma \ltimes L^\infty (G/P)\to {\widetilde {\mathcal {H}}}_\mu $
that restricts to the identity map on
$\Phi \colon \Gamma \ltimes L^\infty (G/P)\to {\widetilde {\mathcal {H}}}_\mu $
that restricts to the identity map on
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
, and to the Poisson transform
$C^{*}_{\mathrm {red}}(\Gamma )$
, and to the Poisson transform
![]() $\mathcal {P}_{\nu _P}\colon L^\infty (G/P) \to \mathcal {H}_\mu $
defined by
$\mathcal {P}_{\nu _P}\colon L^\infty (G/P) \to \mathcal {H}_\mu $
defined by
![]() $\mathcal {P}_{\nu _P}(f)(g) = \int _{G/P}f(gx) \,d\nu _P(x)$
for
$\mathcal {P}_{\nu _P}(f)(g) = \int _{G/P}f(gx) \,d\nu _P(x)$
for
![]() ${f\in L^\infty (G/P)}$
and
${f\in L^\infty (G/P)}$
and
![]() $g\in \Gamma $
.
$g\in \Gamma $
.
Since
![]() $\Phi $
is identity on
$\Phi $
is identity on
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
, it maps the relative commutant
$C^{*}_{\mathrm {red}}(\Gamma )$
, it maps the relative commutant
![]() $\textsf {B}$
of
$\textsf {B}$
of
![]() $\textsf {A}$
in the crossed product
$\textsf {A}$
in the crossed product
![]() $\Gamma \ltimes L^\infty (G/P)$
onto
$\Gamma \ltimes L^\infty (G/P)$
onto
![]() ${\textsf {A}}^\prime \cap {\widetilde {\mathcal {H}}}_\mu $
. Thus, it now suffices to show that the
${\textsf {A}}^\prime \cap {\widetilde {\mathcal {H}}}_\mu $
. Thus, it now suffices to show that the
![]() $\Gamma $
-von Neumann algebra
$\Gamma $
-von Neumann algebra
![]() $\textsf {B}$
admits a
$\textsf {B}$
admits a
![]() $\Gamma $
-invariant state.
$\Gamma $
-invariant state.
Let
![]() $\mathcal {E}_0\colon \Gamma \ltimes L^\infty (G/P)\to L^\infty (G/P)$
be the canonical conditional expectation, which is
$\mathcal {E}_0\colon \Gamma \ltimes L^\infty (G/P)\to L^\infty (G/P)$
be the canonical conditional expectation, which is
![]() $\Gamma $
-equivariant and faithful. Then, the composition
$\Gamma $
-equivariant and faithful. Then, the composition
![]() $\varrho (\cdot ) \colon = \int _{G/P} \mathcal {E}_0(\cdot )(x) \,d\nu _P(x)$
defines a faithful ultraweakly continuous
$\varrho (\cdot ) \colon = \int _{G/P} \mathcal {E}_0(\cdot )(x) \,d\nu _P(x)$
defines a faithful ultraweakly continuous
![]() $\mu $
-stationary state on
$\mu $
-stationary state on
![]() $\Gamma \ltimes L^\infty (G/P)$
. We will show that the restriction of
$\Gamma \ltimes L^\infty (G/P)$
. We will show that the restriction of
![]() $\varrho $
to
$\varrho $
to
![]() $\textsf {B}$
is
$\textsf {B}$
is
![]() $\Gamma $
-invariant.
$\Gamma $
-invariant.
First, we see that
![]() $\Gamma \!\curvearrowright \! \textsf {B}$
is ergodic, that is, the
$\Gamma \!\curvearrowright \! \textsf {B}$
is ergodic, that is, the
![]() $\Gamma $
-invariant subalgebra
$\Gamma $
-invariant subalgebra
![]() $\{\mathfrak {b}\in \textsf {B} : g\mathfrak {b}=\mathfrak {b} \text { for all } g\in \Gamma \}$
of
$\{\mathfrak {b}\in \textsf {B} : g\mathfrak {b}=\mathfrak {b} \text { for all } g\in \Gamma \}$
of
![]() $\textsf {B}$
is trivial. One can show this, for instance, by using the freeness of
$\textsf {B}$
is trivial. One can show this, for instance, by using the freeness of
![]() $\Gamma \!\curvearrowright \! G/P$
to conclude a unique stationarity as in [Reference Hartman and KalantarHK, Example 4.13], and then apply [Reference Bader, Boutonnet, Houdayer and PetersonBBHP20, Proposition 2.7(3)] (alternatively, [Reference Das and PetersonDP20, Theorem A] can be used to show the claim). However, it was pointed out to us by Rémi Boutonnet that the ergodicity in the above follows from the following general fact, which we include for the future easy reference; we thank him for providing us with the proof.
$\Gamma \!\curvearrowright \! G/P$
to conclude a unique stationarity as in [Reference Hartman and KalantarHK, Example 4.13], and then apply [Reference Bader, Boutonnet, Houdayer and PetersonBBHP20, Proposition 2.7(3)] (alternatively, [Reference Das and PetersonDP20, Theorem A] can be used to show the claim). However, it was pointed out to us by Rémi Boutonnet that the ergodicity in the above follows from the following general fact, which we include for the future easy reference; we thank him for providing us with the proof.
Lemma 2.16. (Boutonnet)
Let
![]() $\Gamma $
be an icc group with
$\Gamma $
be an icc group with
![]() $\mu \in \operatorname {Prob}(\Gamma )$
whose support generates
$\mu \in \operatorname {Prob}(\Gamma )$
whose support generates
![]() $\Gamma $
. If
$\Gamma $
. If
![]() $(X,\nu )$
is any ergodic
$(X,\nu )$
is any ergodic
![]() $(\Gamma , \mu )$
-space, then the action of
$(\Gamma , \mu )$
-space, then the action of
![]() $\Gamma $
on the crossed product
$\Gamma $
on the crossed product
![]() $\Gamma \ltimes L^\infty (X)$
by conjugation is ergodic.
$\Gamma \ltimes L^\infty (X)$
by conjugation is ergodic.
Proof. Denote by
![]() $\mathcal {E}: \Gamma \ltimes L^\infty (X)\to L^\infty (X)$
the canonical conditional expectation. Let
$\mathcal {E}: \Gamma \ltimes L^\infty (X)\to L^\infty (X)$
the canonical conditional expectation. Let
![]() $\mathfrak {a}\in \Gamma \ltimes L^\infty (X)$
be
$\mathfrak {a}\in \Gamma \ltimes L^\infty (X)$
be
![]() $\Gamma $
-fixed, and set
$\Gamma $
-fixed, and set
![]() $\mathfrak {b}=\mathfrak {a}- \mathcal {E}(\mathfrak {a})$
. Then,
$\mathfrak {b}=\mathfrak {a}- \mathcal {E}(\mathfrak {a})$
. Then,
![]() $\mathfrak {b}$
is
$\mathfrak {b}$
is
![]() $\Gamma $
-fixed and
$\Gamma $
-fixed and
![]() $\mathcal {E}(\mathfrak {b})=0$
. For
$\mathcal {E}(\mathfrak {b})=0$
. For
![]() $g \in \Gamma $
, let
$g \in \Gamma $
, let
![]() $\phi _{g} := \mathcal {E}(\mathfrak {b} \unicode{x3bb} _{g^{-1}}) \in L^{\infty }(X)$
be the Fourier coefficient of
$\phi _{g} := \mathcal {E}(\mathfrak {b} \unicode{x3bb} _{g^{-1}}) \in L^{\infty }(X)$
be the Fourier coefficient of
![]() $\mathfrak {b}$
at g. Since
$\mathfrak {b}$
at g. Since
![]() $\mathfrak {b}$
is
$\mathfrak {b}$
is
![]() $\Gamma $
-fixed, the map
$\Gamma $
-fixed, the map
![]() $g\mapsto |\phi _{g}|^{2}$
is
$g\mapsto |\phi _{g}|^{2}$
is
![]() $\Gamma $
-equivariant with respect to the conjugation action of
$\Gamma $
-equivariant with respect to the conjugation action of
![]() $\Gamma $
on itself, and so the function
$\Gamma $
on itself, and so the function
![]() $\zeta (g):= \int _{X} |\phi _{g}|^{2}\,d\nu $
is
$\zeta (g):= \int _{X} |\phi _{g}|^{2}\,d\nu $
is
![]() $\mu $
-harmonic (for the conjugate action). Moreover,
$\mu $
-harmonic (for the conjugate action). Moreover,
![]() $\sum _{g \in \Gamma } \zeta (g) = \int _{X} \mathcal {E}(\mathfrak {b}^{*} \mathfrak {b})\,d\nu <\infty $
, and so
$\sum _{g \in \Gamma } \zeta (g) = \int _{X} \mathcal {E}(\mathfrak {b}^{*} \mathfrak {b})\,d\nu <\infty $
, and so
![]() $\zeta \in \ell ^{1}(\Gamma )$
. Thus,
$\zeta \in \ell ^{1}(\Gamma )$
. Thus,
![]() $\zeta $
attains its maximum values on a finite conjugate-invariant subset of
$\zeta $
attains its maximum values on a finite conjugate-invariant subset of
![]() $\Gamma $
, that is,
$\Gamma $
, that is,
![]() $\{e\}$
by the icc assumption. Since
$\{e\}$
by the icc assumption. Since
![]() $\zeta \geq 0$
and
$\zeta \geq 0$
and
![]() $\zeta (e) = 0$
, it follows that
$\zeta (e) = 0$
, it follows that
![]() $\zeta = 0$
. So
$\zeta = 0$
. So
![]() $\mathfrak {b}=0$
and
$\mathfrak {b}=0$
and
![]() $\mathfrak {a}=\mathcal {E}(\mathfrak {a})$
. The function
$\mathfrak {a}=\mathcal {E}(\mathfrak {a})$
. The function
![]() $\mathcal {E}(\mathfrak {a})\in L^\infty (X)$
is
$\mathcal {E}(\mathfrak {a})\in L^\infty (X)$
is
![]() $\Gamma $
-invariant, and hence constant by ergodicity of
$\Gamma $
-invariant, and hence constant by ergodicity of
![]() $\Gamma \!\curvearrowright \! (X, \nu )$
.
$\Gamma \!\curvearrowright \! (X, \nu )$
.
Returning to the proof of the theorem, we can now invoke [Reference Boutonnet and HoudayerBH21, Theorem B] to conclude that either the restriction
![]() ${\varrho }|_{\textsf {B}}$
is
${\varrho }|_{\textsf {B}}$
is
![]() $\Gamma $
-invariant, or that there exists a closed subgroup
$\Gamma $
-invariant, or that there exists a closed subgroup
![]() $P\subset Q\subsetneq G$
and a
$P\subset Q\subsetneq G$
and a
![]() $\Gamma $
-equivariant von Neumann algebra embedding
$\Gamma $
-equivariant von Neumann algebra embedding
![]() ${\vartheta \colon L^{\infty }(G/Q,\nu _{Q}) \to \textsf {B}}$
, such that
${\vartheta \colon L^{\infty }(G/Q,\nu _{Q}) \to \textsf {B}}$
, such that
![]() $\varrho \circ \vartheta =\nu _{Q}$
, where
$\varrho \circ \vartheta =\nu _{Q}$
, where
![]() $\nu _Q$
is the pushforward of
$\nu _Q$
is the pushforward of
![]() $\nu _P$
under the canonical map
$\nu _P$
under the canonical map
![]() $G/P\to G/Q$
. We will rule out the latter possibility.
$G/P\to G/Q$
. We will rule out the latter possibility.
For the sake of contradiction, assume otherwise, that such
![]() $\vartheta $
exists. Then the composition
$\vartheta $
exists. Then the composition
![]() $\mathcal {E}_0\circ \vartheta $
is an ultraweakly continuous
$\mathcal {E}_0\circ \vartheta $
is an ultraweakly continuous
![]() $\Gamma $
-equivariant ucp map from
$\Gamma $
-equivariant ucp map from
![]() $L^{\infty }(G/Q,\nu _{Q})$
into
$L^{\infty }(G/Q,\nu _{Q})$
into
![]() $L^{\infty }(G/P,\nu _P)$
. The canonical embedding is the unique such map (see, e.g., [Reference Bader and ShalomBS06, Theorem 2.14]), so
$L^{\infty }(G/P,\nu _P)$
. The canonical embedding is the unique such map (see, e.g., [Reference Bader and ShalomBS06, Theorem 2.14]), so
![]() $\mathcal {E}_0\circ \vartheta =\operatorname {id}|_{L^{\infty }(G/Q)}$
. Since
$\mathcal {E}_0\circ \vartheta =\operatorname {id}|_{L^{\infty }(G/Q)}$
. Since
![]() $\mathcal {E}_0$
is a faithful conditional expectation, it follows that
$\mathcal {E}_0$
is a faithful conditional expectation, it follows that
![]() $\vartheta =\operatorname {id}|_{L^{\infty }(G/Q)}$
by [Reference HamanaHam85, Lemma 3.3]. In particular,
$\vartheta =\operatorname {id}|_{L^{\infty }(G/Q)}$
by [Reference HamanaHam85, Lemma 3.3]. In particular,
![]() $L^{\infty }(G/Q)\subset \textsf {B}\cap L^{\infty }(G/P)$
, and therefore,
$L^{\infty }(G/Q)\subset \textsf {B}\cap L^{\infty }(G/P)$
, and therefore,
![]() $\Phi (L^{\infty }(G/Q))\subset \textsf {A}^{\prime }\cap \mathcal {H}_\mu $
. As remarked above,
$\Phi (L^{\infty }(G/Q))\subset \textsf {A}^{\prime }\cap \mathcal {H}_\mu $
. As remarked above,
![]() $\Phi $
restricts to the Poisson transform on
$\Phi $
restricts to the Poisson transform on
![]() $L^\infty (G/P)$
, so
$L^\infty (G/P)$
, so
![]() $\textsf {A}\subset {(\mathcal {P}_{\nu _Q}(L^{\infty }(G/Q)))}^\prime $
.
$\textsf {A}\subset {(\mathcal {P}_{\nu _Q}(L^{\infty }(G/Q)))}^\prime $
.
Since
![]() $\textsf {A}$
is non-trivial and
$\textsf {A}$
is non-trivial and
![]() $\textsf {A}\cap \ell ^\infty (\Gamma )={\mathbb {C}}1$
, there exists
$\textsf {A}\cap \ell ^\infty (\Gamma )={\mathbb {C}}1$
, there exists
![]() $\mathfrak {a}\in \textsf {A}$
and
$\mathfrak {a}\in \textsf {A}$
and
![]() $g, h\in \Gamma $
with
$g, h\in \Gamma $
with
![]() $g\neq h$
such that
$g\neq h$
such that
![]() $\langle \mathfrak {a}\delta _{g},\delta _{h}\rangle \neq 0$
. Denoting by
$\langle \mathfrak {a}\delta _{g},\delta _{h}\rangle \neq 0$
. Denoting by
![]() $\mathfrak {m}_\phi \in B(\ell ^2(\Gamma ))$
the multiplication operator associated to a function
$\mathfrak {m}_\phi \in B(\ell ^2(\Gamma ))$
the multiplication operator associated to a function
![]() $\phi \in \ell ^\infty (\Gamma )$
,
$\phi \in \ell ^\infty (\Gamma )$
,
![]() $\mathfrak {a}$
commutes with
$\mathfrak {a}$
commutes with
![]() $\rho (g)\mathfrak {m}_{\mathcal {P}_{\nu _Q}(f)}\rho (g^{-1}) = \mathfrak {m}_{\mathcal {P}_{g\nu _Q}(f)}$
for every
$\rho (g)\mathfrak {m}_{\mathcal {P}_{\nu _Q}(f)}\rho (g^{-1}) = \mathfrak {m}_{\mathcal {P}_{g\nu _Q}(f)}$
for every
![]() $g\in \Gamma $
and
$g\in \Gamma $
and
![]() $f\in C(G/Q)$
. The action
$f\in C(G/Q)$
. The action
![]() $G\!\curvearrowright \! G/Q$
is strongly proximal, so given
$G\!\curvearrowright \! G/Q$
is strongly proximal, so given
![]() $x\in G/Q$
, there exits a net
$x\in G/Q$
, there exits a net
![]() $(g_i)$
in
$(g_i)$
in
![]() $\Gamma $
such that
$\Gamma $
such that
![]() $g_i\nu _Q\xrightarrow {\text {weak*}} \delta _x$
in
$g_i\nu _Q\xrightarrow {\text {weak*}} \delta _x$
in
![]() $\mathrm {Prob}(G/Q)$
. This implies
$\mathrm {Prob}(G/Q)$
. This implies
![]() $\mathfrak {m}_{\mathcal {P}_{g_i\nu _Q}(f)} \xrightarrow {\mathrm {WOT}} \mathfrak {m}_{\mathcal {P}_{\delta _x}(f)}$
in
$\mathfrak {m}_{\mathcal {P}_{g_i\nu _Q}(f)} \xrightarrow {\mathrm {WOT}} \mathfrak {m}_{\mathcal {P}_{\delta _x}(f)}$
in
![]() $B(\ell ^2(\Gamma ))$
, and therefore
$B(\ell ^2(\Gamma ))$
, and therefore
![]() $\mathfrak {a}$
commutes with
$\mathfrak {a}$
commutes with
![]() $\mathfrak {m}_{\mathcal {P}_{\delta _x}(f)}$
for every
$\mathfrak {m}_{\mathcal {P}_{\delta _x}(f)}$
for every
![]() $f\in C(G/Q)$
and
$f\in C(G/Q)$
and
![]() $x\in G/Q$
. Hence,
$x\in G/Q$
. Hence,
 $$ \begin{align*}\begin{split} f(g x) \langle \mathfrak{a} \delta_{g},\delta_{h}\rangle &=\langle \mathfrak{a} \mathfrak{m}_{\mathcal{P}_{\delta_x}(f)}\delta_{g},\delta_{h}\rangle =\langle \mathfrak{m}_{\mathcal{P}_{\delta_x}(f)}\mathfrak{a} \delta_{g},\delta_{h}\rangle \\& =\langle \mathfrak{a} \delta_{g},\mathfrak{m}_{\mathcal{P}_{\delta_x}(\bar{f})}\delta_{h}\rangle= f(h x)\langle \mathfrak{a} \delta_{g},\delta_{h}\rangle \end{split}\end{align*} $$
$$ \begin{align*}\begin{split} f(g x) \langle \mathfrak{a} \delta_{g},\delta_{h}\rangle &=\langle \mathfrak{a} \mathfrak{m}_{\mathcal{P}_{\delta_x}(f)}\delta_{g},\delta_{h}\rangle =\langle \mathfrak{m}_{\mathcal{P}_{\delta_x}(f)}\mathfrak{a} \delta_{g},\delta_{h}\rangle \\& =\langle \mathfrak{a} \delta_{g},\mathfrak{m}_{\mathcal{P}_{\delta_x}(\bar{f})}\delta_{h}\rangle= f(h x)\langle \mathfrak{a} \delta_{g},\delta_{h}\rangle \end{split}\end{align*} $$
for all
![]() $f\in C(G/Q)$
and
$f\in C(G/Q)$
and
![]() $x\in G/Q$
, which implies that
$x\in G/Q$
, which implies that
![]() $gh^{-1}$
lies in
$gh^{-1}$
lies in
![]() $\mathrm {ker}(\Gamma \!\curvearrowright \! G/Q)$
. However, it follows from similar reasoning as in the beginning of the proof that
$\mathrm {ker}(\Gamma \!\curvearrowright \! G/Q)$
. However, it follows from similar reasoning as in the beginning of the proof that
![]() ${\Gamma \!\curvearrowright \! G/Q}$
is faithful. This contradicts
${\Gamma \!\curvearrowright \! G/Q}$
is faithful. This contradicts
![]() $g\neq h$
, and therefore completes the proof of the first half.
$g\neq h$
, and therefore completes the proof of the first half.
Property (T) half. The assumptions imply that G has property (T) and since
![]() $\Gamma $
is a lattice in G,
$\Gamma $
is a lattice in G,
![]() $\Gamma $
also has property (T) (see, e.g., [Reference Bekka, de la Harpe and ValetteBdlHV08, Theorem 1.7.1]). Recall that
$\Gamma $
also has property (T) (see, e.g., [Reference Bekka, de la Harpe and ValetteBdlHV08, Theorem 1.7.1]). Recall that
![]() $\pi $
is a unitary representation of
$\pi $
is a unitary representation of
![]() $\Gamma $
weakly contained in
$\Gamma $
weakly contained in
![]() $\unicode{x3bb} $
.
$\unicode{x3bb} $
.
Let
![]() $M={\pi (\textsf {A})}^\prime $
, and let
$M={\pi (\textsf {A})}^\prime $
, and let
![]() $(M, H_{\textsf {s}}, \textsf {J}_{\textsf {s}}, \textsf {P}_{\textsf {s}})$
be its standard form in the sense of [Reference HaagerupHaa75]. By [Reference HaagerupHaa75, Theorem 3.2], the action
$(M, H_{\textsf {s}}, \textsf {J}_{\textsf {s}}, \textsf {P}_{\textsf {s}})$
be its standard form in the sense of [Reference HaagerupHaa75]. By [Reference HaagerupHaa75, Theorem 3.2], the action
![]() $\Gamma \!\curvearrowright \! M$
is implemented by a unitary representation
$\Gamma \!\curvearrowright \! M$
is implemented by a unitary representation
![]() $\sigma $
of
$\sigma $
of
![]() $\Gamma $
on
$\Gamma $
on
![]() $H_{\textsf {s}}$
. As usual, we endow
$H_{\textsf {s}}$
. As usual, we endow
![]() $B(H_{\textsf {s}})$
with the
$B(H_{\textsf {s}})$
with the
![]() $\Gamma $
-action defined by unitaries
$\Gamma $
-action defined by unitaries
![]() $\sigma _g$
,
$\sigma _g$
,
![]() $g\in \Gamma $
.
$g\in \Gamma $
.
By the amenability half, there exists a
![]() $\Gamma $
-invariant state on M. Since M has separable predual, a standard argument yields a sequence
$\Gamma $
-invariant state on M. Since M has separable predual, a standard argument yields a sequence
![]() $(\omega _n)$
of ultraweakly continuous states on M such that
$(\omega _n)$
of ultraweakly continuous states on M such that
![]() $\|\omega _n\circ \sigma _g - \omega _n\|=\|g\omega _n - \omega _n\|\xrightarrow {n\to \infty } 0$
for every
$\|\omega _n\circ \sigma _g - \omega _n\|=\|g\omega _n - \omega _n\|\xrightarrow {n\to \infty } 0$
for every
![]() $g\in \Gamma $
.
$g\in \Gamma $
.
By [Reference HaagerupHaa75, Lemma 2.10], the map
![]() $\xi \mapsto \omega _\xi |_M$
is a
$\xi \mapsto \omega _\xi |_M$
is a
![]() $\Gamma $
-equivariant homeomorphism from
$\Gamma $
-equivariant homeomorphism from
![]() $\textsf {P}_{\textsf {s}}$
onto
$\textsf {P}_{\textsf {s}}$
onto
![]() $M_*^+$
, where
$M_*^+$
, where
![]() $\omega _\xi $
is the vector state defined by the vector
$\omega _\xi $
is the vector state defined by the vector
![]() $\xi $
. Hence, there exists an almost
$\xi $
. Hence, there exists an almost
![]() $\sigma $
-invariant sequence in
$\sigma $
-invariant sequence in
![]() $H_{\textsf {s}}$
, and property (T) implies the existence of a
$H_{\textsf {s}}$
, and property (T) implies the existence of a
![]() $\sigma (\Gamma )$
-invariant unit vector
$\sigma (\Gamma )$
-invariant unit vector
![]() $\xi _0$
in
$\xi _0$
in
![]() $H_{\textsf {s}}$
. The vector state
$H_{\textsf {s}}$
. The vector state
![]() $\omega _{\xi _0}$
restricts to an ultraweakly continuous
$\omega _{\xi _0}$
restricts to an ultraweakly continuous
![]() $\Gamma $
-invariant state on M. This completes the proof.
$\Gamma $
-invariant state on M. This completes the proof.
We note that the proof of Theorem 1.5 also yields the following theorem.
Theorem 2.17. Every group
![]() $\Gamma $
as in the statement of Theorem 1.5 is
$\Gamma $
as in the statement of Theorem 1.5 is
![]() $\unicode{x3bb} _\Gamma $
-just-infinite.
$\unicode{x3bb} _\Gamma $
-just-infinite.
Remark 2.18. Margulis’ NST and its generalizations, such as Stuck–Zimmer’s theorem [Reference Stuck and ZimmerSZ94], are considered as rigidity results for
![]() $\Gamma $
. However, there are just-infinite groups that should not really be considered as rigid objects in the same spirit as higher rank lattices.
$\Gamma $
. However, there are just-infinite groups that should not really be considered as rigid objects in the same spirit as higher rank lattices.
Examples 2.5 and 2.15 show that the non-commutative just-infiniteness properties we consider in this section are indeed stronger properties than the usual group-theoretic notion, and therefore in this sense, Theorems 1.5 and 2.17 entail sharper results compared to the NST for lattices
![]() $\Gamma $
as in the statements.
$\Gamma $
as in the statements.
Remark 2.19. Another fact that follows from the arguments in the proof of Theorem 1.5 is that, given a discrete property (T) group
![]() $\Gamma $
and a unitary representations
$\Gamma $
and a unitary representations
![]() $\pi $
of
$\pi $
of
![]() $\Gamma $
, the weak
$\Gamma $
, the weak
![]() $\pi $
-just-infiniteness of
$\pi $
-just-infiniteness of
![]() $\Gamma $
depends only on the weak equivalence class of
$\Gamma $
depends only on the weak equivalence class of
![]() $\pi $
.
$\pi $
.
3 Proofs of Theorems 1.1, 1.2, and 1.3
Proof of Theorem 1.1
Let
![]() $\textsf {N}$
be a non-trivial
$\textsf {N}$
be a non-trivial
![]() $\Gamma $
-invariant von Neumann subalgebra of
$\Gamma $
-invariant von Neumann subalgebra of
![]() $\mathcal {L}(\Gamma )$
. By Corollary 2.9 and Theorem 2.17,
$\mathcal {L}(\Gamma )$
. By Corollary 2.9 and Theorem 2.17,
![]() $\textsf {N}$
is a factor. As shown in [Reference BruggerBru18, Lemma 4.3.2 and Remark 4.2.8], there is a non-trivial normal subgroup
$\textsf {N}$
is a factor. As shown in [Reference BruggerBru18, Lemma 4.3.2 and Remark 4.2.8], there is a non-trivial normal subgroup
![]() ${\Lambda }\le \Gamma $
such that the restriction of the
${\Lambda }\le \Gamma $
such that the restriction of the
![]() $\Gamma $
-action on
$\Gamma $
-action on
![]() $\textsf {N}$
to
$\textsf {N}$
to
![]() ${\Lambda }$
is inner. This gives a projective unitary representation
${\Lambda }$
is inner. This gives a projective unitary representation
![]() ${\sigma :{\Lambda }\to \mathcal {U}(\textsf {N})}$
. Since
${\sigma :{\Lambda }\to \mathcal {U}(\textsf {N})}$
. Since
![]() $\textsf {N}$
is a factor, we also get a projective unitary representation
$\textsf {N}$
is a factor, we also get a projective unitary representation
![]() $\sigma ^\prime :\Lambda \to \mathcal {U}(\textsf {N}^\prime \cap \mathcal {L}(\Gamma ))$
such that
$\sigma ^\prime :\Lambda \to \mathcal {U}(\textsf {N}^\prime \cap \mathcal {L}(\Gamma ))$
such that
![]() $\unicode{x3bb} _\Gamma (g) = \sigma (g)\sigma ^\prime (g)$
for all
$\unicode{x3bb} _\Gamma (g) = \sigma (g)\sigma ^\prime (g)$
for all
![]() $g\in \Lambda $
. Furthermore, the latter decomposition is unique. In particular, both
$g\in \Lambda $
. Furthermore, the latter decomposition is unique. In particular, both
![]() $\sigma (\Lambda )^{\prime \prime }$
and
$\sigma (\Lambda )^{\prime \prime }$
and
![]() $\sigma ^\prime (\Lambda )^{\prime \prime }$
are
$\sigma ^\prime (\Lambda )^{\prime \prime }$
are
![]() $\Gamma $
-invariant, hence factors by Corollary 2.9.
$\Gamma $
-invariant, hence factors by Corollary 2.9.
Let
![]() $\tilde {\textsf {N}}$
be the von Neumann algebra generated by
$\tilde {\textsf {N}}$
be the von Neumann algebra generated by
![]() $\sigma ({\Lambda })\cup \sigma ^\prime (\Lambda )$
. Then,
$\sigma ({\Lambda })\cup \sigma ^\prime (\Lambda )$
. Then,
![]() $\mathcal {L}(\Lambda )\subseteq \tilde {\textsf {N}}\subseteq \mathcal {L}(\Gamma )$
. Since
$\mathcal {L}(\Lambda )\subseteq \tilde {\textsf {N}}\subseteq \mathcal {L}(\Gamma )$
. Since
![]() $\Lambda $
has finite index in
$\Lambda $
has finite index in
![]() $\Gamma $
(by Margulis’ NST), it follows that
$\Gamma $
(by Margulis’ NST), it follows that
![]() $\mathcal {L}(\Lambda )$
has finite index in
$\mathcal {L}(\Lambda )$
has finite index in
![]() $\tilde {\textsf {N}}$
. Thus, as observed in the proof of [Reference BruggerBru18, Lemma 4.3.6], it cannot be the case that the trace vanishes on
$\tilde {\textsf {N}}$
. Thus, as observed in the proof of [Reference BruggerBru18, Lemma 4.3.6], it cannot be the case that the trace vanishes on
![]() $\sigma (g)$
and
$\sigma (g)$
and
![]() $\sigma ^\prime (g)$
for all non-neutral
$\sigma ^\prime (g)$
for all non-neutral
![]() $g\in {\Lambda }$
.
$g\in {\Lambda }$
.
The proof of [Reference Boutonnet and HoudayerBH21, Theorem C] can be modified to conclude that if
![]() $\pi $
is a projective unitary representation of
$\pi $
is a projective unitary representation of
![]() ${\Lambda }$
generating a finite factor
${\Lambda }$
generating a finite factor
![]() $\textsf {M}$
, then either
$\textsf {M}$
, then either
![]() $\textsf {M}$
is finite dimensional or else,
$\textsf {M}$
is finite dimensional or else,
![]() $\tau (\pi (g)) = 0$
for all
$\tau (\pi (g)) = 0$
for all
![]() $g\in {\Lambda }$
,
$g\in {\Lambda }$
,
![]() $g\neq e$
, where
$g\neq e$
, where
![]() $\tau $
is the trace of
$\tau $
is the trace of
![]() $\textsf {M}$
.
$\textsf {M}$
.
Thus, either
![]() $\sigma ({\Lambda })^{\prime \prime }$
or
$\sigma ({\Lambda })^{\prime \prime }$
or
![]() $\sigma ^\prime ({\Lambda })^{\prime \prime }$
is finite dimensional, and hence trivial by Proposition 2.7 since they are
$\sigma ^\prime ({\Lambda })^{\prime \prime }$
is finite dimensional, and hence trivial by Proposition 2.7 since they are
![]() $\Gamma $
-invariant. We therefore conclude that either
$\Gamma $
-invariant. We therefore conclude that either
![]() $\textsf {N}$
or its relative commutant
$\textsf {N}$
or its relative commutant
![]() $\textsf {N}^\prime \cap \mathcal {L}(\Gamma )$
contains
$\textsf {N}^\prime \cap \mathcal {L}(\Gamma )$
contains
![]() $\mathcal {L}({\Lambda })$
, and hence has finite index in
$\mathcal {L}({\Lambda })$
, and hence has finite index in
![]() $\mathcal {L}(\Gamma )$
. This implies that one of the these von Neumann algebras is finite dimensional [Reference JonesJon83, Corollary 2.2.3], and hence trivial since they are
$\mathcal {L}(\Gamma )$
. This implies that one of the these von Neumann algebras is finite dimensional [Reference JonesJon83, Corollary 2.2.3], and hence trivial since they are
![]() $\Gamma $
-invariant. Since we assumed
$\Gamma $
-invariant. Since we assumed
![]() $\textsf {N}$
is non-trivial, it follows that
$\textsf {N}$
is non-trivial, it follows that
![]() ${\textsf {N}^\prime \cap \mathcal {L}(\Gamma )}$
is trivial. We may now invoke [Reference Chifan and DasCD20, Theorem 3.15] to conclude the result.
${\textsf {N}^\prime \cap \mathcal {L}(\Gamma )}$
is trivial. We may now invoke [Reference Chifan and DasCD20, Theorem 3.15] to conclude the result.
Remark 3.1. We thank the anonymous referee for pointing out to us that the proof of Theorem 1.1 could be simplified by using the recent results of [Reference Bader, Boutonnet and HoudayerBBH21]. Indeed, since
![]() $\textsf {N} \subset \mathcal {L}(\Gamma )$
is a non-trivial
$\textsf {N} \subset \mathcal {L}(\Gamma )$
is a non-trivial
![]() $\Gamma $
-invariant subfactor, [Reference Bader, Boutonnet and HoudayerBBH21, Theorem 6.2] implies that the inclusion
$\Gamma $
-invariant subfactor, [Reference Bader, Boutonnet and HoudayerBBH21, Theorem 6.2] implies that the inclusion
![]() $\textsf {N} \frak {p} \subset \frak {p} \mathcal {L}(\Gamma ) \frak {p}$
has finite Jones index for some non-zero projection
$\textsf {N} \frak {p} \subset \frak {p} \mathcal {L}(\Gamma ) \frak {p}$
has finite Jones index for some non-zero projection
![]() $\frak {p} \in \textsf {N}^\prime \cap \mathcal {L}(\Gamma )$
. This further implies that
$\frak {p} \in \textsf {N}^\prime \cap \mathcal {L}(\Gamma )$
. This further implies that
![]() $\frak {p}(\textsf {N}^\prime \cap \mathcal {L}(\Gamma ))\frak {p}$
is finite dimensional [Reference JonesJon83, Corollary 2.2.3] and so is
$\frak {p}(\textsf {N}^\prime \cap \mathcal {L}(\Gamma ))\frak {p}$
is finite dimensional [Reference JonesJon83, Corollary 2.2.3] and so is
![]() $\textsf {N}^\prime \cap \mathcal {L}(\Gamma )$
. Then by Corollary 2.9 and Theorem 2.17, it follows that
$\textsf {N}^\prime \cap \mathcal {L}(\Gamma )$
. Then by Corollary 2.9 and Theorem 2.17, it follows that
![]() $\textsf {N}^\prime \cap \mathcal {L}(\Gamma )$
is trivial.
$\textsf {N}^\prime \cap \mathcal {L}(\Gamma )$
is trivial.
Proof of Theorem 1.3
Let
![]() $\mathcal {E}$
be an ultraweakly continuous
$\mathcal {E}$
be an ultraweakly continuous
![]() $\Gamma $
-equivariant conditional expectation on
$\Gamma $
-equivariant conditional expectation on
![]() $\mathcal {L}(\Gamma )$
. Then the image of
$\mathcal {L}(\Gamma )$
. Then the image of
![]() $\mathcal {E}$
is a
$\mathcal {E}$
is a
![]() $\Gamma $
-invariant von Neumann subalgebra of
$\Gamma $
-invariant von Neumann subalgebra of
![]() $\mathcal {L}(\Gamma )$
, and hence of the form
$\mathcal {L}(\Gamma )$
, and hence of the form
![]() $\mathcal {L}({\Lambda })$
for some normal subgroup
$\mathcal {L}({\Lambda })$
for some normal subgroup
![]() ${\Lambda }\le \Gamma $
, by Theorem 1.1. Since
${\Lambda }\le \Gamma $
, by Theorem 1.1. Since
![]() $\Gamma $
is icc, the canonical trace
$\Gamma $
is icc, the canonical trace
![]() $\tau $
is the unique trace on
$\tau $
is the unique trace on
![]() $\mathcal {L}(\Gamma )$
. Since the characteristic function
$\mathcal {L}(\Gamma )$
. Since the characteristic function
![]() $\mathbf {1}_{\Lambda }$
extends to the unique trace preserving conditional expectation from
$\mathbf {1}_{\Lambda }$
extends to the unique trace preserving conditional expectation from
![]() $\mathcal {L}(\Gamma )$
onto
$\mathcal {L}(\Gamma )$
onto
![]() $\mathcal {L}({\Lambda })$
, it suffices to show that
$\mathcal {L}({\Lambda })$
, it suffices to show that
![]() $\mathcal {E}$
is trace preserving. For this, we observe that since
$\mathcal {E}$
is trace preserving. For this, we observe that since
![]() $\mathcal {E}$
is
$\mathcal {E}$
is
![]() $\Gamma $
-equivariant,
$\Gamma $
-equivariant,
![]() $\tau \mathcal {E}$
is
$\tau \mathcal {E}$
is
![]() $\Gamma $
-invariant and ultraweakly continuous, and hence a trace on
$\Gamma $
-invariant and ultraweakly continuous, and hence a trace on
![]() $\mathcal {L}(\Gamma )$
. By the uniqueness of
$\mathcal {L}(\Gamma )$
. By the uniqueness of
![]() $\tau $
, we conclude that
$\tau $
, we conclude that
![]() $\tau \mathcal {E}=\tau $
, thereby completing the proof.
$\tau \mathcal {E}=\tau $
, thereby completing the proof.
Proof of Theorem 1.2
Let
![]() $\pi $
be a weakly mixing unitary representation of
$\pi $
be a weakly mixing unitary representation of
![]() $\Gamma $
. From [Reference Boutonnet and HoudayerBH21, Corollary D], it follows that
$\Gamma $
. From [Reference Boutonnet and HoudayerBH21, Corollary D], it follows that
![]() $\pi $
weakly contains
$\pi $
weakly contains
![]() $\unicode{x3bb} _\Gamma $
and
$\unicode{x3bb} _\Gamma $
and
![]() $C^*_\pi (\Gamma )$
has a unique trace
$C^*_\pi (\Gamma )$
has a unique trace
![]() $\tau $
, which is the lift of the canonical trace on
$\tau $
, which is the lift of the canonical trace on
![]() $C^{*}_{\mathrm { red}}(\Gamma )$
. Let
$C^{*}_{\mathrm { red}}(\Gamma )$
. Let
![]() ${I}$
be the kernel of the quotient map
${I}$
be the kernel of the quotient map
![]() $C^*_\pi (\Gamma )\to C^{*}_{\mathrm {red}}(\Gamma )$
. Since
$C^*_\pi (\Gamma )\to C^{*}_{\mathrm {red}}(\Gamma )$
. Since
![]() $\tau $
factors to a faithful trace on
$\tau $
factors to a faithful trace on
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
, it follows that
$C^{*}_{\mathrm {red}}(\Gamma )$
, it follows that
![]() ${I} = \{\mathfrak {a}\in C^*_\pi (\Gamma ) \colon \tau (\mathfrak {a}^{*} \mathfrak {a}) = 0\}$
.
${I} = \{\mathfrak {a}\in C^*_\pi (\Gamma ) \colon \tau (\mathfrak {a}^{*} \mathfrak {a}) = 0\}$
.
Assume
![]() $\mathcal {E}$
is a
$\mathcal {E}$
is a
![]() $\Gamma $
-equivariant conditional expectation from
$\Gamma $
-equivariant conditional expectation from
![]() $C_{\pi }^{*}(\Gamma )$
onto a
$C_{\pi }^{*}(\Gamma )$
onto a
![]() $C^*$
-subalgebra
$C^*$
-subalgebra
![]() $\textsf {A}\subseteq C_{\pi }^{*}(\Gamma )$
. Since
$\textsf {A}\subseteq C_{\pi }^{*}(\Gamma )$
. Since
![]() $\mathcal {E}$
is
$\mathcal {E}$
is
![]() $\Gamma $
-equivariant,
$\Gamma $
-equivariant,
![]() $\textsf {A}$
is
$\textsf {A}$
is
![]() $\Gamma $
-invariant.
$\Gamma $
-invariant.
Furthermore, the
![]() $\Gamma $
-equivariance of
$\Gamma $
-equivariance of
![]() $\mathcal {E}$
implies that
$\mathcal {E}$
implies that
![]() $\tau \mathcal {E}$
is
$\tau \mathcal {E}$
is
![]() $\Gamma $
-invariant, and hence a trace on
$\Gamma $
-invariant, and hence a trace on
![]() $C^*_\pi (\Gamma )$
. Since
$C^*_\pi (\Gamma )$
. Since
![]() $\tau $
is the unique trace on
$\tau $
is the unique trace on
![]() $C^*_\pi (\Gamma )$
, we conclude
$C^*_\pi (\Gamma )$
, we conclude
![]() $\tau \mathcal {E}=\tau $
.
$\tau \mathcal {E}=\tau $
.
Given
![]() $\mathfrak {a}\in {I}$
, we get
$\mathfrak {a}\in {I}$
, we get
![]() $\tau (\mathcal {E}(\mathfrak {a})^*\mathcal {E}(\mathfrak {a}))\le \tau (\mathcal {E}(\mathfrak {a}^{*}\mathfrak {a})) = \tau (\mathfrak {a}^{*}\mathfrak {a}) = 0$
, which implies
$\tau (\mathcal {E}(\mathfrak {a})^*\mathcal {E}(\mathfrak {a}))\le \tau (\mathcal {E}(\mathfrak {a}^{*}\mathfrak {a})) = \tau (\mathfrak {a}^{*}\mathfrak {a}) = 0$
, which implies
![]() ${\mathcal {E}({I})\subset {I}}$
. Thus,
${\mathcal {E}({I})\subset {I}}$
. Thus,
![]() $\mathcal {E}$
factors to a map on
$\mathcal {E}$
factors to a map on
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
, which is also a
$C^{*}_{\mathrm {red}}(\Gamma )$
, which is also a
![]() $\Gamma $
-equivariant conditional expectation, we still denote it by
$\Gamma $
-equivariant conditional expectation, we still denote it by
![]() $\mathcal {E}$
. Since
$\mathcal {E}$
. Since
![]() $\mathcal {E}$
is trace-preserving, it is faithful on
$\mathcal {E}$
is trace-preserving, it is faithful on
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
, and hence
$C^{*}_{\mathrm {red}}(\Gamma )$
, and hence
![]() $\mathcal {E}$
extends to an ultraweakly continuous trace-preserving conditional expectation
$\mathcal {E}$
extends to an ultraweakly continuous trace-preserving conditional expectation
![]() $\mathcal {E}:\mathcal {L}(\Gamma )\to \textsf {A}^{\prime \prime }$
(see [Reference PopaPop00, Lemma 4.5]). By Theorem 1.3, we have that
$\mathcal {E}:\mathcal {L}(\Gamma )\to \textsf {A}^{\prime \prime }$
(see [Reference PopaPop00, Lemma 4.5]). By Theorem 1.3, we have that
![]() $\mathcal {E}|_{\unicode{x3bb} _\Gamma (\Gamma )}= \mathbf {1}_{\unicode{x3bb} _\Gamma ({\Lambda })}$
for some normal subgroup
$\mathcal {E}|_{\unicode{x3bb} _\Gamma (\Gamma )}= \mathbf {1}_{\unicode{x3bb} _\Gamma ({\Lambda })}$
for some normal subgroup
![]() $\Lambda \leq \Gamma $
. This implies
$\Lambda \leq \Gamma $
. This implies
![]() $\mathcal {E}|_{\pi (\Gamma )}=\mathbf {1}_{\pi ({\Lambda })}$
.
$\mathcal {E}|_{\pi (\Gamma )}=\mathbf {1}_{\pi ({\Lambda })}$
.
We conclude this section with an example of an icc group
![]() $\Gamma $
for which
$\Gamma $
for which
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
contains a
$C^{*}_{\mathrm {red}}(\Gamma )$
contains a
![]() $\Gamma $
-invariant unital
$\Gamma $
-invariant unital
![]() $C^*$
-subalgebra that is not the image of any
$C^*$
-subalgebra that is not the image of any
![]() $\Gamma $
-equivariant conditional expectation.
$\Gamma $
-equivariant conditional expectation.
Example 3.2. Let
![]() $\Gamma $
be as in Example 2.15, and let
$\Gamma $
be as in Example 2.15, and let
![]() $\textsf {A}$
be the
$\textsf {A}$
be the
![]() $C^*$
-subalgebra of
$C^*$
-subalgebra of
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
constructed from a non-zero proper ideal I as in the proof of Proposition 2.14. Assume
$C^{*}_{\mathrm {red}}(\Gamma )$
constructed from a non-zero proper ideal I as in the proof of Proposition 2.14. Assume
![]() $\mathcal {E}:C^{*}_{\mathrm {red}}(\Gamma )\to \textsf {A}$
is a
$\mathcal {E}:C^{*}_{\mathrm {red}}(\Gamma )\to \textsf {A}$
is a
![]() $\Gamma $
-equivariant conditional expectation. Since
$\Gamma $
-equivariant conditional expectation. Since
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
has a unique trace, it follows, as seen in the proof of Theorem 1.5 above, that
$C^{*}_{\mathrm {red}}(\Gamma )$
has a unique trace, it follows, as seen in the proof of Theorem 1.5 above, that
![]() $\mathcal {E}$
is faithful and trace preserving, and hence extends to the canonical trace preserving conditional expectation from
$\mathcal {E}$
is faithful and trace preserving, and hence extends to the canonical trace preserving conditional expectation from
![]() $\mathcal {L}(\Gamma )$
onto
$\mathcal {L}(\Gamma )$
onto
![]() $\textsf {A}^{\prime \prime }$
. Since
$\textsf {A}^{\prime \prime }$
. Since
![]() $\mathcal {L}(\Gamma )$
is a factor, and I is non-zero, it follows that
$\mathcal {L}(\Gamma )$
is a factor, and I is non-zero, it follows that
![]() $\textsf {A}^{\prime \prime }=\mathcal {L}(\Gamma )$
. In particular,
$\textsf {A}^{\prime \prime }=\mathcal {L}(\Gamma )$
. In particular,
![]() $\mathcal {E}=\operatorname {id}$
, and therefore,
$\mathcal {E}=\operatorname {id}$
, and therefore,
![]() $\textsf {A}=C^{*}_{\mathrm {red}}(\Gamma )$
. This implies the quotient of
$\textsf {A}=C^{*}_{\mathrm {red}}(\Gamma )$
. This implies the quotient of
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
by I has dimension one, and so admits a trace, which contradicts the uniqueness of the canonical trace on
$C^{*}_{\mathrm {red}}(\Gamma )$
by I has dimension one, and so admits a trace, which contradicts the uniqueness of the canonical trace on
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
. Hence,
$C^{*}_{\mathrm {red}}(\Gamma )$
. Hence,
![]() $\textsf {A}$
cannot be the image of any
$\textsf {A}$
cannot be the image of any
![]() $\Gamma $
-equivariant conditional expectation on
$\Gamma $
-equivariant conditional expectation on
![]() $C^{*}_{\mathrm {red}}(\Gamma )$
.
$C^{*}_{\mathrm {red}}(\Gamma )$
.
Acknowledgements
We thank Rémi Boutonnet and Eduardo Scarparo for their valuable comments on the earlier versions of this paper. We also thank the anonymous referee for the careful reading of the paper and their insightful comments. M.K. is supported by a Simons Foundation Collaboration Grant (No. 713667).